Is The Square Root Of 12 Rational Or Irrational
Juapaving
Mar 31, 2025 · 5 min read
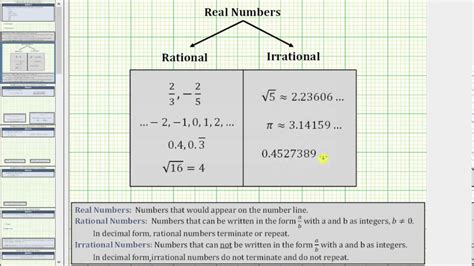
Table of Contents
Is the Square Root of 12 Rational or Irrational? A Deep Dive into Number Theory
The question of whether the square root of 12 is rational or irrational is a fundamental concept in number theory. Understanding this requires grasping the definitions of rational and irrational numbers and employing some basic proof techniques. This article will not only answer the question definitively but also delve deeper into the underlying mathematical principles, providing a comprehensive understanding of the topic.
Understanding Rational and Irrational Numbers
Before we tackle the square root of 12, let's define our terms:
Rational Numbers: A rational number is any number that can be expressed as a fraction p/q, where p and q are integers, and q is not equal to zero. Examples include 1/2, 3, -4/7, and 0. Essentially, rational numbers are numbers that can be represented by a ratio of two whole numbers. Their decimal representations either terminate (e.g., 1/4 = 0.25) or repeat (e.g., 1/3 = 0.333...).
Irrational Numbers: An irrational number is any number that cannot be expressed as a fraction of two integers. Their decimal representations are non-terminating and non-repeating. Famous examples include π (pi) and e (Euler's number). The square root of many numbers also falls into this category.
Determining the Nature of √12
To determine whether √12 is rational or irrational, we can employ a proof by contradiction. This method assumes the opposite of what we want to prove and then demonstrates that this assumption leads to a contradiction, thus proving the original statement.
Hypothesis: Let's assume that √12 is rational. This means it can be expressed as a fraction p/q, where p and q are integers, q ≠ 0, and p/q is in its simplest form (meaning p and q share no common factors other than 1).
Developing the Proof:
-
Express √12 as a fraction: If √12 is rational, then √12 = p/q, where p and q are integers with no common factors.
-
Square both sides: Squaring both sides of the equation, we get: 12 = p²/q²
-
Rearrange the equation: Multiplying both sides by q², we obtain: 12q² = p²
-
Deduction about p: This equation tells us that p² is divisible by 12. Since 12 = 2² * 3, it follows that p² is divisible by 2² and 3. This implies that p itself must be divisible by 2 and 3 (because if a perfect square is divisible by a prime number, the original number must also be divisible by that prime number). Therefore, we can say p = 6k, where k is an integer.
-
Substitute and simplify: Substituting p = 6k into the equation 12q² = p², we get: 12q² = (6k)² which simplifies to 12q² = 36k².
-
Further simplification: Dividing both sides by 12, we get: q² = 3k²
-
Deduction about q: This shows that q² is divisible by 3, and therefore q must also be divisible by 3.
-
Contradiction: We've now shown that both p and q are divisible by 3. This contradicts our initial assumption that p/q is in its simplest form (i.e., they share no common factors). This contradiction arises from our initial assumption that √12 is rational.
Conclusion: Since our assumption that √12 is rational leads to a contradiction, our initial assumption must be false. Therefore, √12 is irrational.
Simplifying √12 and Understanding its Irrationality
While we've proven √12 is irrational, it's helpful to simplify the expression to understand its nature better. We can simplify √12 as follows:
√12 = √(4 * 3) = √4 * √3 = 2√3
This shows that √12 is equivalent to 2 multiplied by the square root of 3. Since √3 is also irrational (a proof similar to the one above can be used), multiplying it by 2 doesn't change its irrational nature. The product of a rational number (2) and an irrational number (√3) remains irrational.
Exploring Other Square Roots: Rational and Irrational
Let's examine a few more examples to solidify our understanding:
- √16: This simplifies to 4, which is a rational number (4/1). Perfect squares always have rational square roots.
- √25: This simplifies to 5, another rational number.
- √2: This is a classic example of an irrational number. Its decimal representation is non-terminating and non-repeating (approximately 1.41421356...).
- √9/4: This simplifies to 3/2, a rational number. This highlights that the square roots of rational numbers aren't always irrational.
Practical Applications and Importance
The distinction between rational and irrational numbers isn't just a theoretical exercise; it has practical applications in various fields:
- Geometry: Calculating the diagonal of a square (using the Pythagorean theorem) often results in irrational numbers.
- Physics: Many physical constants, such as the speed of light, are expressed using irrational numbers.
- Computer Science: Representing irrational numbers in computers requires approximations, leading to potential errors in calculations.
Understanding the nature of numbers is crucial for accurate and reliable results in these fields.
Further Exploration: Proofs of Irrationality
The proof by contradiction is a powerful tool for demonstrating the irrationality of numbers. Many other proofs exist for different irrational numbers. For instance, similar techniques can be used to prove the irrationality of √2, √5, and √7. Exploring these proofs can further enhance your understanding of number theory. The key idea lies in identifying prime factors and demonstrating that an assumption of rationality leads to a contradiction based on the properties of integers and prime factorization.
Conclusion: The Irrationality of √12 and Beyond
The square root of 12 is indeed irrational. This fact stems from the fundamental properties of rational and irrational numbers, and its proof demonstrates the elegant power of mathematical reasoning. Understanding the nature of √12, and irrational numbers in general, provides a deeper appreciation for the rich tapestry of the number system and its implications in various scientific and mathematical disciplines. By exploring the concepts presented here and delving further into number theory, you can build a strong foundation for understanding more advanced mathematical concepts.
Latest Posts
Latest Posts
-
What Is The Symbol Of Acceleration
Apr 01, 2025
-
What Is The Lcm Of 3 And 5
Apr 01, 2025
-
Is Coal And Charcoal The Same
Apr 01, 2025
-
What Are The Common Multiples Of 3 And 5
Apr 01, 2025
-
Lcm Of 2 And 3 And 6
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Is The Square Root Of 12 Rational Or Irrational . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
