What Are The Common Multiples Of 3 And 5
Juapaving
Apr 01, 2025 · 5 min read
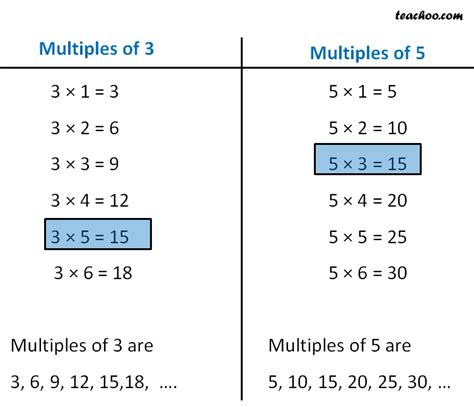
Table of Contents
What Are the Common Multiples of 3 and 5? A Deep Dive into Number Theory
Finding common multiples, especially for seemingly simple numbers like 3 and 5, might seem straightforward at first glance. However, a deeper exploration reveals fascinating connections to number theory, algorithmic thinking, and even practical applications in various fields. This article will delve into the concept of common multiples, focusing specifically on 3 and 5, explaining how to find them, their properties, and their broader significance in mathematics.
Understanding Multiples and Common Multiples
Before we dive into the specifics of 3 and 5, let's establish a firm understanding of fundamental concepts.
Multiples: A multiple of a number is the result of multiplying that number by any integer (whole number). For example, the multiples of 3 are 3, 6, 9, 12, 15, 18, and so on. Similarly, the multiples of 5 are 5, 10, 15, 20, 25, 30, and so on. Notice that the multiples extend infinitely in both positive and negative directions. However, we'll primarily focus on positive multiples in this discussion.
Common Multiples: A common multiple of two or more numbers is a number that is a multiple of all the given numbers. In simpler terms, it's a number that appears in the list of multiples for each number. For example, 15 is a common multiple of 3 and 5 because it appears in both lists (3 x 5 = 15 and 5 x 3 = 15). Other common multiples of 3 and 5 include 30, 45, 60, and so on.
Finding the Common Multiples of 3 and 5
There are several ways to identify the common multiples of 3 and 5:
1. Listing Multiples: A Simple Approach
The most straightforward method is to list the multiples of each number and identify the common ones.
Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48, 51, 54, 57, 60...
Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60...
By comparing the two lists, we can easily spot the common multiples: 15, 30, 45, 60, and so on. This method works well for smaller numbers but becomes less efficient as the numbers get larger.
2. Prime Factorization: A More Powerful Technique
Prime factorization offers a more systematic and efficient approach, especially for larger numbers. Let's break down 3 and 5 into their prime factors:
- 3 = 3 (3 is a prime number)
- 5 = 5 (5 is a prime number)
To find the least common multiple (LCM), we consider the highest power of each prime factor present in the numbers:
- LCM(3, 5) = 3 x 5 = 15
The LCM is the smallest positive common multiple. All other common multiples are multiples of the LCM. Therefore, the common multiples of 3 and 5 are 15, 30, 45, 60, 75, and so on (multiples of 15).
3. Using the Formula: A Concise Method
The relationship between the LCM and the product of two numbers is given by the formula:
LCM(a, b) * GCD(a, b) = a * b
Where:
- LCM(a, b) is the least common multiple of a and b
- GCD(a, b) is the greatest common divisor (highest common factor) of a and b
Since 3 and 5 are coprime (their greatest common divisor is 1), the LCM is simply their product:
LCM(3, 5) = 3 * 5 = 15
This confirms our previous findings. All common multiples are multiples of 15.
Least Common Multiple (LCM) and its Significance
The least common multiple (LCM) plays a crucial role in various mathematical problems and applications:
-
Fraction Addition and Subtraction: Finding the LCM of denominators is essential when adding or subtracting fractions. It ensures that you're working with a common denominator, allowing for simplified calculations.
-
Scheduling Problems: The LCM is often used to solve scheduling problems. For instance, if two events occur at intervals of 3 and 5 days respectively, the LCM (15) indicates when both events will occur simultaneously.
-
Cyclic Patterns: LCM helps identify the points where repeating cycles coincide. This is valuable in various applications, from understanding astronomical cycles to analyzing periodic phenomena in physics and engineering.
The Infinite Nature of Common Multiples
It's crucial to remember that the set of common multiples of any two numbers is infinite. We've identified the smallest common multiple (15) and showed how to generate others. However, there's no largest common multiple. The sequence continues indefinitely: 15, 30, 45, 60, 75, 90, 105, 120, and so on.
Beyond 3 and 5: Extending the Concepts
The principles discussed for finding common multiples of 3 and 5 are readily applicable to any pair (or set) of integers. The same methods – listing multiples, prime factorization, or using the LCM formula – can be employed. The complexity might increase slightly with larger numbers, but the underlying concepts remain the same.
Practical Applications: Real-world Examples
The concept of common multiples extends beyond abstract mathematical exercises. Consider these examples:
-
Concert Scheduling: Imagine two bands, one performing every 3 days and another every 5 days. Using the LCM, you can determine when both bands will perform on the same day, helping to optimize concert scheduling.
-
Production Line Synchronization: In manufacturing, different parts of a production line might operate at varying speeds. The LCM can help synchronize these processes, maximizing efficiency and minimizing downtime.
-
Traffic Light Synchronization: In urban planning, optimizing traffic light timings to minimize congestion often involves understanding common multiples. By syncing lights based on LCM calculations, smoother traffic flow can be achieved.
Conclusion: Understanding the Power of Common Multiples
The seemingly simple task of finding common multiples of 3 and 5 unveils a deeper appreciation for number theory and its applications. Understanding multiples, least common multiples, and the various methods for determining them equips you with valuable mathematical tools. These tools are not just confined to theoretical calculations; they have practical relevance in diverse fields, from scheduling and logistics to manufacturing and urban planning. Mastering the concept of common multiples opens doors to a broader understanding of mathematical relationships and their real-world impact. The journey from simple multiples to solving complex problems is a testament to the interconnectedness of seemingly disparate concepts within mathematics.
Latest Posts
Latest Posts
-
What Are Rows Called In The Periodic Table
Apr 02, 2025
-
120 Mins Is How Many Hours
Apr 02, 2025
-
How Many Miles Are In 10 Kilometers
Apr 02, 2025
-
Where In A Plant Cell Does Photosynthesis Occur
Apr 02, 2025
-
A Motor And A Generator Are
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Are The Common Multiples Of 3 And 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
