What Is The Refractive Index Of A Vacuum
Juapaving
Apr 01, 2025 · 5 min read
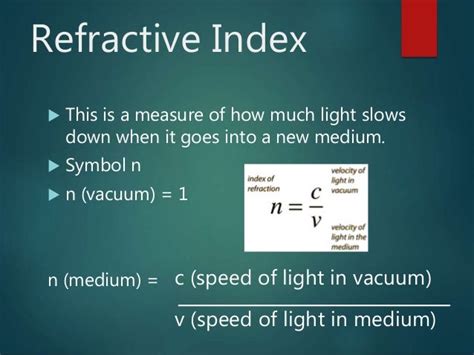
Table of Contents
What is the Refractive Index of a Vacuum?
The refractive index, a fundamental concept in optics, quantifies how fast light propagates through a medium relative to its speed in a vacuum. Understanding the refractive index of a vacuum is crucial for grasping the very foundation of optics and how light interacts with matter. This comprehensive article will delve deep into this topic, exploring its definition, significance, and implications across various fields of physics and engineering.
Defining Refractive Index
The refractive index (n) of a medium is defined as the ratio of the speed of light in a vacuum (c) to the speed of light in that medium (v):
n = c/v
A higher refractive index indicates that light travels slower in that medium compared to a vacuum. For instance, the refractive index of water is approximately 1.33, meaning light travels about 1.33 times slower in water than in a vacuum. This change in speed is what causes light to bend (refract) when it passes from one medium to another.
The Refractive Index of a Vacuum: A Special Case
The vacuum, by definition, is devoid of matter. Therefore, light travels through a vacuum unimpeded at its maximum speed, denoted by 'c'. Consequently, applying the refractive index formula:
n<sub>vacuum</sub> = c/c = 1
The refractive index of a vacuum is precisely 1. This is not merely a numerical value; it signifies the fundamental reference point for all refractive index measurements. All other refractive indices are relative to this value. Any material with a refractive index greater than 1 implies that light travels slower in that material than in a vacuum.
Significance of the Refractive Index of a Vacuum
The refractive index of a vacuum's value of 1 holds immense significance for several reasons:
-
Fundamental Constant: The speed of light in a vacuum (c) is a fundamental physical constant, approximately 299,792,458 meters per second. This constant, combined with the definition of refractive index, makes the refractive index of a vacuum a fundamental constant in physics.
-
Reference Point for Measurements: As mentioned earlier, the refractive index of a vacuum serves as the essential benchmark for measuring the refractive indices of all other materials. Without this reference point, comparing the optical properties of different materials would be impossible.
-
Basis for Optical Calculations: Many optical calculations, such as Snell's Law (governing refraction at interfaces), rely on the refractive index. The refractive index of a vacuum provides the base value essential for these calculations.
-
Understanding Light-Matter Interactions: The difference between the refractive index of a material and that of a vacuum provides insights into how light interacts with the atoms and molecules within that material. This interaction is influenced by factors like the material's electron density and its atomic structure.
Applications in Various Fields
The understanding of the refractive index, particularly in relation to the vacuum's value of 1, has profound implications across various fields:
1. Optics and Photonics
-
Lens Design: The design of lenses and other optical components relies heavily on precise knowledge of refractive indices. The difference in refractive indices between different materials allows for the manipulation of light, enabling the creation of focusing, diverging, and other optical elements.
-
Optical Fiber Communication: Optical fibers transmit light signals over long distances with minimal loss. The core and cladding of these fibers have different refractive indices, enabling total internal reflection, which guides the light along the fiber.
-
Spectroscopy: Spectroscopy utilizes the interaction of light with matter to analyze the composition and properties of substances. The refractive index plays a vital role in understanding how light interacts with the sample, providing insights into its molecular structure and properties.
2. Astronomy and Astrophysics
-
Redshift and Blueshift: The observed change in the wavelength of light from distant celestial objects (redshift or blueshift) is partially influenced by the refractive index of the interstellar medium. Accurate calculations require considering the refractive index variations in the vast expanse of space.
-
Gravitational Lensing: Massive objects like galaxies can bend the path of light, a phenomenon known as gravitational lensing. This effect relies on the interaction of light with the spacetime curvature, which, in turn, is linked to the refractive index variations within the gravitational field.
3. Material Science and Engineering
-
Material Characterization: The refractive index is a crucial parameter for characterizing materials. It provides information about the material's density, electronic structure, and its ability to interact with light.
-
Thin Film Technology: Thin films are used extensively in various applications, including optical coatings and semiconductor devices. Precise control over the refractive index of these films is essential for achieving desired optical properties.
4. Meteorology and Remote Sensing
-
Atmospheric Refraction: The Earth's atmosphere exhibits a varying refractive index due to changes in temperature, pressure, and humidity. This atmospheric refraction affects the propagation of light, particularly impacting astronomical observations and remote sensing applications.
-
Refractive Index Sensors: Sensors based on refractive index measurements are utilized for monitoring environmental parameters like temperature, pressure, and the concentration of various gases in the atmosphere.
Beyond the Ideal Vacuum
While the refractive index of a vacuum is theoretically 1, the concept of a "perfect" vacuum is an idealization. In reality, even the best vacuum achievable still contains a minute amount of residual gas molecules. These molecules, however sparse, can slightly affect the speed of light, leading to a refractive index infinitesimally different from 1. However, this deviation is so minuscule that it is generally negligible in most practical applications. The difference is so small that it is typically ignored in calculations unless dealing with extremely precise measurements or specialized scenarios.
Conclusion
The refractive index of a vacuum, exactly 1, serves as a cornerstone of optics and many related scientific disciplines. It represents the fundamental speed limit for light propagation, providing a crucial reference point for understanding light's interaction with matter. This value is essential for numerous applications across physics, engineering, astronomy, and beyond. While the perfect vacuum is an idealization, the deviation from the ideal value of 1 in real-world scenarios is typically inconsequential for most practical purposes. A thorough understanding of the refractive index of a vacuum is, therefore, crucial for researchers and practitioners working with light and its interactions with various media. Its significance extends far beyond the theoretical realm, influencing the design and function of countless technologies we use daily. The continued exploration and refinement of this fundamental constant promise further advancements in diverse fields, showcasing the enduring importance of a seemingly simple number: 1.
Latest Posts
Latest Posts
-
5 Letter Words Starting With Thu
Apr 02, 2025
-
Find The Ratio Of Their Perimeters
Apr 02, 2025
-
What Does The Fine Adjustment Knob Do On A Microscope
Apr 02, 2025
-
How Many Valence Electrons In Helium
Apr 02, 2025
-
An Equilateral Triangle Has Three Equal Angles
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Refractive Index Of A Vacuum . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
