An Equilateral Triangle Has Three Equal Angles
Juapaving
Apr 02, 2025 · 5 min read
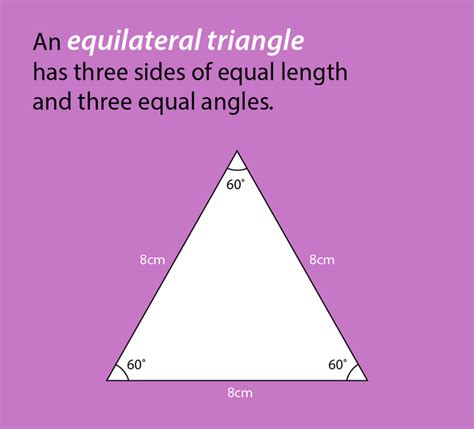
Table of Contents
An Equilateral Triangle Has Three Equal Angles: A Deep Dive into Geometry
An equilateral triangle, a fundamental shape in geometry, possesses a unique and defining characteristic: all three of its angles are equal. This seemingly simple statement underpins a wealth of fascinating properties and applications, extending far beyond the basics of Euclidean geometry. This article will delve into the intricacies of equilateral triangles, exploring their defining properties, derivations, applications, and connections to other mathematical concepts.
Understanding the Definition
Before we delve into the specifics, let's solidify our understanding of the definition: An equilateral triangle is a polygon with three sides of equal length. This equality of sides directly implies the equality of angles. This isn't just an observation; it's a theorem demonstrable through various geometric proofs. The fact that all three angles are equal is a direct consequence of the equal side lengths. This crucial relationship forms the bedrock of much of the subsequent analysis.
The Significance of Equal Angles
The equality of angles in an equilateral triangle is not merely a coincidence; it's a consequence of the fundamental axioms and theorems of geometry. This equality has profound implications:
- Symmetry: Equilateral triangles exhibit perfect rotational symmetry. They can be rotated by 120 degrees (or 240 degrees) about their center and still appear identical. This inherent symmetry makes them aesthetically pleasing and useful in various design applications.
- Stability: The equal angles contribute to the inherent structural stability of an equilateral triangle. This is why equilateral triangles are frequently used in engineering and construction, forming the basis for strong and rigid frameworks.
- Mathematical Elegance: The equilateral triangle's properties lend themselves to elegant mathematical proofs and applications, particularly in fields like trigonometry and calculus. Its simplicity belies its profound mathematical significance.
Proving the Equality of Angles
Several methods can be used to prove that the angles of an equilateral triangle are equal. Let's explore two common approaches:
1. Using the Isosceles Triangle Theorem
An isosceles triangle has at least two sides of equal length. The Isosceles Triangle Theorem states that the angles opposite these equal sides are also equal. Since an equilateral triangle has three equal sides, we can consider it as three isosceles triangles (each formed by choosing two of the equal sides).
- Consider side AB = AC. By the Isosceles Triangle Theorem, angle ABC = angle ACB.
- Consider side AC = BC. By the Isosceles Triangle Theorem, angle BAC = angle ABC.
- Therefore, angle ABC = angle ACB = angle BAC. All three angles are equal.
This proof elegantly demonstrates the connection between equal sides and equal angles, leveraging a well-established theorem in geometry.
2. Using the Interior Angles Sum Theorem
The Interior Angles Sum Theorem states that the sum of the interior angles of any triangle is always 180 degrees. Let's denote the angles of an equilateral triangle as A, B, and C. Since all sides are equal, all angles must also be equal.
- Let A = B = C = x. We've assigned the same variable to represent the equal angles.
- Apply the Interior Angles Sum Theorem: A + B + C = 180 degrees.
- Substitute: x + x + x = 180 degrees.
- Solve for x: 3x = 180 degrees; x = 60 degrees.
This proves that each angle in an equilateral triangle measures 60 degrees.
Applications of Equilateral Triangles
The unique properties of equilateral triangles make them invaluable in various fields:
1. Architecture and Engineering
Equilateral triangles offer exceptional structural strength and stability due to their symmetrical nature. They are frequently incorporated into:
- Trusses: These supporting structures in bridges and buildings often utilize equilateral triangles to distribute weight efficiently and withstand significant forces.
- Roof Structures: The strong and stable geometry of equilateral triangles makes them ideal for roof designs, ensuring structural integrity.
- Geodesic Domes: These structures, often resembling large spheres, use a network of interconnected equilateral triangles to create lightweight yet incredibly strong enclosures.
2. Design and Art
The aesthetic appeal of equilateral triangles is undeniable. Their symmetry and balanced proportions make them a popular element in:
- Logos and Branding: Many companies incorporate equilateral triangles into their logos to convey stability, balance, and precision.
- Tessellations: Equilateral triangles can perfectly tessellate (tile) a plane, creating visually appealing patterns frequently seen in art and architecture.
- Islamic Art: Equilateral triangles are often used in intricate geometric patterns found in Islamic art and architecture.
3. Mathematics and Physics
Equilateral triangles play a significant role in several mathematical and physical concepts:
- Trigonometry: They serve as fundamental examples in understanding trigonometric ratios and functions.
- Fractal Geometry: Equilateral triangles form the basis for the construction of various fractals, such as the Sierpinski Triangle.
- Crystallography: The symmetrical structure of equilateral triangles is observed in the arrangements of atoms in certain crystals.
Beyond the Basics: Exploring Related Concepts
The study of equilateral triangles extends beyond their basic properties. Here are some related concepts worth exploring:
1. The Circumcenter and Incenter
The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect. In an equilateral triangle, the circumcenter also coincides with the centroid (the intersection of the medians) and the orthocenter (the intersection of the altitudes). The incenter, the intersection of the angle bisectors, also coincides with these points. This concurrence of these points is a unique feature of equilateral triangles.
2. Area Calculation
The area of an equilateral triangle with side length 'a' can be calculated using the formula: Area = (√3/4) * a². This formula highlights the relationship between the side length and the area, demonstrating how a simple change in side length significantly affects the overall area.
3. Relationship to Other Shapes
Equilateral triangles are closely related to other geometric shapes, such as hexagons (composed of six equilateral triangles) and various regular polygons. Understanding these relationships broadens one's geometrical understanding and reveals underlying connections between seemingly disparate shapes.
Conclusion
The statement "an equilateral triangle has three equal angles" is much more than a simple geometric fact. It's a foundational principle that underpins a wealth of mathematical properties, practical applications, and aesthetic appeal. From its use in structural engineering to its appearance in artistic designs and its significance in mathematical proofs, the equilateral triangle's influence is far-reaching and continues to fascinate mathematicians, engineers, artists, and anyone captivated by the elegance of geometry. Its simplicity belies its profound importance in various fields, showcasing the power of fundamental geometric principles to unlock deeper understanding and diverse applications. Further exploration of its properties and related concepts will undoubtedly lead to even greater appreciation of this remarkable shape.
Latest Posts
Latest Posts
-
Is A Square Also A Parallelogram
Apr 03, 2025
-
1 Out Of 10 As A Percentage
Apr 03, 2025
-
How Are Prokaryotes And Eukaryotes Alike
Apr 03, 2025
-
Which Of The Following Statements Is Always True
Apr 03, 2025
-
Balanced Chemical Equation For Magnesium Oxide
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about An Equilateral Triangle Has Three Equal Angles . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
