What Is The Factor Of 94
Juapaving
Apr 07, 2025 · 5 min read
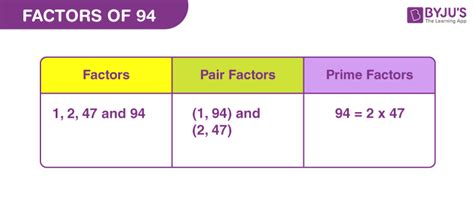
Table of Contents
What are the Factors of 94? A Deep Dive into Prime Factorization and Divisibility
Finding the factors of a number might seem like a simple arithmetic task, but understanding the process reveals fundamental concepts in number theory. This article will explore the factors of 94, explaining the method used to find them and delving into related mathematical ideas. We'll also touch upon the broader implications of factorization in various mathematical fields.
Understanding Factors and Divisibility
Before we tackle the specific case of 94, let's establish a clear definition. A factor (or divisor) of a number is any integer that divides the number without leaving a remainder. In simpler terms, if you can divide a number by another number and get a whole number as the result, then the number you divided by is a factor. This leads to the concept of divisibility: a number is divisible by another if the result of their division is a whole number (an integer).
Finding the Factors of 94: A Step-by-Step Approach
To find the factors of 94, we need to systematically check which numbers divide 94 without leaving a remainder. We can start by checking the smallest numbers:
- 1: Every number is divisible by 1. Therefore, 1 is a factor of 94.
- 2: Since 94 is an even number (ending in 4), it's divisible by 2. 94 divided by 2 is 47. So, 2 is a factor.
- 3: The divisibility rule for 3 states that a number is divisible by 3 if the sum of its digits is divisible by 3. In the case of 94, 9 + 4 = 13, which isn't divisible by 3. Therefore, 3 is not a factor.
- 4: A number is divisible by 4 if its last two digits are divisible by 4. 94's last two digits are 94, which isn't divisible by 4. So, 4 is not a factor.
- 5: A number is divisible by 5 if it ends in 0 or 5. 94 doesn't end in 0 or 5, so 5 is not a factor.
- 6: A number is divisible by 6 if it's divisible by both 2 and 3. Since 94 is divisible by 2 but not 3, 6 is not a factor.
- 7: There's no simple divisibility rule for 7. We need to perform the division: 94 divided by 7 is approximately 13.43, which is not a whole number. Therefore, 7 is not a factor.
- Continue this process: We continue this process, testing for divisibility by subsequent integers until we reach the square root of 94 (approximately 9.7). Once we surpass the square root, any new factor we find will have a corresponding factor that we've already identified.
Through this systematic approach, we find that the factors of 94 are 1, 2, 47, and 94.
Prime Factorization of 94
Prime factorization is the process of expressing a number as a product of its prime factors. A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself (e.g., 2, 3, 5, 7, 11, etc.). Prime factorization provides a unique representation of any number, making it a crucial tool in various mathematical applications.
To find the prime factorization of 94, we can start by dividing it by its smallest prime factor, which is 2:
94 = 2 × 47
Since 47 is itself a prime number, the prime factorization of 94 is simply 2 × 47.
Applications of Factorization
The concept of factorization and prime factorization isn't just an academic exercise; it has far-reaching applications in various areas:
1. Cryptography
Prime factorization forms the basis of many modern cryptographic systems. The difficulty of factoring large numbers into their prime factors is the foundation of the RSA algorithm, a widely used public-key cryptosystem that secures online communications and transactions.
2. Number Theory
Factorization is central to many areas of number theory, including the study of prime numbers, Diophantine equations (equations involving integers), and modular arithmetic.
3. Algebra
In algebra, factorization is a crucial technique used to simplify expressions, solve equations, and analyze functions. Factoring polynomials, for instance, is essential for finding roots of polynomial equations.
4. Computer Science
Efficient algorithms for factorization are crucial in computer science, particularly in cryptography and computational number theory. The development of faster factorization algorithms remains an active area of research.
Finding Factors using Different Methods
While the systematic approach described earlier is effective for smaller numbers like 94, it becomes inefficient for larger numbers. More sophisticated algorithms are necessary for efficient factorization of large integers. Some common methods include:
- Trial division: This is the basic method we used for 94; it's simple but inefficient for large numbers.
- Pollard's rho algorithm: A probabilistic algorithm that is more efficient than trial division for finding small prime factors.
- Quadratic sieve: A more advanced algorithm used to factor larger numbers.
- General number field sieve: Currently, the most efficient known algorithm for factoring very large numbers.
These advanced algorithms are critical in cryptography and related fields where factoring large numbers is computationally challenging.
The Significance of Prime Numbers in Factorization
Prime numbers are the building blocks of all integers. Every integer greater than 1 can be uniquely expressed as a product of prime numbers (Fundamental Theorem of Arithmetic). This unique factorization property is why prime numbers are so important in various mathematical fields and applications. Understanding prime factorization allows us to analyze the structure and properties of numbers in a fundamental way.
Conclusion: Beyond the Factors of 94
The seemingly simple question of "What are the factors of 94?" opens a door to a vast and fascinating world of number theory, cryptography, and computational mathematics. While finding the factors of 94 is straightforward, the underlying principles and algorithms extend to far more complex problems with significant real-world applications. The journey from identifying the simple factors 1, 2, 47, and 94 leads to a deeper understanding of the fundamental building blocks of numbers and their powerful implications in various fields. The exploration of factorization techniques, both elementary and advanced, highlights the elegance and practical significance of this seemingly simple arithmetic concept. From the basic divisibility rules to advanced algorithms used in modern cryptography, the factors of 94 serve as a gateway to a much richer mathematical landscape.
Latest Posts
Latest Posts
-
Which Of The Following Is An Alkane
Apr 08, 2025
-
Lewis Acid And Base Vs Bronsted
Apr 08, 2025
-
Diagram Of Bones Of The Foot
Apr 08, 2025
-
2 3 4 As An Improper Fraction
Apr 08, 2025
-
Find The Gcf Of 8 And 12
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about What Is The Factor Of 94 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.