Step By Step Implicit Differentiation Calculator
Juapaving
Mar 31, 2025 · 5 min read
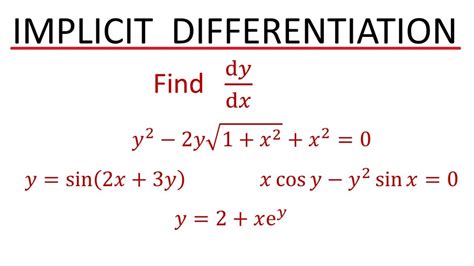
Table of Contents
Step-by-Step Implicit Differentiation Calculator: A Comprehensive Guide
Implicit differentiation is a powerful calculus technique used to find the derivative of a function that's not explicitly solved for y. While the process can seem daunting at first, understanding the steps involved, and even better, having a conceptual understanding of what you're doing, makes it manageable. This article serves as your comprehensive guide, walking you through the process step-by-step and providing insights into how to effectively use a (hypothetical) implicit differentiation calculator. We'll cover the theoretical underpinnings and then delve into practical examples to solidify your understanding.
Understanding Implicit Differentiation
Before we dive into the mechanics of using a calculator, let's understand the core concept. Explicit differentiation involves finding the derivative of a function where 'y' is explicitly defined in terms of 'x' (e.g., y = x² + 2x + 1). Implicit differentiation, on the other hand, deals with equations where 'y' is not explicitly defined (e.g., x² + y² = 25 – a circle).
The key idea behind implicit differentiation is to differentiate both sides of the equation with respect to 'x', treating 'y' as a function of 'x'. This requires applying the chain rule diligently. The chain rule states that the derivative of a composite function is the derivative of the outer function (with the inside function left alone) times the derivative of the inside function.
Step-by-Step Process of Implicit Differentiation
Let's outline the steps involved. Imagine our hypothetical implicit differentiation calculator follows this process:
Step 1: Differentiation of Both Sides
The first step involves differentiating both sides of the equation with respect to 'x'. This is where the chain rule comes into play. Remember to apply the chain rule whenever you differentiate a term containing 'y'.
Example: Consider the equation x² + y² = 25. Differentiating both sides with respect to x, we get:
2x + 2y(dy/dx) = 0
Notice that the derivative of y² with respect to x is 2y(dy/dx). This is because we're treating 'y' as a function of 'x', and hence the chain rule requires multiplying by the derivative of the inner function (which is dy/dx).
Step 2: Isolating dy/dx
Once you've differentiated both sides, the next step is to isolate dy/dx, which represents the derivative we're seeking. This often involves algebraic manipulation.
Continuing the Example: To isolate dy/dx from 2x + 2y(dy/dx) = 0:
- Subtract 2x from both sides: 2y(dy/dx) = -2x
- Divide both sides by 2y: dy/dx = -2x / 2y = -x/y
Step 3: Simplifying the Result (If Possible)
Finally, simplify the expression for dy/dx as much as possible. This may involve factoring, canceling terms, or other algebraic techniques.
In our example: dy/dx = -x/y is already in its simplest form.
Using a Hypothetical Implicit Differentiation Calculator
Let's assume our hypothetical implicit differentiation calculator has a user-friendly interface. The user would input the equation, and the calculator would perform the steps outlined above, displaying each step clearly.
Input: The user would input the equation, for example, x³ + y³ - 9xy = 0
Step 1 Display (Differentiation): The calculator would display the differentiated equation:
3x² + 3y²(dy/dx) - 9(x(dy/dx) + y) = 0
(Note: The product rule is applied to the term 9xy).
Step 2 Display (Isolation of dy/dx): The calculator would then show the steps to isolate dy/dx. This would likely involve a series of algebraic manipulations, neatly displayed step by step. The intermediate steps might include grouping terms with dy/dx, factoring it out, and finally solving for it. The result might be presented like this:
dy/dx = (3y - x²) / (y² - 3x)
Step 3 Display (Simplification): The calculator would display the simplified derivative, if possible.
Additional Features of a Hypothetical Calculator:
- Step-by-step explanation: Each step would have a clear explanation, detailing the rule or technique applied.
- Visualization: The calculator could provide a graphical representation of the equation and its derivative, helping users visualize the relationship.
- Multiple equation handling: The ability to handle multiple equations simultaneously or different types of implicit functions.
- Error handling: The calculator should gracefully handle invalid inputs or equations it cannot process.
- Advanced features: The calculator could also incorporate functionalities to handle higher-order derivatives or partial derivatives in multivariable calculus.
Advanced Implicit Differentiation Examples
Let's tackle more complex scenarios to further solidify our understanding:
Example 1: Equation with Trigonometric Functions
Consider the equation sin(x + y) = x + cos(y).
Step 1 (Differentiation): Applying the chain rule and the derivative rules for trigonometric functions, we get:
cos(x + y) * (1 + dy/dx) = 1 - sin(y) * dy/dx
Step 2 (Isolation of dy/dx): Algebraic manipulation would lead to:
dy/dx = (cos(x + y) - 1) / (sin(y) + cos(x + y))
Example 2: Equation with Exponential Functions
Let's work with e<sup>xy</sup> = x + y.
Step 1 (Differentiation): Using the chain rule and product rule:
e<sup>xy</sup> * (x(dy/dx) + y) = 1 + dy/dx
Step 2 (Isolation of dy/dx): After algebraic simplification:
dy/dx = (1 - ye<sup>xy</sup>) / (xe<sup>xy</sup> - 1)
Common Mistakes to Avoid
Several pitfalls can lead to errors in implicit differentiation. Being aware of these common mistakes is crucial:
- Forgetting the chain rule: The most common mistake is forgetting to multiply by dy/dx whenever differentiating a term containing 'y'.
- Incorrect algebraic manipulation: Errors in simplifying or isolating dy/dx can lead to incorrect results. Double-check your algebra!
- Not considering the product rule or quotient rule: When appropriate, always remember to apply the product rule or quotient rule for differentiation.
- Neglecting to simplify: While not always necessary for a correct answer, simplifying the final expression improves readability and understanding.
Conclusion
Implicit differentiation is a fundamental technique in calculus with broad applications. While the process can initially seem challenging, a structured approach, as outlined in this article, and the assistance of a (hypothetical) step-by-step implicit differentiation calculator can significantly ease the process. Understanding the underlying principles and diligently applying the chain rule and other differentiation rules are crucial to obtaining accurate results. By mastering implicit differentiation, you open up a world of possibilities in solving complex mathematical problems and gaining a deeper understanding of calculus. Remember to practice regularly, work through diverse examples, and don’t hesitate to revisit the step-by-step process when needed. This comprehensive guide, coupled with diligent practice, will equip you with the necessary skills to confidently tackle implicit differentiation problems.
Latest Posts
Latest Posts
-
Highest Common Factor Of 6 And 9
Apr 02, 2025
-
How Do You Find The Base Area
Apr 02, 2025
-
Are There Centrioles In Plant Cells
Apr 02, 2025
-
Common Factors Of 35 And 50
Apr 02, 2025
-
What Is The Difference Between Ac And Dc Motors
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Step By Step Implicit Differentiation Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
