Moment Of Inertia Of A Circle Formula
Juapaving
Apr 01, 2025 · 6 min read
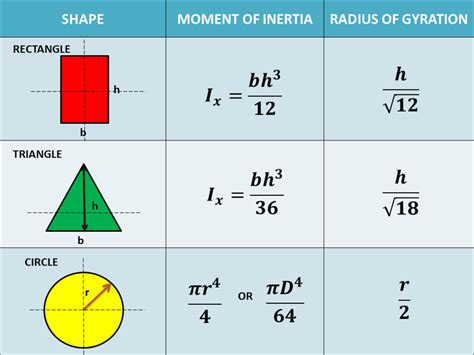
Table of Contents
Moment of Inertia of a Circle: A Comprehensive Guide
The moment of inertia, a crucial concept in physics and engineering, describes an object's resistance to changes in its rotation. Understanding the moment of inertia is essential for analyzing rotational motion, calculating angular momentum, and designing rotating machinery. This comprehensive guide delves into the moment of inertia of a circle, exploring its derivation, various scenarios (solid disc, hollow disc, ring), practical applications, and related concepts.
Understanding Moment of Inertia
Before diving into the specifics of a circle, let's establish a foundational understanding of moment of inertia. Unlike mass, which represents resistance to linear acceleration, the moment of inertia (often denoted as I) represents resistance to angular acceleration. It depends not only on the object's mass but also on how that mass is distributed relative to the axis of rotation. The further the mass is from the axis, the greater the moment of inertia, and thus, the harder it is to change its rotational speed.
Mathematically, the moment of inertia is calculated by summing the products of each infinitesimal mass element (dm) and the square of its distance (r) from the axis of rotation:
I = ∫ r² dm
This integral represents the summation across the entire object. The complexity of this integral depends heavily on the object's shape and the chosen axis of rotation.
Moment of Inertia of a Solid Circular Disc
A solid circular disc is a common geometrical shape frequently encountered in physics and engineering problems. Let's derive the formula for its moment of inertia about an axis perpendicular to the disc and passing through its center.
We can consider the disc as a collection of concentric rings. Each ring has a radius r, thickness dr, and density ρ. The mass of an individual ring is:
dm = ρ * 2πr * dr (where ρ = M/πR²) is the area density)
Substituting this into the general moment of inertia equation:
I = ∫₀ᴿ (r²) (ρ * 2πr * dr)
Solving this integral, we arrive at the formula for the moment of inertia of a solid circular disc about an axis through its center:
I = ½ * M * R²
Where:
- I is the moment of inertia
- M is the total mass of the disc
- R is the radius of the disc
This formula tells us that the moment of inertia is directly proportional to the mass and the square of the radius. A larger mass or a larger radius results in a larger moment of inertia.
Practical Applications of the Solid Disc Formula
The formula for the moment of inertia of a solid circular disc finds extensive application in various fields:
- Mechanical Engineering: Designing flywheels for energy storage, analyzing rotating shafts, calculating torques in gears.
- Automotive Engineering: Modeling wheels and tires during braking and acceleration, analyzing vehicle stability.
- Robotics: Designing robotic arms and joints, calculating required torques for robotic movements.
- Physics Experiments: Analyzing rotational motion experiments, validating theoretical models.
Moment of Inertia of a Hollow Circular Disc (Ring)
A hollow circular disc, or ring, represents a different mass distribution compared to a solid disc. All the mass is concentrated at a single radius. The derivation of the moment of inertia for a ring is simpler, as we don't need to integrate over a range of radii:
The mass of the ring is simply:
M = ρ * 2πR * t (where t is thickness and R is radius)
Assuming the thickness is negligible compared to the radius, we can treat the mass as concentrated at the radius R.
Therefore, the moment of inertia of a thin ring about an axis perpendicular to the plane and passing through its center is:
I = M * R²
Observe that the moment of inertia of a ring is twice that of a solid disc of the same mass and radius. This is because all the mass is concentrated at the maximum distance from the axis of rotation.
Applications of the Hollow Disc Formula
The moment of inertia of a ring or hollow disc is relevant in scenarios involving:
- Flywheels: Hollow cylinders are often preferred for flywheels because of their higher moment of inertia for a given mass, making them more effective at storing rotational energy.
- Gyroscopes: The design and performance of gyroscopes rely heavily on the moment of inertia of their rotating components.
- Mechanical Seals: Understanding the moment of inertia helps in designing efficient and reliable mechanical seals that minimize friction and wear.
Moment of Inertia of a Circular Disc About a Diameter
The moment of inertia of a circular disc also changes depending on the axis of rotation. If we consider the axis of rotation to be a diameter of the disc, the calculation becomes slightly more complex, requiring integration in two dimensions. The result is:
I = (1/4) * M * R²
This value is exactly half the moment of inertia about the axis perpendicular to the plane of the disc and passing through its center. This illustrates how mass distribution relative to the axis significantly impacts the moment of inertia.
Applications of Diameteral Axis Formula
This formula is important in applications where the disc rotates about a diameter, such as:
- Rotating Shafts with Off-Center Loads: Analyzing the stresses and strains in a rotating shaft when a load is applied eccentrically.
- Gyroscopic Precession: Understanding the precessional motion of a spinning object, particularly when the rotation axis is not fixed.
Parallel Axis Theorem
The parallel axis theorem provides a valuable tool for calculating the moment of inertia about an axis parallel to an axis through the center of mass. If I<sub>cm</sub> is the moment of inertia about an axis passing through the center of mass and I is the moment of inertia about a parallel axis a distance d away, then:
I = I<sub>cm</sub> + M * d²
This theorem simplifies calculations significantly, particularly when dealing with objects rotating about axes not passing through their center of mass. For example, it can be used to determine the moment of inertia of a disc rotating about an axis along its edge.
Radius of Gyration
The radius of gyration (k) is a useful concept related to the moment of inertia. It represents the distance from the axis of rotation at which the entire mass of the object could be concentrated to produce the same moment of inertia. It's defined as:
k = √(I/M)
The radius of gyration provides a concise way to compare the mass distribution of different objects concerning their rotational inertia.
Conclusion
The moment of inertia of a circle, whether a solid disc, hollow disc, or ring, is a fundamental concept with wide-ranging applications across various engineering disciplines and physics. Understanding its derivation, the impact of mass distribution, and the use of the parallel axis theorem allows for accurate analysis and design of rotating systems. The formulas presented here provide the essential tools for tackling a wide range of problems involving rotational motion. Mastering these concepts is crucial for anyone working with rotating machinery, designing mechanical systems, or exploring the intricacies of rotational dynamics. Furthermore, the detailed derivations provided, and the emphasis on practical applications, aid in solidifying understanding and promoting real-world application of the theoretical knowledge. Remember to always consider the specific geometry and axis of rotation when determining the moment of inertia. This understanding forms a cornerstone of advanced engineering and physics principles.
Latest Posts
Latest Posts
-
Do The Diagonals Of A Kite Bisect Bisect The Angles
Apr 03, 2025
-
Peasants And Workers Party Of India
Apr 03, 2025
-
Compressions And Rarefactions Are Characteristics Of
Apr 03, 2025
-
Explain The Process Of Making Yarn From Fibre Answer
Apr 03, 2025
-
Does Lemon Juice Dissolve In Water
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Moment Of Inertia Of A Circle Formula . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
