Is 18 A Prime Or Composite Number
Juapaving
Apr 06, 2025 · 6 min read
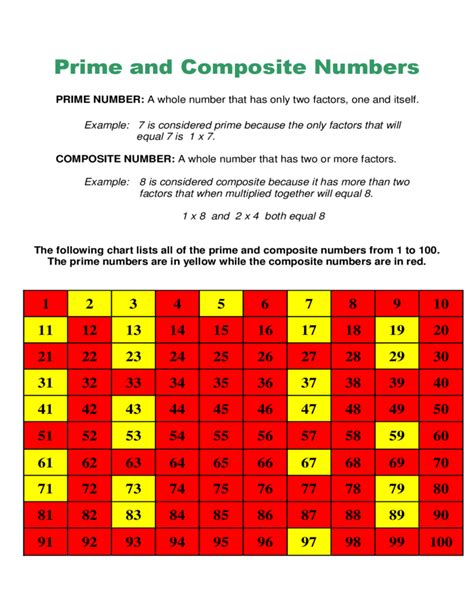
Table of Contents
Is 18 a Prime or Composite Number? A Deep Dive into Number Theory
Determining whether a number is prime or composite is a fundamental concept in number theory. This seemingly simple question—is 18 a prime or composite number?—opens the door to exploring fascinating mathematical properties and their applications. Let's delve into the definition of prime and composite numbers, explore the factors of 18, and then definitively answer the question, solidifying our understanding of these core mathematical concepts.
Understanding Prime and Composite Numbers
Before we tackle the specific case of 18, let's establish a clear understanding of prime and composite numbers. These classifications are based on the number's divisors, or the numbers that divide it evenly without leaving a remainder.
Prime Numbers: The Building Blocks of Arithmetic
A prime number is a natural number greater than 1 that has only two distinct positive divisors: 1 and itself. This means it's not divisible by any other number except 1 and the number itself. Prime numbers are the fundamental building blocks of all other integers through a process called prime factorization. Examples of prime numbers include 2, 3, 5, 7, 11, and so on. Note that 1 is neither prime nor composite; it's a special case.
Composite Numbers: Products of Primes
A composite number is a natural number greater than 1 that is not a prime number. In other words, a composite number has more than two positive divisors. This means it can be factored into smaller whole numbers. Every composite number can be uniquely expressed as a product of prime numbers, a concept known as the Fundamental Theorem of Arithmetic. For example, 12 is a composite number because it's divisible by 1, 2, 3, 4, 6, and 12. Its prime factorization is 2 x 2 x 3 (or 2² x 3).
The Importance of Prime and Composite Numbers
The distinction between prime and composite numbers is crucial in various areas of mathematics and computer science:
-
Cryptography: Prime numbers form the foundation of many modern encryption methods, ensuring secure online transactions and data protection. The difficulty of factoring large composite numbers into their prime components is the basis for the security of these systems.
-
Number Theory: Prime numbers are a central topic in number theory, a branch of mathematics that studies the properties of integers. Many unsolved problems in mathematics, such as the Riemann Hypothesis, involve prime numbers.
-
Algorithms and Computing: Algorithms for factoring large numbers into primes are computationally intensive and have practical applications in areas like cryptography and computational number theory.
-
Abstract Algebra: Prime numbers and their properties play a critical role in abstract algebra, a field that deals with algebraic structures and their properties.
Determining if 18 is Prime or Composite
Now, let's address the central question: Is 18 a prime or composite number? To determine this, we need to find all the divisors of 18.
The divisors of 18 are: 1, 2, 3, 6, 9, and 18.
Since 18 has more than two divisors (it has six divisors), it fulfills the definition of a composite number. Therefore, 18 is a composite number.
Finding the Prime Factorization of 18
We can express 18 as a product of prime numbers:
18 = 2 x 9 = 2 x 3 x 3 = 2 x 3²
This confirms that 18 is composite, as it's expressed as a product of prime numbers (2 and 3). This prime factorization is unique according to the Fundamental Theorem of Arithmetic.
Expanding on Composite Numbers and Their Properties
Composite numbers exhibit a rich variety of properties and relationships within number theory. Let's explore some key aspects:
Abundance of Composite Numbers
Unlike prime numbers, which become increasingly sparse as you move to larger numbers, composite numbers are significantly more abundant. As you consider larger ranges of numbers, the proportion of composite numbers compared to prime numbers drastically increases.
Divisibility Rules and Factorization Techniques
Identifying composite numbers often involves applying divisibility rules. For example, if a number is even (divisible by 2), it's automatically composite (except for the number 2 itself). Similarly, if a number is divisible by 3 (the sum of its digits is divisible by 3), it's composite unless it's 3 itself. There are divisibility rules for other numbers as well, which aids in quick identification of factors.
Efficient algorithms exist for prime factorization, but for very large numbers, these algorithms can become computationally expensive. This complexity is what makes RSA encryption, which relies on the difficulty of factoring large composite numbers, so secure.
Perfect Numbers and Abundant Numbers
Some composite numbers possess unique properties. Perfect numbers are positive integers that are equal to the sum of their proper divisors (excluding the number itself). For example, 6 is a perfect number (1 + 2 + 3 = 6). Abundant numbers are positive integers where the sum of their proper divisors is greater than the number itself. For example, 12 is abundant (1 + 2 + 3 + 4 + 6 = 16 > 12).
Applications in Cryptography
The difficulty in factoring large composite numbers into their prime factors underpins the security of many public-key cryptosystems. RSA encryption, for instance, relies on this computational challenge. The keys used in RSA are based on the product of two very large prime numbers. Breaking the encryption would require factoring this extremely large composite number, a task that is computationally infeasible with current technology for sufficiently large numbers.
Beyond 18: Exploring Other Composite Numbers
Let's expand our understanding by looking at other composite numbers and their characteristics. Consider the following:
-
24: 24 is a composite number because it's divisible by 1, 2, 3, 4, 6, 8, 12, and 24. Its prime factorization is 2³ x 3.
-
36: 36 is a composite number with divisors 1, 2, 3, 4, 6, 9, 12, 18, and 36. Its prime factorization is 2² x 3².
-
100: 100 is a composite number (divisors 1, 2, 4, 5, 10, 20, 25, 50, 100) with a prime factorization of 2² x 5².
These examples showcase the diversity of composite numbers and how their prime factorizations reveal their structure.
Conclusion: The Significance of 18's Composite Nature
In conclusion, 18 is definitively a composite number. Its possession of more than two divisors and its expressible prime factorization (2 x 3²) firmly place it within the category of composite numbers. Understanding the difference between prime and composite numbers is essential for grasping fundamental concepts in mathematics, and 18 serves as a perfect example for illustrating these principles. The seemingly simple question, "Is 18 a prime or composite number?", unlocks a world of mathematical exploration, revealing the intricate relationships and profound implications of these fundamental number classifications within various fields, including cryptography and computer science. The journey from the simple identification of 18 as composite extends to the vast and fascinating realm of number theory, highlighting the importance of these seemingly basic concepts in both theoretical mathematics and practical applications.
Latest Posts
Latest Posts
-
Sum Of Interior Angles Of A Nonagon
Apr 07, 2025
-
Which Of The Planet Is Farthest From The Sun
Apr 07, 2025
-
Find Three Consecutive Even Integers Whose Sum Is 108
Apr 07, 2025
-
What Is The Most Abundant Gas In Earths Atmosphere
Apr 07, 2025
-
Is Phosphorus A Metal Or Nonmetal
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Is 18 A Prime Or Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
