How Do You Factorize An Equation
Juapaving
Apr 02, 2025 · 5 min read
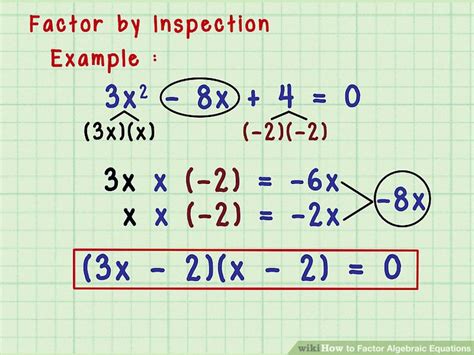
Table of Contents
How Do You Factorize an Equation? A Comprehensive Guide
Factoring equations is a fundamental skill in algebra, crucial for solving higher-degree equations, simplifying expressions, and understanding various mathematical concepts. While the process might seem daunting at first, with a systematic approach and understanding of different techniques, factorization becomes manageable and even enjoyable. This comprehensive guide will walk you through various methods, providing examples and tips to master the art of equation factorization.
Understanding Factorization
Before diving into specific techniques, let's clarify what factorization means. Essentially, it's the process of breaking down a complex expression into simpler expressions that, when multiplied together, produce the original expression. Think of it as the reverse of expanding brackets. For example, factoring the expression x² + 5x + 6 would result in (x + 2)(x + 3). The factors are (x + 2) and (x + 3).
Why is factorization important?
-
Solving Equations: Factoring quadratic and higher-degree equations allows us to find their roots (the values of the variable that make the equation true). This is achieved by setting each factor to zero and solving the resulting simpler equations.
-
Simplifying Expressions: Factoring can significantly simplify complex algebraic expressions, making them easier to manipulate and analyze.
-
Analyzing Functions: Factorization helps in understanding the behavior of functions, such as identifying intercepts, asymptotes, and turning points.
-
Foundation for Advanced Math: Factoring forms the basis for more advanced mathematical concepts, including calculus, differential equations, and linear algebra.
Common Factoring Techniques
Various methods exist for factoring equations, and the best approach often depends on the specific form of the equation. Here are some of the most commonly used techniques:
1. Greatest Common Factor (GCF)
This is the simplest form of factorization. It involves identifying the greatest common factor among all terms in the expression and factoring it out.
Example:
Factorize 3x² + 6x.
The GCF of 3x² and 6x is 3x. Factoring it out, we get:
3x(x + 2)
2. Factoring Quadratic Trinomials (ax² + bx + c)
Quadratic trinomials are expressions of the form ax² + bx + c, where 'a', 'b', and 'c' are constants. Factoring these trinomials often involves finding two numbers that add up to 'b' and multiply to 'ac'.
Example:
Factorize x² + 5x + 6.
We need two numbers that add up to 5 (the coefficient of x) and multiply to 6 (the constant term). These numbers are 2 and 3. Therefore, the factorization is:
(x + 2)(x + 3)
Example with 'a' not equal to 1:
Factorize 2x² + 7x + 3.
Here, we need two numbers that add up to 7 and multiply to 2 * 3 = 6. These numbers are 6 and 1. We can rewrite the expression as:
2x² + 6x + x + 3
Now, we factor by grouping:
2x(x + 3) + 1(x + 3)
(2x + 1)(x + 3)
3. Difference of Squares
This method applies to expressions of the form a² - b², which factorizes to (a + b)(a - b).
Example:
Factorize x² - 9.
This is a difference of squares, where a = x and b = 3. Therefore, the factorization is:
(x + 3)(x - 3)
4. Sum and Difference of Cubes
These methods apply to expressions of the form a³ + b³ and a³ - b³. The factorizations are:
- Sum of Cubes:
a³ + b³ = (a + b)(a² - ab + b²) - Difference of Cubes:
a³ - b³ = (a - b)(a² + ab + b²)
Example:
Factorize x³ - 8.
This is a difference of cubes, where a = x and b = 2. Therefore, the factorization is:
(x - 2)(x² + 2x + 4)
5. Factoring by Grouping
This method is useful when dealing with expressions containing four or more terms. It involves grouping terms with common factors and then factoring out the common factors from each group.
Example:
Factorize xy + 2x + 3y + 6.
Group the terms:
(xy + 2x) + (3y + 6)
Factor out common factors from each group:
x(y + 2) + 3(y + 2)
Now, factor out the common factor (y + 2):
(x + 3)(y + 2)
6. Using the Quadratic Formula
For quadratic equations that are difficult to factor using the methods above, the quadratic formula can be used to find the roots. Once the roots are known, the factorization can be determined.
The quadratic formula is:
x = (-b ± √(b² - 4ac)) / 2a
Where 'a', 'b', and 'c' are the coefficients of the quadratic equation ax² + bx + c = 0.
Advanced Factoring Techniques
Beyond the fundamental methods, several more advanced techniques can be employed for more complex expressions. These often involve a combination of the basic methods or require a deeper understanding of algebraic manipulation.
1. Factoring Higher-Degree Polynomials
Factoring polynomials of degree three or higher can be significantly more challenging. Strategies often involve using the rational root theorem to find potential rational roots, then using polynomial long division or synthetic division to reduce the polynomial's degree.
2. Factoring with Complex Numbers
Some quadratic equations have no real roots but possess complex roots. These roots involve the imaginary unit 'i' (√-1). Factoring in such cases involves complex numbers.
3. Partial Fraction Decomposition
This technique is used to decompose rational functions (fractions with polynomials in the numerator and denominator) into simpler fractions. It's crucial in calculus and other advanced mathematical fields.
Tips and Tricks for Successful Factoring
- Always look for the greatest common factor (GCF) first. This simplifies the expression and often makes subsequent factorization easier.
- Practice regularly. The more you practice, the faster and more efficient you'll become at recognizing different factoring patterns.
- Check your work. Expand the factored expression to ensure it matches the original expression.
- Use online resources and calculators. Many online tools can help you check your work or provide guidance on more challenging factoring problems. Remember to understand the process, not just the answer.
- Break down complex problems into smaller, manageable steps. This helps to avoid errors and improve understanding.
- Master the fundamental techniques before moving on to more advanced methods. A strong foundation is key to success in algebra and beyond.
Conclusion
Factoring equations is a core skill in algebra. While the process might initially seem complex, mastering various techniques, from the simple GCF to more advanced methods like polynomial long division and partial fraction decomposition, opens doors to solving a wider range of mathematical problems. Consistent practice and a systematic approach are crucial to developing proficiency. By understanding the underlying principles and practicing regularly, you can confidently tackle even the most challenging factoring problems. Remember, the key is not just getting the right answer but understanding the why behind the steps. This deep understanding will serve as a solid foundation for your future mathematical endeavors.
Latest Posts
Latest Posts
-
How Is Photosynthesis And Cellular Respiration Different
Apr 03, 2025
-
Which Of The Following Is Strongest Acid
Apr 03, 2025
-
Are Prime Numbers Even Or Odd
Apr 03, 2025
-
What Is 104 Degrees Fahrenheit In Celsius
Apr 03, 2025
-
Fossil Fuels Are Classified As Non Renewable Because They
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How Do You Factorize An Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
