1 Out Of 10 As A Percentage
Juapaving
Apr 03, 2025 · 5 min read
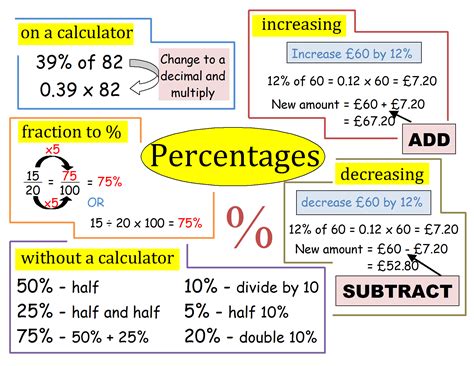
Table of Contents
1 Out of 10 as a Percentage: A Comprehensive Guide
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. One common scenario that often requires percentage calculation is expressing a ratio like "1 out of 10" as a percentage. This seemingly simple conversion can be confusing for some, but with a clear understanding of the underlying principles, it becomes straightforward. This article will delve into the concept of representing "1 out of 10" as a percentage, providing a comprehensive guide with practical examples and exploring its implications in different contexts.
Understanding Percentages
Before diving into the specific calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The word "percent" itself originates from the Latin "per centum," meaning "out of one hundred." Therefore, any percentage can be easily converted into a fraction or a decimal. For instance:
- 50% is equal to 50/100, which simplifies to 1/2, or 0.5 as a decimal.
- 25% is equal to 25/100, which simplifies to 1/4, or 0.25 as a decimal.
- 10% is equal to 10/100, which simplifies to 1/10, or 0.1 as a decimal.
Calculating 1 Out of 10 as a Percentage
To convert "1 out of 10" into a percentage, we need to express this ratio as a fraction and then convert it to a percentage. The process is as follows:
-
Express the ratio as a fraction: "1 out of 10" can be written as the fraction 1/10.
-
Convert the fraction to a decimal: To do this, divide the numerator (1) by the denominator (10): 1 ÷ 10 = 0.1
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the "%" symbol: 0.1 x 100 = 10%.
Therefore, 1 out of 10 is equal to 10%.
Practical Applications of 10%
Understanding that 1 out of 10 equals 10% has numerous practical applications across various fields. Here are some examples:
1. Sales and Discounts:
Imagine a store offering a 10% discount on all items. If you buy an item worth $100, you'll receive a discount of $10 (10% of $100). This simple calculation relies on the understanding that 10% represents 1 out of 10.
2. Statistics and Data Analysis:
In surveys and polls, 10% often represents a significant portion of the respondents. For example, if 10% of 1000 respondents answered "yes" to a particular question, it means 100 respondents chose "yes." This data point is crucial for understanding trends and drawing meaningful conclusions.
3. Financial Calculations:
Interest rates, tax rates, and investment returns are often expressed as percentages. Understanding the relationship between fractions and percentages is critical for making informed financial decisions. For example, if you invest $1000 and earn a 10% return, you'll earn an additional $100.
4. Probability and Chance:
In probability, percentages help us understand the likelihood of events. If there's a 10% chance of rain, it means that out of 10 similar days, you can expect it to rain on approximately one of them.
Expanding the Concept: Variations on the Theme
While we've focused on "1 out of 10," the same principles can be applied to other ratios. Let's explore some variations:
1. 2 out of 10:
- Fraction: 2/10
- Decimal: 2 ÷ 10 = 0.2
- Percentage: 0.2 x 100 = 20%
Therefore, 2 out of 10 is equal to 20%.
2. 3 out of 10:
- Fraction: 3/10
- Decimal: 3 ÷ 10 = 0.3
- Percentage: 0.3 x 100 = 30%
Therefore, 3 out of 10 is equal to 30%.
3. x out of 10:
The general formula for calculating "x out of 10" as a percentage is:
(x/10) * 100%
This formula can be used to calculate the percentage for any value of 'x' between 0 and 10.
Beyond 10: Calculating Percentages from Other Ratios
The fundamental principle of converting a ratio to a percentage remains the same, regardless of the denominator. Let's look at an example:
Example: What is 3 out of 20 as a percentage?
- Fraction: 3/20
- Decimal: 3 ÷ 20 = 0.15
- Percentage: 0.15 x 100 = 15%
Therefore, 3 out of 20 is equal to 15%.
Common Mistakes to Avoid
While the process is straightforward, several common mistakes can occur when calculating percentages:
-
Incorrect Fraction Formation: Ensure the ratio is correctly expressed as a fraction before proceeding with the calculation. The "out of" part represents the denominator.
-
Division Errors: Double-check your division when converting the fraction to a decimal. A simple calculator can help avoid errors.
-
Multiplication Errors: Carefully multiply the decimal by 100 when converting to a percentage.
-
Forgetting the Percentage Symbol: Always include the "%" symbol to denote that the result is a percentage.
Conclusion: Mastering Percentage Calculations
The ability to convert ratios like "1 out of 10" into percentages is an essential skill with wide-ranging applications. By understanding the underlying principles of fractions, decimals, and percentages, and by following the steps outlined in this guide, you can confidently perform these calculations in various scenarios. Remember to practice regularly and to double-check your work to avoid common errors. Mastering percentage calculations will significantly enhance your ability to analyze data, make informed decisions, and navigate various aspects of life and work more effectively. The seemingly simple conversion of "1 out of 10" to 10% unlocks a world of practical understanding and application.
Latest Posts
Latest Posts
-
Cbse Class 6th Maths Book Pdf
Apr 04, 2025
-
How Many Atoms Are In Carbon
Apr 04, 2025
-
3 632 Rounded To The Nearest Tenth
Apr 04, 2025
-
2550 50 100 20 100 100 200
Apr 04, 2025
-
What Are Two Kinds Of Fermentation
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 1 Out Of 10 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
