What Triangle Has 2 Equal Sides
Juapaving
Mar 28, 2025 · 5 min read
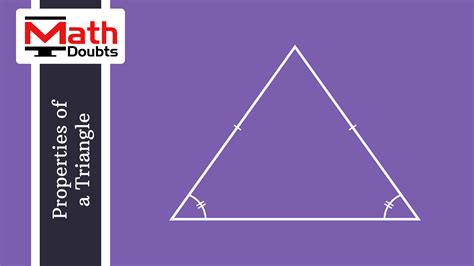
Table of Contents
What Triangle Has 2 Equal Sides? A Deep Dive into Isosceles Triangles
The question, "What triangle has 2 equal sides?" leads us directly to the fascinating world of isosceles triangles. This seemingly simple geometric shape holds a wealth of properties and applications, extending far beyond basic geometry into more advanced mathematical concepts and even real-world scenarios. This comprehensive guide will explore the definition, properties, theorems, and practical applications of isosceles triangles, providing a deep understanding of this fundamental geometric figure.
Defining the Isosceles Triangle
An isosceles triangle is a triangle with at least two sides of equal length. These equal sides are called legs, and the angle formed by the two legs is called the vertex angle. The side opposite the vertex angle is called the base. It's crucial to understand the "at least" part of the definition: while most commonly envisioned, an equilateral triangle (with all three sides equal) is also considered a special case of an isosceles triangle. This inclusive definition simplifies many theorems and proofs.
Distinguishing Isosceles from Other Triangles
It's important to differentiate isosceles triangles from other types:
- Equilateral Triangle: All three sides are equal in length, and all three angles are 60 degrees.
- Scalene Triangle: All three sides have different lengths.
- Right-Angled Triangle: One angle is a right angle (90 degrees). A right-angled triangle can be isosceles (e.g., a 45-45-90 triangle) or scalene.
Key Properties of Isosceles Triangles
Isosceles triangles possess several unique and significant properties:
- Base Angles are Equal: The two angles opposite the equal sides (the base angles) are always equal in measure. This is a fundamental theorem in geometry and forms the basis for many proofs related to isosceles triangles.
- Altitude from the Vertex Angle Bisects the Base: The line segment drawn from the vertex angle perpendicular to the base bisects the base; it divides the base into two equal segments. This altitude also bisects the vertex angle.
- Median from the Vertex Angle Bisects the Base: The line segment from the vertex angle to the midpoint of the base bisects the base. This median is congruent to the altitude from the vertex angle in an isosceles triangle.
- Angle Bisector from the Vertex Angle Bisects the Base: The line segment that bisects the vertex angle also bisects the base. This means the altitude, median, and angle bisector from the vertex angle are all the same line segment.
Theorems Related to Isosceles Triangles
Several important geometric theorems revolve around isosceles triangles:
- Isosceles Triangle Theorem: This theorem states that if two sides of a triangle are congruent (equal in length), then the angles opposite those sides are congruent (equal in measure). This is a cornerstone of isosceles triangle geometry.
- Converse of the Isosceles Triangle Theorem: This theorem states that if two angles of a triangle are congruent, then the sides opposite those angles are congruent. This theorem provides a method to prove that a triangle is isosceles based on its angles.
- The Triangle Inequality Theorem: While not exclusive to isosceles triangles, this theorem applies to all triangles. It states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. This is crucial for determining the feasibility of constructing a triangle given side lengths.
Applications of Isosceles Triangles
Isosceles triangles appear frequently in various fields:
- Architecture and Design: Many architectural structures, from roofs to bridges, utilize isosceles triangles for their structural strength and aesthetic appeal. The symmetrical nature of isosceles triangles lends itself well to balanced and visually pleasing designs. Think of the triangular gable ends often found on houses.
- Engineering: Isosceles triangles play a role in structural engineering, particularly in truss systems and bridge designs, where their strength and stability are crucial.
- Art and Design: The balanced and symmetrical nature of isosceles triangles is utilized in art and graphic design to create aesthetically pleasing compositions.
- Computer Graphics: Isosceles triangles are fundamental building blocks in computer graphics, used in creating various shapes and patterns.
- Nature: Isosceles triangles can be found in natural formations, though often imperfectly. Crystalline structures sometimes exhibit isosceles triangular patterns.
Solving Problems Involving Isosceles Triangles
Numerous problems in geometry involve isosceles triangles. Successfully solving these problems often requires applying the properties and theorems discussed earlier. Here are some common problem types:
- Finding Missing Angles: Given one angle of an isosceles triangle (typically the vertex angle or a base angle), you can readily find the other angles using the property that the base angles are equal and the sum of angles in a triangle is 180 degrees.
- Finding Missing Sides: Using the Pythagorean theorem (if it's a right-angled isosceles triangle) or the Law of Cosines or the Law of Sines (for general isosceles triangles), you can determine the lengths of unknown sides.
- Proofs: Many geometry problems involve proving properties or relationships related to isosceles triangles. These proofs often leverage the isosceles triangle theorem, its converse, and other geometric axioms and postulates.
Advanced Concepts and Related Topics
The study of isosceles triangles extends to more advanced concepts:
- Area Calculation: The area of an isosceles triangle can be calculated using the standard formula: Area = (1/2) * base * height, where the height is the altitude from the vertex angle to the base.
- Circumradius and Inradius: The circumradius (radius of the circumscribed circle) and inradius (radius of the inscribed circle) of an isosceles triangle can be calculated using specific formulas derived from its properties.
- Isosceles Triangles in Coordinate Geometry: Isosceles triangles can be defined and analyzed using coordinate geometry, allowing for algebraic solutions to geometric problems.
Conclusion: The Enduring Significance of Isosceles Triangles
The isosceles triangle, though seemingly simple, holds a significant place in geometry and its applications. Its unique properties, coupled with the associated theorems, provide a powerful toolset for solving a wide range of geometric problems. From its role in structural engineering to its presence in artistic compositions, the isosceles triangle’s influence extends far beyond the realm of theoretical mathematics, demonstrating its enduring relevance and practical significance. A deep understanding of its properties and characteristics is essential for anyone pursuing a serious study of geometry or its related fields. This exploration has only scratched the surface of the rich mathematical landscape associated with this fundamental geometric shape, revealing its surprising depth and continued relevance in diverse areas of study and application. Further investigation into advanced topics and specialized applications will undoubtedly uncover even more fascinating facets of the isosceles triangle.
Latest Posts
Latest Posts
-
Suez Canal Connects Which Two Bodies Of Water
Mar 31, 2025
-
How Many Vertices Do A Square Have
Mar 31, 2025
-
Lcm Of 3 9 And 12
Mar 31, 2025
-
How Many Feet In 95 Inches
Mar 31, 2025
-
350 Square Meters In Square Feet
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Triangle Has 2 Equal Sides . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
