What Percentage Is 1 Out Of 3
Juapaving
Apr 02, 2025 · 5 min read
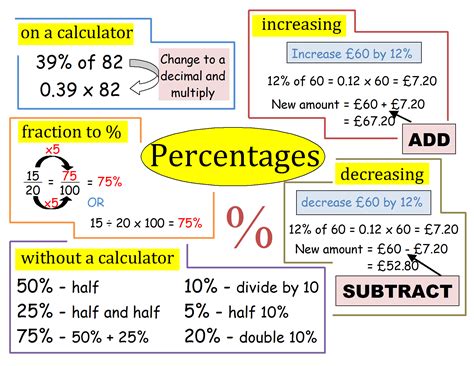
Table of Contents
What Percentage is 1 out of 3? A Comprehensive Guide to Fractions, Percentages, and Ratios
Understanding fractions, percentages, and ratios is fundamental to various aspects of life, from everyday calculations to complex financial analyses. One common question that often arises is: "What percentage is 1 out of 3?" This seemingly simple query opens the door to a broader exploration of these interconnected mathematical concepts. This comprehensive guide will not only answer this question but also equip you with the skills to tackle similar problems confidently.
Understanding the Basics: Fractions, Percentages, and Ratios
Before delving into the specific calculation, let's establish a clear understanding of the core concepts:
Fractions: Representing Parts of a Whole
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). In the question "What percentage is 1 out of 3?", the fraction is 1/3. The numerator (1) represents the part, and the denominator (3) represents the whole.
Percentages: Expressing Fractions as Hundredths
A percentage is a fraction expressed as a part of 100. The symbol "%" represents "per cent" or "out of 100". To convert a fraction to a percentage, you multiply the fraction by 100%.
Ratios: Comparing Quantities
A ratio compares two or more quantities. It can be expressed using a colon (e.g., 1:3) or as a fraction (e.g., 1/3). In our example, the ratio of 1 to 3 represents the comparison between the part (1) and the whole (3).
Calculating the Percentage: 1 out of 3
Now, let's tackle the central question: What percentage is 1 out of 3?
To find the percentage, we follow these steps:
-
Express the fraction: The given information is "1 out of 3," which translates to the fraction 1/3.
-
Convert the fraction to a decimal: Divide the numerator (1) by the denominator (3): 1 ÷ 3 = 0.3333... (This is a recurring decimal).
-
Convert the decimal to a percentage: Multiply the decimal by 100%: 0.3333... × 100% ≈ 33.33%.
Therefore, 1 out of 3 is approximately 33.33%. The "..." indicates that the decimal 0.3333 continues infinitely. For practical purposes, rounding to two decimal places is usually sufficient.
Practical Applications: Real-World Examples
Understanding percentages is crucial in various real-world scenarios. Here are a few examples demonstrating the practical application of calculating percentages:
1. Sales and Discounts:
Imagine a store offering a discount of 1 out of 3 on all items. This means a discount of approximately 33.33%. If an item costs $90, the discount would be $90 × 0.3333 ≈ $30, making the final price $60.
2. Survey Results:
Suppose a survey of 300 people shows that 100 people prefer a particular brand. To express this as a percentage, we calculate 100/300 = 1/3 ≈ 33.33%. Therefore, approximately 33.33% of the surveyed people prefer that brand.
3. Test Scores:
If a test has 3 questions, and a student answers 1 question correctly, their score is 1/3 or approximately 33.33%.
4. Financial Investments:
In financial investments, understanding percentages is vital for tracking returns, calculating interest, and analyzing growth rates. If an investment grows by 1 out of 3 of its initial value, it has grown by approximately 33.33%.
5. Data Analysis:
In data analysis, percentages are commonly used to represent proportions and trends within datasets. For example, if 1 out of 3 data points falls within a specific range, this represents approximately 33.33% of the data.
Beyond the Basics: Working with More Complex Scenarios
The fundamental principle of converting fractions to percentages remains consistent even with more complex scenarios. Let's explore some variations:
Calculating Percentages with Larger Numbers:
The process remains the same regardless of the magnitude of the numbers. For example, if we have 100 out of 300 items, the fraction is 100/300, which simplifies to 1/3, leading to the same 33.33% result.
Dealing with Recurring Decimals:
The recurring decimal 0.333... can be represented as 1/3. Understanding this equivalence is crucial for accurate calculations and avoids rounding errors in more complex problems.
Combining Percentages:
In scenarios involving multiple percentages, it's essential to follow the order of operations carefully. For instance, if you have a 10% discount followed by a further 33.33% discount, the total discount is not simply the sum of the two percentages. The second discount is applied to the already discounted price.
Practical Tips and Tricks for Percentage Calculations
Here are some helpful tips to enhance your percentage calculation skills:
- Master the basics: A strong foundation in fractions and decimals is crucial for accurate percentage calculations.
- Use a calculator: For complex calculations, use a calculator to ensure accuracy, especially when dealing with recurring decimals.
- Learn common percentage equivalents: Memorizing common fraction-to-percentage conversions (e.g., 1/4 = 25%, 1/2 = 50%, 3/4 = 75%) will speed up your calculations.
- Practice regularly: Consistent practice with various percentage problems will improve your understanding and proficiency.
- Utilize online resources: Many websites and educational platforms offer interactive exercises and tutorials to enhance your understanding of percentages.
Conclusion: Mastering Percentages for Success
Understanding what percentage is 1 out of 3—approximately 33.33%—is a foundational step in mastering percentages. The ability to convert fractions to percentages and vice-versa is essential in various aspects of life. This guide has provided not only the answer to the central question but also equipped you with the knowledge and skills to confidently tackle diverse percentage-related problems. Remember that consistent practice and a strong grasp of the underlying mathematical concepts are key to achieving mastery in this critical area. By understanding fractions, decimals, and the relationship between them and percentages, you can effectively analyze data, make informed decisions, and solve problems in a wide array of contexts. So, keep practicing, and you'll soon be a percentage pro!
Latest Posts
Latest Posts
-
How Many Bones Does Shark Have
Apr 03, 2025
-
Ca Oh 2 Acid Or Base
Apr 03, 2025
-
Quadrilateral With One Pair Of Parallel Sides
Apr 03, 2025
-
Any Change In Velocity Is Called
Apr 03, 2025
-
What Is The Difference Heat And Temperature
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 1 Out Of 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
