What Is The Lcm Of 7 5 And 3
Juapaving
Mar 29, 2025 · 4 min read
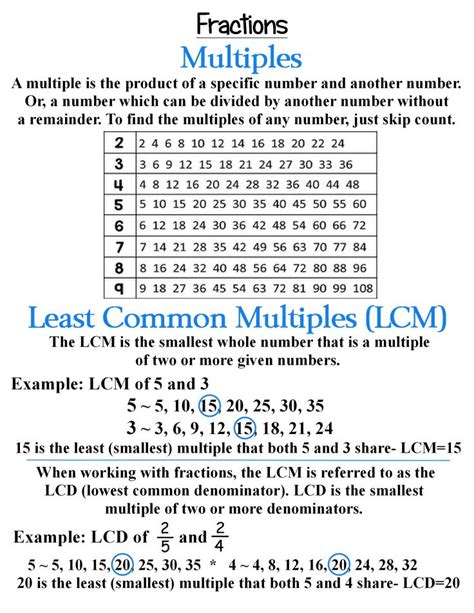
Table of Contents
What is the LCM of 7, 5, and 3? A Deep Dive into Least Common Multiples
Finding the least common multiple (LCM) is a fundamental concept in mathematics, crucial for various applications, from simplifying fractions to solving complex problems in algebra and beyond. This comprehensive guide will not only answer the question "What is the LCM of 7, 5, and 3?" but also delve into the underlying principles and methods for calculating LCMs, providing you with a robust understanding of this essential mathematical tool.
Understanding Least Common Multiples (LCM)
Before we tackle the specific problem of finding the LCM of 7, 5, and 3, let's establish a firm grasp of what LCMs are and why they're important.
The least common multiple of two or more integers is the smallest positive integer that is a multiple of all the given integers. In simpler terms, it's the smallest number that all the given numbers can divide into evenly.
For example, consider the numbers 2 and 3. Multiples of 2 are 2, 4, 6, 8, 10, 12… Multiples of 3 are 3, 6, 9, 12, 15… The common multiples of 2 and 3 are 6, 12, 18, and so on. The least common multiple is 6.
Why is LCM Important?
The LCM finds practical applications in various areas, including:
-
Fraction Addition and Subtraction: To add or subtract fractions with different denominators, you need to find the LCM of the denominators to create a common denominator. This allows for easy addition or subtraction of the numerators.
-
Scheduling Problems: LCM is useful in solving scheduling problems. For instance, if event A happens every 7 days, event B every 5 days, and event C every 3 days, finding the LCM helps determine when all three events will occur simultaneously.
-
Modular Arithmetic: LCM plays a key role in modular arithmetic, a branch of number theory with applications in cryptography and computer science.
-
Rhythm and Music: LCM helps in understanding musical rhythms and time signatures. Finding the LCM of different note durations helps synchronize musical patterns.
Methods for Calculating LCM
There are several effective methods for calculating the LCM of numbers, including:
1. Listing Multiples Method
This method involves listing the multiples of each number until a common multiple is found. It's straightforward but can be time-consuming for larger numbers. Let's illustrate this with our example:
- Multiples of 7: 7, 14, 21, 28, 35, 42, 49, 56, 63, 70, 77, 84, 91, 98, 105...
- Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60, 65, 70, 75, 80, 85, 90, 95, 100, 105...
- Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48, 51, 54, 57, 60, 63, 66, 69, 72, 75, 78, 81, 84, 87, 90, 93, 96, 99, 102, 105...
The smallest common multiple is 105.
2. Prime Factorization Method
This is a more efficient method, especially for larger numbers. It involves finding the prime factorization of each number and then constructing the LCM using the highest powers of all prime factors present.
- Prime factorization of 7: 7 (7 is a prime number)
- Prime factorization of 5: 5 (5 is a prime number)
- Prime factorization of 3: 3 (3 is a prime number)
Since 7, 5, and 3 are all prime numbers and are distinct, the LCM is simply the product of these numbers:
LCM(7, 5, 3) = 7 × 5 × 3 = 105
3. Greatest Common Divisor (GCD) Method
The LCM and GCD (Greatest Common Divisor) are related. The product of the LCM and GCD of two numbers is equal to the product of the two numbers. This relationship can be extended to more than two numbers, though the calculation becomes more complex. However, for our relatively small numbers, this method is not significantly more efficient than prime factorization.
Solving the Problem: LCM of 7, 5, and 3
Using the most efficient method, prime factorization, we've already determined that the least common multiple of 7, 5, and 3 is 105. This is because 7, 5, and 3 are all prime numbers and share no common factors other than 1. Therefore, their LCM is simply their product.
Applications and Further Exploration
Understanding LCM extends beyond simple arithmetic. Here are some areas where this concept proves invaluable:
-
Computer Science: In scheduling tasks within an operating system or managing resource allocation, LCM helps optimize efficiency.
-
Engineering: In designing systems with repetitive cycles or patterns, LCM plays a role in ensuring synchronization and preventing conflicts.
-
Cryptography: The concept of LCM is fundamental in certain cryptographic algorithms.
Conclusion: Mastering LCM for Mathematical Success
This detailed exploration of LCMs, particularly focusing on the LCM of 7, 5, and 3, provides a comprehensive understanding of this crucial mathematical concept. We've covered various methods for calculating LCMs, highlighted their importance in diverse fields, and demonstrated how to apply these methods to solve real-world problems. By mastering the concepts and techniques presented here, you'll be well-equipped to tackle more advanced mathematical challenges and appreciate the significance of LCM in numerous applications. Remember to practice using different methods to solidify your understanding and choose the most efficient method based on the numbers involved. This will not only enhance your mathematical skills but also strengthen your problem-solving abilities across various domains.
Latest Posts
Latest Posts
-
The Pitch Of A Sound Is Determined By What
Apr 01, 2025
-
Is 2 3 Less Than 1 2
Apr 01, 2025
-
Is 23 A Prime Number Or Composite
Apr 01, 2025
-
Is The Square Root Of 45 A Rational Number
Apr 01, 2025
-
Which Phase Do Chromosomes First Become Visible
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Lcm Of 7 5 And 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
