What Is A Least Common Multiple Of 8 And 12
Juapaving
Apr 01, 2025 · 5 min read
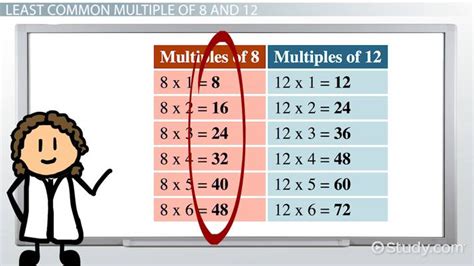
Table of Contents
What is the Least Common Multiple (LCM) of 8 and 12? A Comprehensive Guide
Finding the least common multiple (LCM) might seem like a simple arithmetic task, but understanding the underlying concepts and various methods for calculation is crucial for a strong foundation in mathematics. This comprehensive guide delves into the LCM of 8 and 12, exploring different approaches, practical applications, and its significance in various mathematical contexts. We'll go beyond just finding the answer and delve into the why and how of LCM calculations.
Understanding Least Common Multiple (LCM)
The least common multiple (LCM) of two or more integers is the smallest positive integer that is divisible by all the integers. In simpler terms, it's the smallest number that contains all the numbers as factors. Think of it as the smallest common ground where all the numbers "meet" in terms of divisibility.
For example, let's consider the numbers 2 and 3. The multiples of 2 are 2, 4, 6, 8, 10, 12... and the multiples of 3 are 3, 6, 9, 12, 15... The common multiples are 6, 12, 18... The smallest of these common multiples is 6; therefore, the LCM of 2 and 3 is 6.
Finding the LCM of 8 and 12: Multiple Methods
There are several ways to determine the LCM of 8 and 12. Let's explore the most common and effective methods:
1. Listing Multiples Method
This method is straightforward but can become cumbersome for larger numbers. We list the multiples of each number until we find the smallest common multiple.
- Multiples of 8: 8, 16, 24, 32, 40, 48, 56...
- Multiples of 12: 12, 24, 36, 48, 60...
The smallest number that appears in both lists is 24. Therefore, the LCM of 8 and 12 is 24.
2. Prime Factorization Method
This is a more efficient method, especially for larger numbers. It involves breaking down each number into its prime factors.
- Prime factorization of 8: 2 x 2 x 2 = 2³
- Prime factorization of 12: 2 x 2 x 3 = 2² x 3
To find the LCM, we take the highest power of each prime factor present in the factorizations and multiply them together.
- Highest power of 2: 2³ = 8
- Highest power of 3: 3¹ = 3
LCM (8, 12) = 2³ x 3 = 8 x 3 = 24
3. Greatest Common Divisor (GCD) Method
This method utilizes the relationship between the LCM and the greatest common divisor (GCD) of two numbers. The GCD is the largest number that divides both numbers without leaving a remainder.
- Finding the GCD of 8 and 12: The divisors of 8 are 1, 2, 4, 8. The divisors of 12 are 1, 2, 3, 4, 6, 12. The greatest common divisor is 4.
The LCM and GCD are related by the following formula:
LCM(a, b) x GCD(a, b) = a x b
Substituting the values:
LCM(8, 12) x 4 = 8 x 12
LCM(8, 12) = (8 x 12) / 4 = 96 / 4 = 24
Practical Applications of LCM
The concept of LCM isn't just a theoretical exercise; it has numerous practical applications in various fields:
1. Scheduling and Time Management
Imagine you have two machines that perform a task. One takes 8 minutes, and the other takes 12 minutes. To find out when both machines will finish their tasks simultaneously, you need the LCM. The LCM of 8 and 12 (24 minutes) represents the time when both machines will complete their cycles at the same time.
2. Fractions and Arithmetic Operations
LCM plays a vital role in adding and subtracting fractions with different denominators. To add 1/8 and 1/12, we find the LCM of 8 and 12, which is 24. We then convert the fractions to have a common denominator of 24 before adding them.
3. Measurement and Conversions
When dealing with measurements involving different units, LCM can help in finding a common unit for easier calculations.
4. Music and Rhythm
In music theory, LCM is used to determine the least common multiple of rhythmic durations. It helps in coordinating different musical parts that have different rhythmic patterns.
5. Engineering and Design
In engineering and design, LCM can help to determine optimal cycle times or synchronization points in complex systems with multiple components that operate at different speeds or intervals.
Beyond the Basics: Extending LCM Concepts
The concept of LCM extends beyond just two numbers. You can find the LCM of three or more numbers using similar methods, primarily the prime factorization method. For example, to find the LCM of 8, 12, and 15:
- Prime factorization of 8: 2³
- Prime factorization of 12: 2² x 3
- Prime factorization of 15: 3 x 5
The LCM would be 2³ x 3 x 5 = 120
LCM and Divisibility Rules
Understanding divisibility rules can assist in finding the LCM more efficiently. For instance, knowing that a number is divisible by 8 if its last three digits are divisible by 8, and it's divisible by 12 if it's divisible by both 3 and 4 can help in quickly identifying common multiples.
Conclusion: Mastering LCM for Mathematical Proficiency
The least common multiple is a fundamental concept in mathematics with broad applications across various disciplines. Mastering different methods for calculating the LCM, from listing multiples to using prime factorization and the GCD relationship, is essential for developing a strong mathematical foundation. Understanding the underlying principles and exploring its diverse applications will enhance your problem-solving skills and deepen your appreciation for the interconnectedness of mathematical concepts. The seemingly simple task of finding the LCM of 8 and 12 serves as a gateway to a deeper understanding of number theory and its real-world implications. Remember, practice makes perfect, so continue exploring and applying these concepts to solidify your understanding and build your mathematical proficiency.
Latest Posts
Latest Posts
-
How Are Sedimentary Rocks Formed Short Answer
Apr 02, 2025
-
Difference Between Atom And An Ion
Apr 02, 2025
-
2 Variances F Hypothesis Test Calculator
Apr 02, 2025
-
What Monument In India Is Made Of Metamorphic Rock
Apr 02, 2025
-
65 Inches In Feet And Inches
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is A Least Common Multiple Of 8 And 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
