What Can You Multiply To Get 72
Juapaving
Mar 30, 2025 · 5 min read
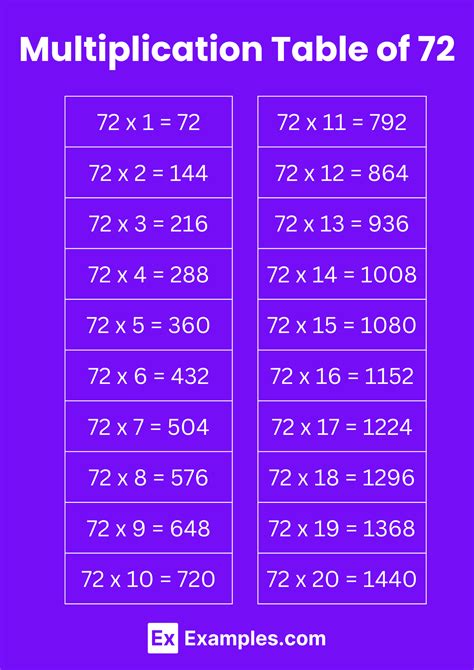
Table of Contents
What Can You Multiply to Get 72? A Deep Dive into Factor Pairs and Number Theory
The seemingly simple question, "What can you multiply to get 72?" opens a fascinating door into the world of mathematics, specifically number theory and factorization. While the immediate answer might seem straightforward, exploring this question fully reveals a rich tapestry of mathematical concepts and applications. This article will delve into finding all the factor pairs of 72, exploring different approaches, and connecting this seemingly basic problem to more advanced mathematical ideas.
Understanding Factors and Factor Pairs
Before diving into the specifics of 72, let's establish a clear understanding of fundamental terms. A factor of a number is a whole number that divides evenly into that number without leaving a remainder. A factor pair is a set of two factors whose product equals the given number. For example, the factor pairs of 12 are (1, 12), (2, 6), (3, 4), and their reverses (12,1), (6,2), (4,3).
Finding the Factor Pairs of 72: A Systematic Approach
There are several ways to find all the factor pairs of 72. Let's explore a few effective methods:
1. The Systematic Listing Method
This method involves systematically listing all possible whole number factors starting from 1 and working upwards. We check if each number divides 72 evenly:
- 1: 72 ÷ 1 = 72 (Factor pair: 1, 72)
- 2: 72 ÷ 2 = 36 (Factor pair: 2, 36)
- 3: 72 ÷ 3 = 24 (Factor pair: 3, 24)
- 4: 72 ÷ 4 = 18 (Factor pair: 4, 18)
- 6: 72 ÷ 6 = 12 (Factor pair: 6, 12)
- 8: 72 ÷ 8 = 9 (Factor pair: 8, 9)
Notice that after 8, we've essentially found all the factor pairs. Any further numbers we test will simply be the reverse of pairs we've already identified.
Therefore, the complete list of factor pairs for 72 is: (1, 72), (2, 36), (3, 24), (4, 18), (6, 12), (8, 9). And their reverses (72,1), (36,2), (24,3), (18,4), (12,6), (9,8).
2. Prime Factorization Method
This method leverages the concept of prime factorization. Prime factorization is the process of expressing a number as a product of its prime factors. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself (e.g., 2, 3, 5, 7, 11...).
The prime factorization of 72 is 2³ x 3². This means 72 = 2 x 2 x 2 x 3 x 3.
Using this prime factorization, we can systematically create factor pairs:
- Using only the prime factors: (2, 36), (3, 24), (4, 18), (6, 12), (8, 9)
- Combining prime factors in different ways: (1, 72) (We can achieve 1 by multiplying no prime factors and 72 by multiplying all prime factors)
This method provides a more structured approach, especially for larger numbers.
Extending the Concept: Beyond Simple Factor Pairs
The question of what numbers multiply to 72 can extend beyond simple integer pairs.
1. Fractions and Decimals: Infinite Possibilities
If we relax the constraint to only whole numbers, the number of possible combinations explodes. For example, 0.5 x 144 = 72, 1.5 x 48 = 72 and countless others using fractions and decimals.
2. Negative Numbers
Including negative numbers expands the possibilities even further. (-1, -72), (-2, -36), (-3, -24) and so on are all valid factor pairs.
3. Complex Numbers
In the realm of complex numbers (numbers involving the imaginary unit 'i', where i² = -1), the possibilities become truly infinite.
Applications of Factorization in Real-World Scenarios
Understanding factors and factorization is not merely an academic exercise; it has numerous practical applications:
- Simplification of Fractions: Finding the greatest common factor (GCF) of the numerator and denominator allows us to simplify fractions to their lowest terms. For example, simplifying 72/144 involves finding the GCF of 72 and 144, which is 72, leading to the simplified fraction 1/2.
- Algebra and Equation Solving: Factoring is crucial for solving quadratic equations and other polynomial equations.
- Cryptography: Prime factorization is at the heart of many modern encryption methods, including RSA cryptography, which secures online transactions and sensitive data.
- Geometry and Measurement: Calculating areas, volumes, and other geometric properties often involves working with factors and multiples.
- Computer Science and Algorithms: Efficient algorithms for finding prime factors and performing other factorization tasks are essential in various computer science applications.
Further Exploration: Divisibility Rules and Number Properties
Understanding divisibility rules can further aid in quickly identifying factors. For example:
- Divisibility by 2: A number is divisible by 2 if its last digit is even.
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3. (7 + 2 = 9, which is divisible by 3, so 72 is divisible by 3).
- Divisibility by 4: A number is divisible by 4 if its last two digits are divisible by 4.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 6: A number is divisible by 6 if it is divisible by both 2 and 3.
- Divisibility by 8: A number is divisible by 8 if its last three digits are divisible by 8.
- Divisibility by 9: A number is divisible by 9 if the sum of its digits is divisible by 9.
Mastering these divisibility rules can significantly speed up the process of finding factors.
Conclusion: The Richness of a Simple Question
The seemingly simple question, "What can you multiply to get 72?" has led us on a journey through fundamental mathematical concepts, highlighting the importance of factor pairs, prime factorization, and their wide-ranging applications. From simplifying fractions to securing online transactions, the ability to efficiently find factors underpins many crucial aspects of mathematics and computer science. This exploration demonstrates the beauty and power of mathematics, even within a seemingly simple problem. The seemingly straightforward question unveils a wealth of mathematical concepts, showcasing the interconnectedness and depth within even the most basic arithmetic. Understanding these principles can significantly enhance your mathematical skills and open up a world of further exploration.
Latest Posts
Latest Posts
-
What Is The Prime Factorization For 23
Apr 01, 2025
-
Greatest Common Factor For 36 And 90
Apr 01, 2025
-
Find The Least Common Multiple Of 5 And 9
Apr 01, 2025
-
Pxidation Number Of H In H20
Apr 01, 2025
-
Sweating Is An Example Of Homeostasis
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Can You Multiply To Get 72 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
