What Are The Factors Of 102
Juapaving
Mar 28, 2025 · 6 min read
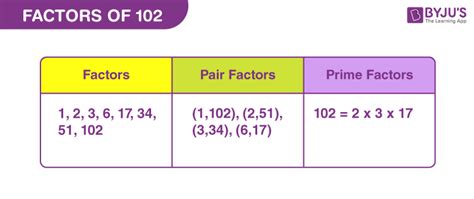
Table of Contents
What are the Factors of 102? A Deep Dive into Prime Factorization and Divisibility
Finding the factors of a number might seem like a simple arithmetic task, but it's a fundamental concept in mathematics with wide-ranging applications in number theory, algebra, and even cryptography. This article will delve deep into determining the factors of 102, exploring the methods used, and connecting it to broader mathematical principles. We'll move beyond simply listing the factors to understand why these numbers are factors and how to find them efficiently for any number.
Understanding Factors and Divisibility
Before we tackle the factors of 102 specifically, let's define some key terms:
-
Factor (or Divisor): A factor of a number is a whole number that divides evenly into that number without leaving a remainder. For example, 2 is a factor of 10 because 10 ÷ 2 = 5.
-
Divisibility: Divisibility refers to the ability of one number to be divided evenly by another number. If a number is divisible by another, the remainder is zero.
-
Prime Number: A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. Examples include 2, 3, 5, 7, 11, and so on.
-
Composite Number: A composite number is a whole number greater than 1 that is not a prime number; it has more than two divisors.
Finding the Factors of 102: A Step-by-Step Approach
There are several ways to find the factors of 102. Let's explore a few:
Method 1: Systematic Listing
The most straightforward method is to systematically test each whole number, starting from 1, to see if it divides 102 without leaving a remainder.
- 1: 102 ÷ 1 = 102 (1 is always a factor)
- 2: 102 ÷ 2 = 51 (2 is a factor)
- 3: 102 ÷ 3 = 34 (3 is a factor)
- 4: 102 ÷ 4 = 25.5 (4 is not a factor)
- 5: 102 ÷ 5 = 20.4 (5 is not a factor)
- 6: 102 ÷ 6 = 17 (6 is a factor)
- 7: 102 ÷ 7 ≈ 14.57 (7 is not a factor)
- 8: 102 ÷ 8 = 12.75 (8 is not a factor)
- 9: 102 ÷ 9 ≈ 11.33 (9 is not a factor)
- 10: 102 ÷ 10 = 10.2 (10 is not a factor)
- 12: 102 ÷ 12 = 8.5 (12 is not a factor)
- 17: 102 ÷ 17 = 6 (17 is a factor)
- 34: 102 ÷ 34 = 3 (34 is a factor)
- 51: 102 ÷ 51 = 2 (51 is a factor)
- 102: 102 ÷ 102 = 1 (102 is always a factor)
Therefore, the factors of 102 are 1, 2, 3, 6, 17, 34, 51, and 102.
Method 2: Prime Factorization
Prime factorization is a more efficient method, particularly for larger numbers. It involves breaking down a number into its prime factors – the prime numbers that multiply together to give the original number.
-
Start with the smallest prime number, 2: 102 is an even number, so it's divisible by 2. 102 ÷ 2 = 51.
-
Consider the next prime number, 3: 51 is divisible by 3. 51 ÷ 3 = 17.
-
17 is a prime number: We've reached a prime number, so the factorization is complete.
Therefore, the prime factorization of 102 is 2 x 3 x 17.
Method 3: Using the Factor Pairs
Once you have the prime factorization, you can easily find all the factors. You can create factor pairs by combining the prime factors in different ways:
- 1 x 102
- 2 x 51
- 3 x 34
- 6 x 17
This method quickly generates all the factors: 1, 2, 3, 6, 17, 34, 51, 102.
Connecting Factors to Divisibility Rules
Understanding divisibility rules can speed up the process of finding factors. Here are some helpful rules:
-
Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, or 8). 102 is divisible by 2 because its last digit is 2.
-
Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3. 1 + 0 + 2 = 3, which is divisible by 3, so 102 is divisible by 3.
-
Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5. 102 is not divisible by 5.
-
Divisibility by 6: A number is divisible by 6 if it's divisible by both 2 and 3. Since 102 is divisible by both 2 and 3, it's divisible by 6.
-
Divisibility by 9: A number is divisible by 9 if the sum of its digits is divisible by 9. The sum of digits in 102 is 3, which is not divisible by 9, so 102 is not divisible by 9.
Applications of Finding Factors
Finding factors isn't just an academic exercise; it has practical applications in various fields:
-
Algebra: Factoring is crucial for solving algebraic equations and simplifying expressions.
-
Number Theory: Factors play a vital role in understanding concepts like greatest common divisors (GCD), least common multiples (LCM), and modular arithmetic.
-
Cryptography: Prime factorization is fundamental to many modern encryption methods, such as RSA encryption, which relies on the difficulty of factoring very large numbers.
-
Computer Science: Efficient algorithms for finding factors are essential in various computational tasks.
Beyond the Basics: Exploring Related Concepts
Understanding the factors of 102 provides a foundation for exploring more advanced concepts:
Greatest Common Divisor (GCD)
The greatest common divisor (GCD) of two or more numbers is the largest number that divides all of them without leaving a remainder. For example, to find the GCD of 102 and another number, say 136, we'd find the factors of both and identify the largest common factor.
Least Common Multiple (LCM)
The least common multiple (LCM) of two or more numbers is the smallest number that is a multiple of all of them. Finding the LCM often involves using the prime factorization of the numbers involved.
Perfect Numbers and Abundant/Deficient Numbers
A perfect number is a positive integer that is equal to the sum of its proper divisors (excluding itself). An abundant number has a sum of its proper divisors greater than itself, while a deficient number has a sum of its proper divisors less than itself. The factors of a number are essential in determining whether it falls into any of these categories.
Conclusion: The Significance of Factorization
This in-depth exploration of the factors of 102 has demonstrated that finding factors is more than just a simple arithmetic calculation. It's a foundational concept with significant implications across various mathematical fields and practical applications. By understanding different methods, divisibility rules, and related concepts like GCD and LCM, we can appreciate the power and importance of factorization in mathematics and beyond. The seemingly simple question, "What are the factors of 102?" has opened a door to a rich and fascinating world of number theory.
Latest Posts
Latest Posts
-
Least Common Multiple Of 8 And 36
Mar 31, 2025
-
Which Of The Following Are Found In Prokaryotic Cells
Mar 31, 2025
-
Is The Square Root Of 36 Rational
Mar 31, 2025
-
Is Condensation A Chemical Or Physical Change
Mar 31, 2025
-
Is Calcium A Metal Nonmetal Or Metalloid
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Are The Factors Of 102 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
