What Are The Common Factors Of 56
Juapaving
Apr 01, 2025 · 6 min read
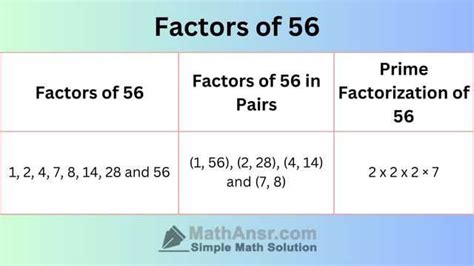
Table of Contents
What are the Common Factors of 56? A Deep Dive into Number Theory
Finding the factors of a number might seem like a simple arithmetic task, but it opens the door to understanding fundamental concepts in number theory. This article explores the common factors of 56, delving into the methods for finding them, their significance in mathematics, and how this seemingly basic concept connects to more complex mathematical ideas.
Understanding Factors and Divisibility
Before we dive into the specifics of 56, let's define some key terms:
-
Factor (or Divisor): A factor of a number is a whole number that divides the number exactly without leaving a remainder. For example, 2 is a factor of 6 because 6 divided by 2 equals 3 with no remainder.
-
Divisibility: Divisibility refers to the property of one number being completely divisible by another. If a number a is divisible by a number b, then a/b results in a whole number.
-
Common Factor: A common factor of two or more numbers is a number that divides each of them without leaving a remainder. For instance, 3 is a common factor of both 6 and 9.
-
Greatest Common Factor (GCF) or Highest Common Factor (HCF): The greatest common factor is the largest number that divides each of a set of numbers without leaving a remainder.
Finding the Factors of 56
To find the common factors of 56, we first need to determine all the factors of 56 itself. We can do this systematically:
-
Start with 1: 1 is a factor of every number.
-
Check for 2: 56 is an even number, so it's divisible by 2 (56/2 = 28).
-
Check for 3: The sum of the digits of 56 (5 + 6 = 11) is not divisible by 3, so 56 is not divisible by 3.
-
Check for 4: 56 is divisible by 4 (56/4 = 14).
-
Check for 5: 56 does not end in 0 or 5, so it's not divisible by 5.
-
Check for 6: Since 56 is divisible by 2 but not 3, it's not divisible by 6.
-
Check for 7: 56 is divisible by 7 (56/7 = 8).
-
Check for 8: 56 is divisible by 8 (56/8 = 7).
-
Continue until the square root: We continue checking factors until we reach the square root of 56 (approximately 7.48). Once we find a factor beyond this point, its corresponding pair will already have been found.
Therefore, the factors of 56 are 1, 2, 4, 7, 8, 14, 28, and 56.
Finding Common Factors
To find the common factors of 56 with another number, let's say we want to find the common factors of 56 and 42. We first find the factors of 42:
The factors of 42 are 1, 2, 3, 6, 7, 14, 21, and 42.
Now, we compare the factor lists of 56 and 42:
- 1 is a common factor.
- 2 is a common factor.
- 7 is a common factor.
- 14 is a common factor.
Therefore, the common factors of 56 and 42 are 1, 2, 7, and 14. The greatest common factor (GCF) of 56 and 42 is 14.
Prime Factorization and the GCF
Prime factorization provides a more efficient way to find the greatest common factor (GCF) of two or more numbers. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself.
The prime factorization of 56 is 2 x 2 x 2 x 7 (or 2³ x 7).
Let's use this method to find the GCF of 56 and 42. The prime factorization of 42 is 2 x 3 x 7.
To find the GCF, we identify the common prime factors and their lowest powers:
- Both 56 and 42 have a factor of 2 (to the power of 1)
- Both 56 and 42 have a factor of 7 (to the power of 1)
Therefore, the GCF is 2 x 7 = 14. This method is particularly useful when dealing with larger numbers.
Applications of Finding Factors
The seemingly simple process of finding factors has numerous applications in various fields:
-
Algebra: Factoring polynomials relies heavily on understanding the factors of numbers. This is crucial for solving equations and simplifying expressions.
-
Cryptography: Prime factorization is a cornerstone of modern cryptography. The difficulty of factoring large numbers into their prime components forms the basis of many encryption algorithms.
-
Computer Science: Algorithms for finding prime factors are used in various computer science applications, including data compression and security protocols.
-
Music Theory: Musical intervals and harmonies are related to mathematical ratios, and understanding factors helps in analyzing and composing music.
Beyond the Basics: Exploring Number Theory Concepts Related to Factors
The concept of factors extends into deeper mathematical concepts:
-
Perfect Numbers: A perfect number is a positive integer that is equal to the sum of its proper divisors (excluding the number itself). 6 is a perfect number (1 + 2 + 3 = 6). The search for perfect numbers is an ongoing area of mathematical research.
-
Abundant Numbers: An abundant number is a number where the sum of its proper divisors is greater than the number itself. For example, 12 is abundant (1 + 2 + 3 + 4 + 6 = 16 > 12).
-
Deficient Numbers: A deficient number is a number where the sum of its proper divisors is less than the number itself. Most numbers are deficient.
-
Modular Arithmetic: Modular arithmetic is a system of arithmetic for integers, where numbers "wrap around" upon reaching a certain value (the modulus). Understanding divisibility and factors is crucial for working with modular arithmetic.
-
Euclidean Algorithm: The Euclidean algorithm is an efficient method for computing the greatest common divisor (GCD) of two integers. It's based on the principle of repeated division.
-
Diophantine Equations: Diophantine equations are polynomial equations where only integer solutions are sought. Finding factors and GCDs plays a crucial role in solving certain types of Diophantine equations.
Conclusion: The Significance of Simple Arithmetic
While finding the factors of 56 might seem like a simple exercise, it represents a fundamental concept in number theory with far-reaching implications. Understanding factors, prime factorization, and the related concepts we've explored opens doors to a deeper appreciation of mathematical structures and their applications in various fields. From cryptography to music theory, the seemingly simple act of finding factors underpins complex and fascinating mathematical ideas. The journey from understanding the basic factors of 56 to grasping the sophisticated applications of number theory highlights the power and beauty of mathematical exploration.
Latest Posts
Latest Posts
-
Is Boiling A Physical Or Chemical Change
Apr 02, 2025
-
Rectangle Has How Many Lines Of Symmetry
Apr 02, 2025
-
How To Find Recoil In Physics
Apr 02, 2025
-
87 Inches Is How Many Feet
Apr 02, 2025
-
Electrons Are Lost Or Gained During
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Are The Common Factors Of 56 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
