How To Find Recoil In Physics
Juapaving
Apr 02, 2025 · 5 min read
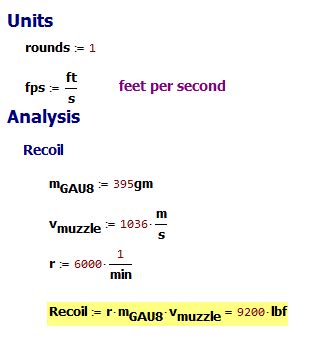
Table of Contents
How to Find Recoil in Physics: A Comprehensive Guide
Understanding recoil in physics is crucial for numerous applications, from designing firearms to analyzing collisions between subatomic particles. This comprehensive guide will delve into the fundamental principles governing recoil, exploring various methods for calculating its magnitude and direction, and highlighting practical examples to solidify your understanding.
Understanding the Fundamentals of Recoil
Recoil, also known as recoil velocity or kick, is the backward momentum experienced by a system after it expels or releases something forward. This phenomenon is a direct consequence of Newton's Third Law of Motion: for every action, there's an equal and opposite reaction. When a system expels mass in one direction, it experiences an equal and opposite momentum in the opposite direction. This is the essence of recoil.
Key Concepts to Grasp:
-
Momentum: Momentum (p) is a vector quantity representing the mass (m) of an object multiplied by its velocity (v): p = mv. It's crucial to understand that momentum is conserved in a closed system, meaning the total momentum before an event equals the total momentum afterward.
-
Conservation of Momentum: This principle forms the cornerstone of recoil calculations. In a system where no external forces act, the total momentum remains constant before and after an event like firing a projectile or a collision.
-
Center of Mass: Understanding the center of mass is vital, particularly for more complex systems. The center of mass acts as a single point where the entire mass of the system is concentrated. Recoil is often calculated relative to the center of mass.
Calculating Recoil: Methods and Applications
The precise method for calculating recoil depends on the specifics of the system involved. However, the fundamental principle of conservation of momentum remains central.
1. Recoil of a Gun: A Simple Example
Consider the classic example of firing a bullet from a gun. We'll make some simplifying assumptions:
- No external forces: We ignore air resistance and friction.
- Gun and bullet as a system: The gun and the bullet constitute a closed system before firing.
- Initial momentum is zero: Before firing, the system is at rest.
Using the conservation of momentum:
- Initial momentum (before firing) = 0
- Final momentum (after firing) = Momentum of bullet + Momentum of gun
Therefore:
0 = m<sub>bullet</sub> * v<sub>bullet</sub> + m<sub>gun</sub> * v<sub>gun</sub>
Where:
- m<sub>bullet</sub> = mass of the bullet
- v<sub>bullet</sub> = velocity of the bullet
- m<sub>gun</sub> = mass of the gun
- v<sub>gun</sub> = recoil velocity of the gun (what we want to find)
Solving for v<sub>gun</sub>:
v<sub>gun</sub> = - (m<sub>bullet</sub> * v<sub>bullet</sub>) / m<sub>gun</sub>
The negative sign indicates that the recoil velocity is in the opposite direction to the bullet's velocity.
2. Recoil in Rocket Propulsion
Rocket propulsion offers a more complex but fascinating illustration of recoil. Instead of a single bullet, a rocket continuously expels propellant, leading to a continuous recoil force. The calculation involves considering the rate of propellant expulsion (mass flow rate) and the velocity of the expelled propellant.
The thrust (force) produced by a rocket is given by:
F = ṁ * v<sub>e</sub>
Where:
- F = thrust force
- ṁ = mass flow rate of the propellant
- v<sub>e</sub> = exhaust velocity of the propellant
This thrust force generates the recoil, propelling the rocket forward. The analysis becomes more involved when considering the changing mass of the rocket as it burns fuel.
3. Recoil in Collisions: Elastic and Inelastic
Recoil isn't limited to firearms and rockets; it's fundamental to all types of collisions. The calculation method differs slightly depending on whether the collision is elastic (kinetic energy is conserved) or inelastic (kinetic energy is not conserved).
-
Elastic Collisions: In elastic collisions, both momentum and kinetic energy are conserved. This leads to a system of equations that can be solved to find the recoil velocities of the colliding objects.
-
Inelastic Collisions: In inelastic collisions, some kinetic energy is lost, usually transformed into heat or sound. Momentum is still conserved, but the calculations are simpler as we only need to consider momentum conservation.
4. Advanced Recoil Calculations: Multi-body Systems and External Forces
For more complex scenarios involving multiple objects or external forces (like air resistance or friction), the calculations become more intricate. Techniques such as vector analysis and numerical methods (e.g., finite element analysis) are often employed. These advanced methods often involve utilizing software tools to solve the equations.
Factors Influencing Recoil
Several factors influence the magnitude of recoil experienced:
- Mass of the projectile: A heavier projectile will produce a greater recoil.
- Velocity of the projectile: A higher projectile velocity leads to a greater recoil.
- Mass of the system: A heavier system (like a heavier gun) will experience less recoil for the same projectile.
- Angle of projection: The angle at which the projectile is launched affects the recoil vector's direction.
- Friction and air resistance: These factors can influence the magnitude and direction of the recoil, though often negligible in many practical situations.
Practical Examples and Real-World Applications
Recoil principles aren't confined to theoretical physics; they have numerous practical applications:
-
Firearms design: Understanding recoil is paramount in designing firearms that are safe and effective to use. Features like recoil reduction systems are directly based on recoil principles.
-
Rocket science: The design and optimization of rocket engines rely heavily on mastering recoil calculations to achieve desired trajectory and performance.
-
Nuclear physics: The recoil of atomic nuclei after radioactive decay or nuclear reactions is crucial for understanding these processes.
-
Sports: Many sports, from shooting to archery, involve recoil management for accuracy and performance enhancement.
-
Collision safety: Understanding recoil is vital for designing safety systems in vehicles and other applications to mitigate the impact of collisions.
Conclusion: Mastering Recoil Calculations
Mastering recoil calculations is a multifaceted journey that takes you through the core principles of Newtonian mechanics, particularly the conservation of momentum. While simple scenarios can be solved with relatively straightforward equations, more complex systems often necessitate advanced computational techniques. However, the underlying principle—the equality of action and reaction—remains the foundation of all recoil phenomena. This understanding has far-reaching implications in various scientific and engineering disciplines, from designing safer firearms to propelling rockets into space. The more deeply you delve into the subject, the more you'll appreciate the elegance and power of this fundamental physical principle.
Latest Posts
Latest Posts
-
Biotic Factors In An Aquatic Ecosystem
Apr 03, 2025
-
Least Common Multiple Of 10 And 8
Apr 03, 2025
-
Four Ways To Write A Number
Apr 03, 2025
-
How To Calculate The Perimeter Of A Rhombus
Apr 03, 2025
-
Is The Electromagnetic Spectrum Transverse Or Longitudinal
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How To Find Recoil In Physics . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
