Volume Of A Cylinder In Cubic Feet
Juapaving
Apr 02, 2025 · 5 min read
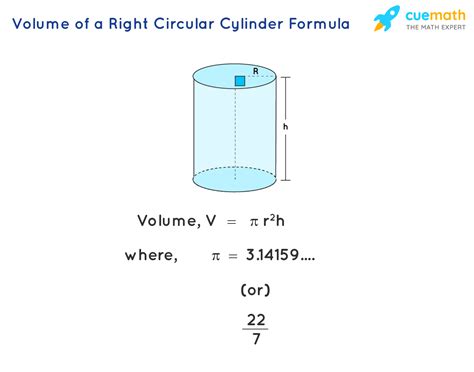
Table of Contents
Calculating the Volume of a Cylinder in Cubic Feet: A Comprehensive Guide
Understanding how to calculate the volume of a cylinder, particularly in cubic feet, is crucial in various fields, from engineering and construction to agriculture and storage. Whether you're designing a water tank, estimating the capacity of a silo, or simply tackling a geometry problem, mastering this calculation is essential. This comprehensive guide will walk you through the process, offering practical examples and tips to ensure you confidently calculate the volume of any cylindrical object in cubic feet.
Understanding the Formula: πr²h
The foundation of calculating a cylinder's volume lies in its formula: V = πr²h. Let's break down each component:
- V: Represents the volume of the cylinder, expressed in cubic units (in our case, cubic feet).
- π (pi): A mathematical constant, approximately equal to 3.14159. It represents the ratio of a circle's circumference to its diameter. For most calculations, using 3.14 is sufficiently accurate, but for greater precision, use your calculator's π function.
- r: Represents the radius of the cylinder's circular base. The radius is half the diameter (diameter/2). Ensure you measure the radius in feet to obtain the volume in cubic feet.
- h: Represents the height of the cylinder. Similar to the radius, measure the height in feet for a volume expressed in cubic feet.
Step-by-Step Calculation Guide
To effectively calculate the volume of a cylinder in cubic feet, follow these steps:
-
Measure the radius (r): Use a measuring tape or ruler to find the radius of the cylinder's circular base. Remember to measure in feet. If your measurement is in inches, convert it to feet by dividing by 12 (e.g., 12 inches = 1 foot). Be precise with your measurement, as even small errors can significantly impact the final volume.
-
Measure the height (h): Measure the height of the cylinder from the base to the top, again ensuring your measurement is in feet. Consistent units are paramount for accurate results.
-
Square the radius (r²): Multiply the radius by itself (r * r).
-
Multiply by π: Multiply the squared radius (r²) by π (approximately 3.14 or use your calculator's π function for better accuracy).
-
Multiply by the height (h): Multiply the result from step 4 by the height (h) of the cylinder.
-
Express the result: The final result represents the volume of the cylinder in cubic feet.
Practical Examples
Let's solidify our understanding with some practical examples:
Example 1: A simple cylindrical tank
Imagine a cylindrical water tank with a radius of 2 feet and a height of 5 feet. Let's calculate its volume:
- Radius (r) = 2 feet
- Height (h) = 5 feet
- r² = 2 * 2 = 4 square feet
- πr² = 3.14 * 4 = 12.56 square feet
- V = 12.56 * 5 = 62.8 cubic feet
Therefore, the volume of the water tank is approximately 62.8 cubic feet.
Example 2: A more complex scenario
Consider a cylindrical silo with a diameter of 10 feet and a height of 12 feet. Note that we're given the diameter, not the radius.
- Diameter = 10 feet
- Radius (r) = 10 feet / 2 = 5 feet
- Height (h) = 12 feet
- r² = 5 * 5 = 25 square feet
- πr² = 3.14 * 25 = 78.5 square feet
- V = 78.5 * 12 = 942 cubic feet
The volume of the silo is approximately 942 cubic feet.
Example 3: Dealing with inches
Let's say you have a cylindrical container with a radius of 6 inches and a height of 18 inches. First, convert inches to feet:
- Radius (r) = 6 inches / 12 inches/foot = 0.5 feet
- Height (h) = 18 inches / 12 inches/foot = 1.5 feet
- r² = 0.5 * 0.5 = 0.25 square feet
- πr² = 3.14 * 0.25 = 0.785 square feet
- V = 0.785 * 1.5 = 1.1775 cubic feet
The volume of the container is approximately 1.18 cubic feet.
Troubleshooting Common Mistakes
Several common errors can lead to inaccurate volume calculations. Here's how to avoid them:
-
Incorrect unit conversions: Always ensure all measurements are in feet before applying the formula. Consistent units are crucial.
-
Confusing radius and diameter: Remember that the radius is half the diameter. Using the diameter directly in the formula will yield an incorrect result.
-
Rounding errors: While rounding for simplicity is acceptable, excessive rounding early in the calculation can accumulate significant errors. It's best to round only at the final step.
-
Using an inaccurate value for π: For most applications, 3.14 is sufficient. However, using your calculator's π function provides greater precision.
Advanced Applications and Considerations
Understanding cylinder volume extends beyond basic calculations. Here are some advanced applications and considerations:
-
Partial cylinders: If you're dealing with a portion of a cylinder (e.g., a segment of a pipe), you'll need to adjust the calculation accordingly. This might involve using calculus or geometric principles to determine the volume of the partial segment.
-
Cylinders with varying radii: If the radius isn't constant throughout the cylinder's height, the calculation becomes more complex and might require integral calculus.
-
Real-world applications: Accurate volume calculations are critical in various fields. For example, in construction, it's crucial for estimating material quantities (concrete, water, etc.). In agriculture, it aids in determining storage capacity for grains and other products.
Conclusion
Calculating the volume of a cylinder in cubic feet is a fundamental skill with wide-ranging applications. By following the step-by-step guide, understanding the formula, and avoiding common errors, you can confidently determine the volume of cylindrical objects, enabling accurate estimations and informed decision-making in various contexts. Remember the formula: V = πr²h, and always prioritize accurate measurements and consistent units. Mastering this calculation will empower you to tackle numerous practical and theoretical problems involving cylinders.
Latest Posts
Latest Posts
-
Good Words To Describe Your Mother
Apr 03, 2025
-
How Many Bones Does Shark Have
Apr 03, 2025
-
Ca Oh 2 Acid Or Base
Apr 03, 2025
-
Quadrilateral With One Pair Of Parallel Sides
Apr 03, 2025
-
Any Change In Velocity Is Called
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Volume Of A Cylinder In Cubic Feet . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
