State Newton's Law Of Universal Gravitation In Words
Juapaving
Mar 28, 2025 · 6 min read
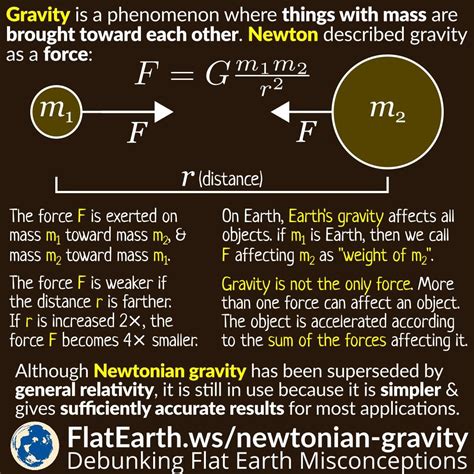
Table of Contents
Newton's Law of Universal Gravitation: A Deep Dive
Newton's Law of Universal Gravitation is a cornerstone of classical mechanics, explaining the attractive force between any two objects with mass. Understanding this law is crucial for grasping celestial mechanics, projectile motion, and even the behavior of everyday objects. While seemingly simple at first glance, the implications of this law are vast and far-reaching. This article will explore Newton's Law of Universal Gravitation in detail, explaining its statement, its mathematical formulation, its applications, and its limitations.
Stating Newton's Law of Universal Gravitation in Words
In simple terms, Newton's Law of Universal Gravitation states that every particle attracts every other particle in the universe with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. Let's break this down:
-
Every particle attracts every other particle: This means that gravity is a universal force affecting all objects with mass, regardless of their size or composition. A tiny speck of dust exerts a gravitational pull on the Earth, and the Earth exerts a gravitational pull back on the speck of dust. The force is always present, even if it's incredibly weak in many everyday scenarios.
-
Directly proportional to the product of their masses: The more massive the objects, the stronger the gravitational attraction between them. Double the mass of one object, and the gravitational force doubles. Double the mass of both objects, and the force quadruples. This explains why the Earth exerts a much stronger gravitational pull on us than, say, a nearby car.
-
Inversely proportional to the square of the distance between their centers: This is the "inverse square law" part. As the distance between the centers of the two objects increases, the gravitational force decreases rapidly. If you double the distance, the force becomes four times weaker (2² = 4). Triple the distance, and the force becomes nine times weaker (3² = 9). This explains why the gravitational pull of the Sun is much stronger on the Earth than it is on distant comets.
The Mathematical Formulation of the Law
The verbal description of Newton's Law is elegantly summarized by a simple mathematical equation:
F = G * (m1 * m2) / r²
Where:
- F represents the gravitational force between the two objects.
- G is the gravitational constant, a fundamental constant in physics that determines the strength of gravitational interaction. Its value is approximately 6.674 x 10⁻¹¹ N⋅m²/kg².
- m1 and m2 represent the masses of the two objects.
- r represents the distance between the centers of the two objects.
This equation quantifies the relationship described above. It allows us to calculate the precise magnitude of the gravitational force between any two objects, given their masses and the distance separating them. The units of force (F) are Newtons (N).
Applications of Newton's Law of Universal Gravitation
Newton's Law of Universal Gravitation has had profound implications across various fields of science and engineering. Some key applications include:
1. Celestial Mechanics:
This is perhaps the most significant application. Newton's Law allows us to:
-
Predict the orbits of planets and other celestial bodies: By applying the law to the Sun and the planets, we can accurately predict their orbital paths, periods, and velocities. This understanding formed the basis for modern astronomy and our comprehension of the solar system.
-
Explain Kepler's Laws of Planetary Motion: Kepler's empirical laws, derived from observational data, are direct consequences of Newton's Law of Universal Gravitation. Newton's law provides the theoretical framework to explain why Kepler's laws work.
-
Understand the dynamics of binary star systems, galaxies, and other large-scale structures: The gravitational interactions between stars, galaxies, and other celestial objects are governed by Newton's law, allowing astronomers to model their behavior and evolution.
2. Terrestrial Applications:
While often overlooked in everyday life, gravity's effects are constantly present:
-
Determining the weight of objects: The weight of an object is simply the gravitational force exerted on it by the Earth. This allows us to understand why objects fall to the ground.
-
Designing structures and buildings: Engineers must account for the effects of gravity when designing bridges, buildings, and other structures. Understanding gravitational forces is critical to ensuring stability and safety.
-
Predicting the trajectory of projectiles: The path of a projectile, such as a ball or a rocket, is influenced by gravity. Newton's Law allows for accurate predictions of projectile motion.
3. Navigation Systems:
- GPS Technology: The accuracy of Global Positioning Systems (GPS) relies heavily on precise calculations involving the gravitational forces exerted by the Earth and its satellites.
Limitations of Newton's Law of Universal Gravitation
Despite its immense success, Newton's Law of Universal Gravitation has its limitations:
1. Doesn't account for extremely strong gravitational fields:
Newton's law breaks down in situations with extremely strong gravitational fields, such as those found near black holes or neutron stars. In these extreme environments, Einstein's theory of General Relativity provides a more accurate description of gravity. General Relativity treats gravity not as a force, but as a curvature of spacetime caused by mass and energy.
2. Doesn't explain the behavior of light:
Newton's Law doesn't explain the effect of gravity on light. According to General Relativity, light bends as it passes through a gravitational field. This effect has been experimentally verified, further highlighting the limitations of Newton's classical description.
3. Doesn't explain the phenomenon of gravitational waves:
Gravitational waves, ripples in spacetime caused by accelerating massive objects, were predicted by Einstein's theory and have been subsequently detected. Newton's Law does not predict or account for the existence of gravitational waves.
Newton's Law and the Concept of Mass
Newton's Law utilizes the concept of mass in two distinct ways:
-
Gravitational Mass: This refers to the mass of an object as it participates in gravitational interactions. It determines the strength of the gravitational force exerted by or on an object.
-
Inertial Mass: This refers to the object's resistance to acceleration. It's a measure of an object's inertia. A more massive object requires a larger force to achieve the same acceleration as a less massive object.
Remarkably, experiments have shown that gravitational mass and inertial mass are equivalent. This equivalence is a cornerstone of Einstein's theory of General Relativity.
Conclusion
Newton's Law of Universal Gravitation, despite its limitations in extreme conditions, remains a remarkably accurate and useful model for understanding gravitational interactions in a wide range of scenarios. Its elegant simplicity and powerful predictive capabilities have revolutionized our understanding of the universe, from the motion of planets to the design of everyday structures. While General Relativity offers a more complete description of gravity, Newton's Law serves as a fundamental stepping stone in our journey to unravel the mysteries of the cosmos. Its enduring legacy lies in its ability to explain the everyday pull of gravity while providing a foundation for more sophisticated gravitational theories. Understanding its principles is essential for anyone seeking a deeper understanding of the physical world.
Latest Posts
Latest Posts
-
Organelles That Are The Sites Of Protein Synthesis
Mar 31, 2025
-
What Is The Percentage Of 2 5
Mar 31, 2025
-
How To Find Reciprocal Of A Mixed Number
Mar 31, 2025
-
Which Of The Following Is Considered A Micronutrient
Mar 31, 2025
-
How Many Inches Is 2 Meters
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about State Newton's Law Of Universal Gravitation In Words . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
