Negative 16 X Greater Or Equal Than Negative 48
Juapaving
Apr 06, 2025 · 5 min read
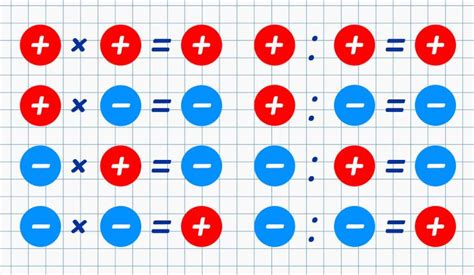
Table of Contents
Decoding the Inequality: -16x ≥ -48
Understanding inequalities is crucial for success in algebra and beyond. This comprehensive guide dives deep into solving the inequality "-16x ≥ -48," explaining the process step-by-step, exploring related concepts, and offering practical applications. We'll cover everything from the fundamental principles to advanced techniques, ensuring you master this concept thoroughly.
Understanding Inequalities
Before tackling our specific problem, let's refresh our understanding of inequalities. Unlike equations, which declare equality between two expressions, inequalities express a relationship of "greater than," "less than," "greater than or equal to," or "less than or equal to." These relationships are represented by the symbols >, <, ≥, and ≤, respectively.
Key Differences between Equations and Inequalities:
- Solution Set: Equations typically have one or a few specific solutions. Inequalities, on the other hand, often have an infinite number of solutions, represented as a range or interval.
- Graphing: Solutions to equations are usually plotted as single points on a number line. Inequality solutions are graphed as intervals or rays, representing the range of values that satisfy the inequality.
- Operations: Most algebraic operations are similar for equations and inequalities. However, multiplying or dividing by a negative number requires flipping the inequality sign. This is a crucial point to remember.
Solving -16x ≥ -48: A Step-by-Step Approach
Now, let's tackle the inequality at hand: -16x ≥ -48. Our goal is to isolate 'x' on one side of the inequality sign.
Step 1: Isolate the term with 'x'
The term with 'x' is already on the left side of the inequality. We need to remove the coefficient -16.
Step 2: Divide both sides by -16
To isolate 'x', we divide both sides of the inequality by -16:
(-16x) / -16 ≥ (-48) / -16
Step 3: Remember the crucial rule!
Because we divided both sides by a negative number (-16), we must reverse the inequality sign. This is the most common mistake made when solving inequalities. Failing to reverse the sign will lead to an incorrect solution.
Therefore, our inequality becomes:
x ≤ 3
Step 4: Verify the solution
Let's verify our solution by testing a few values.
- x = 3: -16(3) = -48. This satisfies the inequality -16x ≥ -48.
- x = 2: -16(2) = -32. Since -32 > -48, this also satisfies the inequality.
- x = 4: -16(4) = -64. This does not satisfy the inequality because -64 is not greater than or equal to -48.
These tests confirm that our solution, x ≤ 3, is correct.
Graphing the Solution
The solution x ≤ 3 can be easily represented on a number line. We would draw a closed circle (or a filled-in dot) at 3, indicating that 3 is included in the solution set, and then shade the number line to the left of 3, representing all values less than or equal to 3.
[Insert image of a number line with a closed circle at 3 and shading to the left.]
Interval Notation
Another way to represent the solution set is using interval notation. In this case, the solution is represented as:
(-∞, 3]
The parenthesis "(" indicates that negative infinity is not included (as infinity is not a number), and the bracket "]" indicates that 3 is included in the interval.
Real-World Applications of Inequalities
Inequalities are not just abstract mathematical concepts; they have numerous real-world applications. Here are a few examples:
- Budgeting: Imagine you have a budget of $100 to spend on groceries. Let 'x' represent the cost of groceries. The inequality x ≤ 100 represents the constraint that your grocery spending must be less than or equal to $100.
- Speed Limits: Speed limits are another example. If the speed limit is 65 mph, then the inequality v ≤ 65 represents the constraint that your speed (v) must be less than or equal to 65 mph.
- Manufacturing: In manufacturing, inequalities are used to ensure that products meet certain specifications. For instance, the length of a manufactured part might need to be within a certain tolerance range.
- Temperature Control: In many industries, maintaining a specific temperature range is critical. Inequalities are used to define and monitor these ranges, ensuring optimal operating conditions.
Advanced Concepts and Extensions
While we've solved a basic inequality, many more complex scenarios can arise. Here are some advanced concepts related to solving inequalities:
- Compound Inequalities: These involve multiple inequalities connected by "and" or "or." For example: 2x + 1 > 5 and x - 3 < 2.
- Absolute Value Inequalities: These involve absolute value expressions within inequalities, requiring careful consideration of positive and negative cases. For example: |x - 2| < 5.
- Polynomial Inequalities: These inequalities involve polynomial expressions of degree greater than one. Solving them often requires finding the roots of the polynomial and testing intervals.
- Rational Inequalities: These inequalities involve rational expressions (fractions with polynomials in the numerator and denominator). Solving them requires finding critical points where the numerator or denominator equals zero.
Troubleshooting Common Mistakes
Solving inequalities can be tricky. Here's a summary of common mistakes to avoid:
- Forgetting to reverse the inequality sign when multiplying or dividing by a negative number: This is the most frequent error. Always remember to flip the sign!
- Incorrectly handling compound inequalities: Pay close attention to the "and" or "or" connecting the inequalities and how it affects the solution set.
- Mistakes with absolute value inequalities: Be careful when considering the positive and negative cases.
- Errors in graphing the solution: Ensure the correct type of circle (open or closed) is used and that the shading represents the solution set accurately.
Conclusion
Mastering the skill of solving inequalities, such as -16x ≥ -48, is a fundamental step in your mathematical journey. This comprehensive guide provides a solid foundation, taking you from the basics to more advanced concepts. Remember to practice regularly, paying close attention to the nuances of inequality operations, to build your confidence and accuracy. With consistent effort, you'll become proficient in solving a wide range of inequalities and applying them to various real-world problems. Remember, understanding the underlying principles and practicing regularly are key to mastering this essential algebraic skill.
Latest Posts
Latest Posts
-
Each Orbital Can Hold How Many Electrons
Apr 06, 2025
-
5 Letter Words Beginning With Thu
Apr 06, 2025
-
Isotopes Of The Same Element Have Different
Apr 06, 2025
-
Least Common Multiple Of 3 4 And 9
Apr 06, 2025
-
How Many Glasses In 1 Liter Of Water
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Negative 16 X Greater Or Equal Than Negative 48 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
