Lowest Common Denominator Of 12 And 9
Juapaving
Apr 01, 2025 · 5 min read
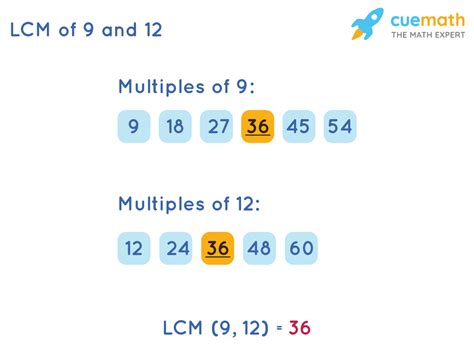
Table of Contents
Finding the Lowest Common Denominator (LCD) of 12 and 9: A Comprehensive Guide
Finding the lowest common denominator (LCD) is a fundamental concept in arithmetic, crucial for adding and subtracting fractions. This comprehensive guide will walk you through multiple methods for determining the LCD of 12 and 9, exploring the underlying mathematical principles, and providing practical examples to solidify your understanding. We'll also delve into the broader implications of LCDs in various mathematical applications.
Understanding Lowest Common Denominator (LCD)
The lowest common denominator, also known as the least common multiple (LCM), represents the smallest whole number that is a multiple of two or more given numbers. When working with fractions, the LCD allows us to express fractions with a common denominator, making addition and subtraction straightforward. For instance, adding ½ and ⅓ requires finding their LCD, which is 6, allowing us to rewrite the fractions as 3/6 and 2/6, respectively, for easy addition.
Methods for Finding the LCD of 12 and 9
Several effective methods can be employed to determine the LCD of 12 and 9. Let's explore the most common approaches:
Method 1: Listing Multiples
This method involves listing the multiples of each number until a common multiple is found. The smallest common multiple is the LCD.
- Multiples of 12: 12, 24, 36, 48, 60, 72, ...
- Multiples of 9: 9, 18, 27, 36, 45, 54, 72, ...
Observing the lists, we see that 36 is the smallest number present in both sequences. Therefore, the LCD of 12 and 9 is 36.
Method 2: Prime Factorization
This method is particularly useful for larger numbers and involves breaking down each number into its prime factors. The LCD is then constructed by taking the highest power of each prime factor present in the factorizations.
- Prime factorization of 12: 2² x 3
- Prime factorization of 9: 3²
The prime factors involved are 2 and 3. The highest power of 2 is 2², and the highest power of 3 is 3². Therefore, the LCD is 2² x 3² = 4 x 9 = 36.
Method 3: Greatest Common Divisor (GCD) Method
This method leverages the relationship between the LCD and the greatest common divisor (GCD) of two numbers. The formula connecting the LCD (LCM) and GCD is:
LCM(a, b) = (a x b) / GCD(a, b)
First, we find the GCD of 12 and 9 using the Euclidean algorithm or prime factorization.
- Prime factorization of 12: 2² x 3
- Prime factorization of 9: 3²
The common factor is 3, making the GCD of 12 and 9 equal to 3.
Now, we apply the formula:
LCM(12, 9) = (12 x 9) / GCD(12, 9) = (12 x 9) / 3 = 108 / 3 = **36**
Therefore, the LCD of 12 and 9 is 36.
Practical Applications of LCD
Understanding LCDs is vital in various mathematical contexts:
1. Fraction Addition and Subtraction
As mentioned earlier, the LCD is essential for adding and subtracting fractions with different denominators. Without a common denominator, direct addition or subtraction isn't possible.
Example: Add ½ + ⅓
- Find the LCD of 2 and 3, which is 6.
- Rewrite the fractions with the LCD: (3/6) + (2/6)
- Add the numerators: 3 + 2 = 5
- The result is 5/6.
2. Solving Equations Involving Fractions
LCDs play a crucial role in simplifying equations containing fractions. Multiplying both sides of the equation by the LCD eliminates the denominators, making the equation easier to solve.
Example: Solve the equation (x/12) + (x/9) = 1
- Find the LCD of 12 and 9, which is 36.
- Multiply both sides of the equation by 36: 36 * (x/12) + 36 * (x/9) = 36 * 1
- Simplify: 3x + 4x = 36
- Combine like terms: 7x = 36
- Solve for x: x = 36/7
3. Ratio and Proportion Problems
LCDs are helpful in solving problems involving ratios and proportions, especially when dealing with fractions.
Example: A recipe calls for a ratio of 12 parts flour to 9 parts water. If you want to use 36 parts of flour, how much water do you need?
- The ratio is 12:9, which simplifies to 4:3.
- Set up a proportion: 12/9 = 36/x
- Find the LCD of 9 and 12 (which is 36) and simplify the equation.
- Cross-multiply: 12x = 9 * 36
- Solve for x: x = 27
4. Working with Mixed Numbers
Mixed numbers (a whole number and a fraction) require converting them to improper fractions before adding or subtracting. The LCD is used to find a common denominator for these improper fractions.
Advanced Concepts and Applications
The concept of LCD extends beyond basic arithmetic. It finds application in more advanced mathematical areas:
1. Abstract Algebra
In abstract algebra, the concept of least common multiple generalizes to other algebraic structures, providing insights into ideal theory and module theory.
2. Number Theory
Number theory extensively utilizes the concepts of LCM and GCD in exploring the properties of integers, including divisibility, prime factorization, and modular arithmetic.
Conclusion
Finding the lowest common denominator is a fundamental skill with far-reaching applications in mathematics. Understanding the various methods – listing multiples, prime factorization, and the GCD method – equips you with the tools to tackle diverse problems involving fractions, equations, ratios, and more. Mastering LCD calculation enhances your problem-solving abilities and expands your mathematical understanding, paving the way for more advanced studies in arithmetic and beyond. Remember that consistent practice is key to internalizing these concepts and applying them confidently.
Latest Posts
Latest Posts
-
How Many Cubic Feet Is 50 Quarts
Apr 02, 2025
-
How Many Inches Is 2 M
Apr 02, 2025
-
A Quadrilateral With One Pair Of Opposite Sides Parallel
Apr 02, 2025
-
Flip A Coin Roll A Die
Apr 02, 2025
-
Calculating Ka From A Titration Curve
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Lowest Common Denominator Of 12 And 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
