Least Common Denominator Of Rational Expressions Calculator
Juapaving
Mar 30, 2025 · 6 min read
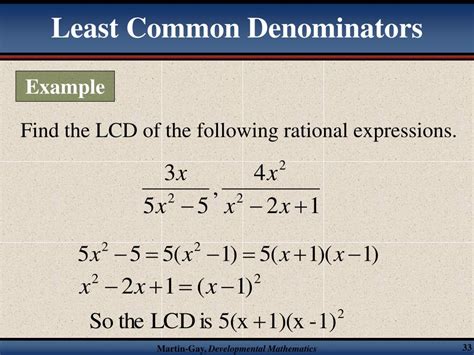
Table of Contents
Least Common Denominator of Rational Expressions Calculator: A Comprehensive Guide
Finding the least common denominator (LCD) of rational expressions can be a complex task, especially when dealing with intricate polynomials. This comprehensive guide will delve into the intricacies of calculating the LCD, explore various methods, and provide practical examples to solidify your understanding. We'll also discuss the benefits of using a least common denominator of rational expressions calculator and how to effectively utilize such a tool.
Understanding Rational Expressions and the LCD
Before diving into the mechanics of finding the LCD, let's revisit the fundamentals. A rational expression is simply a fraction where the numerator and denominator are polynomials. For instance, (3x² + 2x)/(x² - 4) is a rational expression.
The least common denominator (LCD) of two or more rational expressions is the simplest polynomial expression that contains all the factors of each denominator. It's crucial in adding, subtracting, and simplifying rational expressions, ensuring that we're working with a common base for our operations.
Methods for Finding the LCD
Several methods exist for determining the LCD, each with its own advantages and suitability depending on the complexity of the rational expressions involved.
1. Factoring the Denominators
This is the cornerstone of finding the LCD. The process involves factoring each denominator into its prime factors—irreducible polynomials that cannot be factored further. Once factored, the LCD is constructed by taking the highest power of each unique factor present in the denominators.
Example: Find the LCD of (2x)/(x² - 4) and (3)/(x + 2).
-
Factor the denominators:
- x² - 4 = (x - 2)(x + 2)
- x + 2 remains as (x + 2)
-
Identify unique factors: The unique factors are (x - 2) and (x + 2).
-
Determine the highest power: Both factors appear only to the first power.
-
Construct the LCD: The LCD is (x - 2)(x + 2).
2. Using Prime Factorization for Numerical Coefficients
When dealing with numerical coefficients in the denominators, it's beneficial to factor them into their prime factors as well. This helps in identifying the least common multiple (LCM) of the numerical parts, which then forms part of the overall LCD.
Example: Find the LCD of (5)/(6x) and (7)/(15x²).
-
Factor the numerical coefficients:
- 6 = 2 x 3
- 15 = 3 x 5
-
Factor the denominators:
- 6x = 2 x 3 x x
- 15x² = 3 x 5 x x²
-
Identify unique factors and highest powers: Unique factors are 2, 3, 5, and x. The highest powers are 2¹, 3¹, 5¹, and x².
-
Construct the LCD: The LCD is 2 x 3 x 5 x x² = 30x².
3. Handling Common Factors
Efficiently handling common factors is vital for simplifying the calculation and ensuring the LCD is indeed the least common denominator. Avoid including the same factor multiple times unless it appears with a higher power in one of the denominators.
4. Dealing with Complex Polynomials
For rational expressions with complex polynomial denominators, employing polynomial long division or other factorization techniques might be necessary. This could involve using synthetic division, grouping, or the quadratic formula depending on the polynomial's structure.
The Role of a Least Common Denominator of Rational Expressions Calculator
While mastering the manual methods is crucial for a strong understanding of the underlying principles, utilizing a least common denominator of rational expressions calculator can significantly streamline the process, especially when dealing with complex expressions. These calculators automate the factorization and LCD calculation, freeing you to focus on the application of the LCD in solving problems.
Benefits of using a calculator:
- Speed and efficiency: Calculators drastically reduce the time spent on calculations, particularly for complex expressions.
- Reduced errors: Manual calculations are prone to errors, especially when dealing with multiple factors and high powers. Calculators significantly mitigate this risk.
- Focus on problem-solving: By automating the LCD calculation, you can dedicate more time to understanding the bigger picture and applying the LCD to solve problems involving addition, subtraction, or simplification of rational expressions.
- Learning tool: Calculators can serve as a powerful learning tool. By comparing your manual calculations with the calculator's output, you can identify potential errors and improve your understanding of the process.
Practical Applications and Examples
The LCD is fundamental to several operations involving rational expressions:
1. Addition and Subtraction
Adding or subtracting rational expressions requires a common denominator. The LCD facilitates this, allowing us to combine the numerators while retaining the common denominator.
Example: Add (2x)/(x² - 4) + (3)/(x + 2).
-
Find the LCD: As calculated earlier, the LCD is (x - 2)(x + 2).
-
Rewrite the expressions with the LCD:
- (2x)/(x² - 4) = (2x)/((x - 2)(x + 2))
- (3)/(x + 2) = (3(x - 2))/((x - 2)(x + 2))
-
Add the numerators:
- (2x + 3(x - 2))/((x - 2)(x + 2)) = (5x - 6)/((x - 2)(x + 2))
2. Simplification
The LCD can be utilized to simplify complex rational expressions by canceling out common factors in the numerator and denominator.
Example: Simplify ((x² - 4)(x + 3))/((x + 2)(x² + 5x + 6)).
-
Factor the numerator and denominator:
- Numerator: (x - 2)(x + 2)(x + 3)
- Denominator: (x + 2)(x + 2)(x + 3)
-
Identify common factors: (x + 2) and (x + 3) are common factors.
-
Cancel the common factors: The simplified expression is (x - 2)/(x + 2).
3. Solving Equations
When solving equations involving rational expressions, the LCD plays a critical role in eliminating the denominators and simplifying the equation.
Choosing the Right Approach
The most effective approach to finding the LCD depends on the complexity of the expressions. For simple expressions, manual factorization is sufficient. However, for complex polynomials, a combination of manual factorization techniques and a calculator can significantly improve efficiency and accuracy.
Beyond the Basics: Advanced Techniques
As you progress, you'll encounter more complex scenarios, including:
- Expressions with higher-degree polynomials: These necessitate more sophisticated factoring techniques.
- Expressions with multiple variables: The process expands to include multiple variables in the LCD.
- Expressions involving irrational numbers: Additional considerations are necessary to handle irrational numbers.
Conclusion
Mastering the calculation of the least common denominator is essential for proficient work with rational expressions. While a solid understanding of the underlying principles and manual techniques is crucial, leveraging a least common denominator of rational expressions calculator can significantly enhance efficiency and accuracy, particularly when dealing with intricate expressions. Remember to always check your work and strive for a deeper understanding of the mathematical concepts involved. By combining manual skill with the power of computational tools, you can confidently tackle even the most challenging problems involving rational expressions.
Latest Posts
Latest Posts
-
The Proper Electron Dot Symbol For Aluminum Is
Apr 01, 2025
-
Biuret Test Shows The Presence Of
Apr 01, 2025
-
What Is The Distance Between Rarefactions Called
Apr 01, 2025
-
13 Gallons Is How Many Liters
Apr 01, 2025
-
How Is Mechanical Energy Converted To Electrical Energy
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Least Common Denominator Of Rational Expressions Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
