Is The Number 13 Prime Or Composite
Juapaving
Apr 02, 2025 · 6 min read
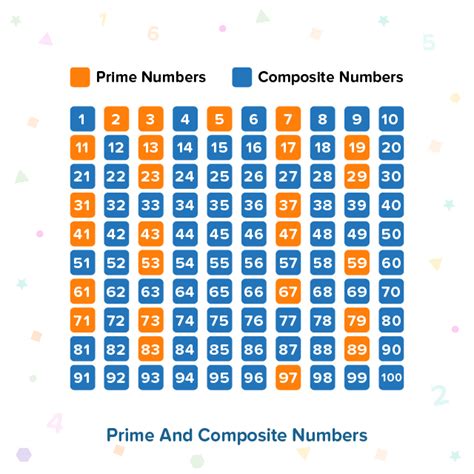
Table of Contents
Is the Number 13 Prime or Composite? A Deep Dive into Prime Numbers
The question, "Is the number 13 prime or composite?" might seem deceptively simple. However, understanding the answer requires a deeper exploration into the fascinating world of prime numbers – numbers that form the fundamental building blocks of arithmetic. This article will not only definitively answer the question about 13 but also provide a comprehensive understanding of prime and composite numbers, their properties, and their significance in mathematics.
Understanding Prime and Composite Numbers
Before we delve into the specifics of the number 13, let's define the key terms:
Prime Numbers: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. This means it cannot be factored into smaller whole numbers. Examples include 2, 3, 5, 7, 11, and so on. The number 1 is neither prime nor composite.
Composite Numbers: A composite number is a natural number greater than 1 that is not prime. In other words, it can be factored into smaller whole numbers besides 1 and itself. Examples include 4 (2 x 2), 6 (2 x 3), 9 (3 x 3), and so on.
The Significance of Prime Numbers: Prime numbers are fundamental to number theory and have numerous applications in cryptography, computer science, and other fields. Their unique properties make them crucial for secure communication and data encryption. Understanding prime numbers is a cornerstone of advanced mathematical concepts.
Determining if 13 is Prime or Composite
Now, let's address the main question: Is 13 prime or composite?
To determine this, we need to see if 13 has any positive divisors other than 1 and itself. Let's systematically check:
- Divisibility by 2: 13 is not divisible by 2 (it's an odd number).
- Divisibility by 3: 13 is not divisible by 3 (13/3 = 4 with a remainder of 1).
- Divisibility by 5: 13 is not divisible by 5 (13/5 = 2 with a remainder of 3).
- Divisibility by 7: 13 is not divisible by 7 (13/7 = 1 with a remainder of 6).
- Divisibility by 11: 13 is not divisible by 11 (13/11 = 1 with a remainder of 2).
We can stop here because the next number to check would be 13 itself. Since we've checked all the whole numbers less than the square root of 13 (approximately 3.6), and found no divisors other than 1 and 13, we can definitively conclude that:
13 is a prime number.
Exploring Properties of Prime Numbers
Prime numbers possess several intriguing properties that have fascinated mathematicians for centuries:
-
Infinitude of Primes: There are infinitely many prime numbers. This was proven by Euclid over two thousand years ago, and it's a fundamental result in number theory. The search for ever-larger primes continues to this day.
-
Distribution of Primes: While the primes are infinite, their distribution amongst the natural numbers is irregular. There are stretches of consecutive composite numbers, followed by gaps where primes are more densely packed. The Prime Number Theorem offers an approximation of the distribution, but pinpointing the exact location of the next prime is a complex problem.
-
Prime Factorization: Every composite number can be uniquely expressed as a product of prime numbers. This is known as the Fundamental Theorem of Arithmetic. This factorization is crucial for various mathematical applications, including cryptography. For example, 12 can be factored as 2 x 2 x 3 (2² x 3).
-
Twin Primes: Twin primes are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 11 and 13). Whether there are infinitely many twin primes remains one of the unsolved problems in mathematics.
-
Mersenne Primes: Mersenne primes are prime numbers of the form 2<sup>p</sup> - 1, where p is itself a prime number. These primes are particularly interesting because they are often very large, and the search for new Mersenne primes is a significant area of research. The Great Internet Mersenne Prime Search (GIMPS) is a distributed computing project dedicated to this pursuit.
Applications of Prime Numbers
The seemingly abstract world of prime numbers has far-reaching practical applications:
-
Cryptography: Prime numbers are the cornerstone of modern cryptography. Public-key cryptography systems, such as RSA, rely on the difficulty of factoring large composite numbers into their prime factors. This difficulty ensures the security of online transactions, secure communication, and data protection.
-
Hashing Algorithms: Prime numbers are used in hashing algorithms, which are essential for data integrity and efficient data retrieval. Hashing functions map data of arbitrary size to a fixed-size hash value. Prime numbers help in minimizing collisions and ensuring uniform distribution.
-
Random Number Generation: Prime numbers are utilized in pseudorandom number generators (PRNGs), which are widely used in simulations, statistical modeling, and computer graphics. The properties of prime numbers ensure a more uniform and less predictable distribution of random numbers.
-
Coding Theory: Prime numbers play a role in error-correcting codes, which are used to detect and correct errors in data transmission and storage. These codes are crucial for reliable communication in noisy environments.
Advanced Concepts Related to Prime Numbers
The study of prime numbers extends far beyond the basics. Here are a few advanced concepts:
-
The Riemann Hypothesis: One of the most important unsolved problems in mathematics, the Riemann Hypothesis deals with the distribution of prime numbers and the location of their zeros in the Riemann zeta function. Its resolution would have profound implications for number theory and related fields.
-
Goldbach's Conjecture: This conjecture states that every even integer greater than 2 can be expressed as the sum of two prime numbers. While extensively tested and believed to be true, it remains unproven.
-
The Sieve of Eratosthenes: This ancient algorithm provides a systematic way to find all prime numbers up to a given limit. It's a classic example of an algorithm for generating prime numbers.
-
Miller-Rabin Primality Test: This probabilistic test is used to determine whether a given number is prime or composite. It's efficient for large numbers where deterministic primality tests become computationally expensive.
Conclusion: The Prime Nature of 13 and its Broader Significance
We've definitively established that 13 is a prime number. However, the significance of this simple fact extends far beyond the number itself. The exploration of prime numbers reveals a fascinating landscape of mathematical concepts with practical applications that shape our modern world. From securing online transactions to enabling efficient algorithms, the properties of primes continue to drive innovation and advance our understanding of the fundamental building blocks of numbers. The continued study and exploration of prime numbers remain a vibrant area of mathematical research, promising further discoveries and advancements in the years to come. The seemingly simple question of whether 13 is prime or composite has, in fact, opened a door to a vast and compelling field of mathematical investigation.
Latest Posts
Latest Posts
-
Good Words To Describe Your Mother
Apr 03, 2025
-
How Many Bones Does Shark Have
Apr 03, 2025
-
Ca Oh 2 Acid Or Base
Apr 03, 2025
-
Quadrilateral With One Pair Of Parallel Sides
Apr 03, 2025
-
Any Change In Velocity Is Called
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Is The Number 13 Prime Or Composite . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
