Is 17 A Prime Number Or A Composite Number
Juapaving
Apr 02, 2025 · 5 min read
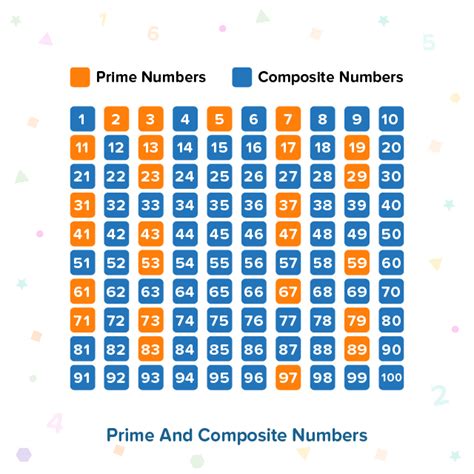
Table of Contents
Is 17 a Prime Number or a Composite Number? A Deep Dive into Number Theory
The question, "Is 17 a prime number or a composite number?" seems deceptively simple. However, exploring this seemingly basic question opens a window into the fascinating world of number theory, revealing fundamental concepts and elegant proofs that have captivated mathematicians for centuries. This article will not only definitively answer the question but also delve into the underlying principles that define prime and composite numbers, exploring related concepts and demonstrating their significance in mathematics and beyond.
Understanding Prime and Composite Numbers
Before we determine the classification of 17, let's establish the definitions of prime and composite numbers. These definitions form the bedrock of number theory and are essential for understanding the properties of integers.
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In other words, it's only divisible by 1 and itself without leaving a remainder. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. Notice that 2 is the only even prime number; all other even numbers are divisible by 2, and therefore, have more than two divisors.
Composite Number: A composite number is a natural number greater than 1 that is not a prime number. This means it has at least one positive divisor other than 1 and itself. For example, 4 (divisible by 1, 2, and 4), 6 (divisible by 1, 2, 3, and 6), and 9 (divisible by 1, 3, and 9) are composite numbers.
The Number 1: It's crucial to note that the number 1 is neither prime nor composite. This seemingly arbitrary exclusion is a consequence of fundamental theorems in number theory, such as the Fundamental Theorem of Arithmetic (which states that every integer greater than 1 can be uniquely represented as a product of prime numbers). Including 1 would break this fundamental theorem.
Determining if 17 is Prime or Composite
Now, let's address the central question: Is 17 a prime number or a composite number?
To determine this, we need to check if 17 has any divisors other than 1 and itself. We can systematically test for divisibility by prime numbers less than the square root of 17 (approximately 4.12). This optimization stems from the fact that if a number has a divisor larger than its square root, it must also have a divisor smaller than its square root.
Let's check the prime numbers less than 4.12:
- 2: 17 is not divisible by 2 (it's an odd number).
- 3: 17 is not divisible by 3 (1 + 7 = 8, which is not divisible by 3).
- 4: This is not a prime number, but we can use this to note that 17 is not divisible by 4.
- 5: 17 is not divisible by 5.
- 7: This is where it would get more interesting if we were assessing a different number. However, 17 is not divisible by 7.
Since 17 is not divisible by any prime number less than its square root, it follows that 17 is only divisible by 1 and itself.
Therefore, 17 is a prime number.
The Significance of Prime Numbers
Prime numbers are not merely abstract mathematical curiosities; they hold immense significance in various fields:
Cryptography:
Prime numbers are the cornerstone of modern cryptography. Algorithms like RSA (Rivest–Shamir–Adleman) rely heavily on the difficulty of factoring large numbers into their prime factors. The security of online transactions and sensitive data depends on the computational intractability of this problem.
Number Theory:
Prime numbers are fundamental building blocks in number theory. Many theorems and conjectures, such as the Riemann Hypothesis (one of the most important unsolved problems in mathematics), revolve around the distribution and properties of prime numbers.
Computer Science:
Prime numbers find applications in hashing algorithms, random number generation, and other computational processes. Their unique properties make them valuable tools in various computational tasks.
Other Applications:
Prime numbers even appear in unexpected places, such as in the design of certain types of error-correcting codes and in some aspects of physics and chemistry.
Exploring Related Concepts
Understanding prime numbers necessitates exploring related concepts within number theory:
Twin Primes:
Twin primes are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 11 and 13). The Twin Prime Conjecture postulates that there are infinitely many twin primes, but this remains unproven.
Mersenne Primes:
Mersenne primes are prime numbers of the form 2<sup>p</sup> - 1, where p is also a prime number. Finding Mersenne primes is a significant undertaking, often involving distributed computing projects like the Great Internet Mersenne Prime Search (GIMPS).
Goldbach's Conjecture:
This famous unsolved conjecture states that every even integer greater than 2 can be expressed as the sum of two primes. Despite extensive testing, a rigorous proof remains elusive.
Methods for Primality Testing
Determining whether a large number is prime can be computationally intensive. Several sophisticated algorithms have been developed to address this challenge:
-
Trial Division: This is the most basic method, involving testing for divisibility by all prime numbers up to the square root of the number in question. It is efficient only for relatively small numbers.
-
Probabilistic Primality Tests: These tests, like the Miller-Rabin test, don't definitively prove primality but provide a high probability that a number is prime or composite. They are significantly faster than deterministic tests for large numbers.
-
Deterministic Primality Tests: These tests, such as the AKS primality test, provide a definitive answer but are computationally more expensive than probabilistic tests.
Conclusion
The seemingly simple question of whether 17 is a prime or composite number has led us on a journey into the fascinating world of number theory. We've established that 17 is indeed a prime number, highlighting the fundamental definitions and exploring the broader significance of prime numbers in mathematics, cryptography, computer science, and beyond. The continuing exploration of prime numbers and related concepts drives advancements in various fields, showcasing the enduring power and elegance of this fundamental mathematical concept. The seemingly simple question of whether 17 is prime has served as a gateway to understanding far deeper mathematical concepts and their real-world applications, reminding us that even seemingly basic questions can unlock vast intellectual territories. Further exploration into number theory and the world of prime numbers is highly encouraged for anyone interested in the beauty and power of mathematics.
Latest Posts
Latest Posts
-
Common Factors Of 28 And 42
Apr 03, 2025
-
Can The Quotient Of Two Irrational Numbers Be Rational
Apr 03, 2025
-
12 Cm Is What In Inches
Apr 03, 2025
-
How Many Liters In 100 Gallons
Apr 03, 2025
-
Are Minerals A Renewable Resource Why Or Why Not
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Is 17 A Prime Number Or A Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
