Common Factors Of 28 And 42
Juapaving
Apr 03, 2025 · 6 min read
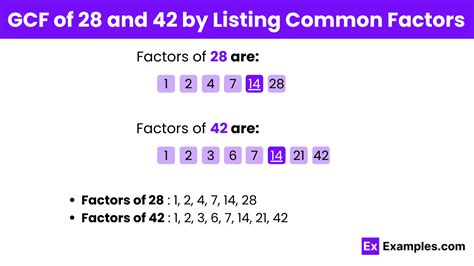
Table of Contents
Unveiling the Secrets of Common Factors: A Deep Dive into 28 and 42
Finding the common factors of two numbers might seem like a simple arithmetic exercise, but it's a fundamental concept in mathematics with far-reaching applications in various fields. This in-depth exploration will delve into the common factors of 28 and 42, going beyond a simple answer to uncover the underlying principles and their significance. We'll explore different methods for finding these factors, delve into the concept of the greatest common factor (GCF), and examine real-world applications where understanding common factors is crucial. We will also explore related concepts like prime factorization and least common multiple (LCM), showing how they interconnect.
Understanding Factors and Common Factors
Before we embark on our journey into the world of 28 and 42, let's clarify some fundamental concepts. A factor of a number is a whole number that divides evenly into that number without leaving a remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12.
A common factor, as the name suggests, is a factor shared by two or more numbers. These are the numbers that divide evenly into both numbers without leaving a remainder. Finding common factors is a key step in simplifying fractions, solving algebraic equations, and understanding relationships between different quantities.
Finding the Factors of 28 and 42
Let's begin by listing all the factors of 28 and 42 individually:
Factors of 28: 1, 2, 4, 7, 14, 28
Factors of 42: 1, 2, 3, 6, 7, 14, 21, 42
By comparing these two lists, we can easily identify the common factors of 28 and 42: 1, 2, 7, and 14. These are the numbers that divide evenly into both 28 and 42.
The Greatest Common Factor (GCF)
Among the common factors, the greatest common factor (GCF), also known as the highest common factor (HCF), holds particular importance. It's the largest number that divides evenly into both numbers. In the case of 28 and 42, the GCF is 14.
Understanding the GCF is vital for simplifying fractions. For example, the fraction 28/42 can be simplified to 2/3 by dividing both the numerator and denominator by their GCF, 14. This simplification makes the fraction easier to understand and work with.
Methods for Finding the GCF
Several methods can be used to determine the GCF of two numbers. Let's explore two common techniques:
1. Listing Factors Method
This is the method we used above. It involves listing all the factors of each number and then identifying the largest common factor. While straightforward for smaller numbers, this method can become cumbersome when dealing with larger numbers.
2. Prime Factorization Method
This method is more efficient for larger numbers. It involves expressing each number as a product of its prime factors. A prime factor is a whole number greater than 1 that has only two factors: 1 and itself (e.g., 2, 3, 5, 7, 11...).
Let's apply this method to 28 and 42:
- Prime factorization of 28: 2 x 2 x 7 (or 2² x 7)
- Prime factorization of 42: 2 x 3 x 7
To find the GCF, we identify the common prime factors and multiply them together. Both 28 and 42 share one factor of 2 and one factor of 7. Therefore, the GCF is 2 x 7 = 14.
Euclidean Algorithm: A More Efficient Approach for Larger Numbers
For significantly larger numbers, the prime factorization method can still be time-consuming. The Euclidean algorithm provides a more efficient approach. This algorithm is based on repeated application of the division algorithm. Let's illustrate it:
- Divide the larger number (42) by the smaller number (28): 42 ÷ 28 = 1 with a remainder of 14.
- Replace the larger number with the smaller number (28) and the smaller number with the remainder (14).
- Repeat the division: 28 ÷ 14 = 2 with a remainder of 0.
- The GCF is the last non-zero remainder, which is 14.
Real-World Applications of Common Factors and GCF
The concept of common factors and the GCF has numerous practical applications across various domains:
-
Fraction Simplification: As mentioned earlier, finding the GCF is essential for simplifying fractions to their lowest terms. This improves clarity and ease of calculation.
-
Measurement and Division: When dealing with measurements, determining the GCF helps in finding the largest common unit for measuring objects. For example, if you have two pieces of wood measuring 28 inches and 42 inches, the GCF (14 inches) tells you the largest length that will divide both evenly without leaving any remainder. This is useful when cutting or dividing materials efficiently.
-
Arranging Objects: Imagine you have 28 red marbles and 42 blue marbles. You want to arrange them into identical groups, each with the same number of red and blue marbles. To find the maximum number of groups you can make, calculate the GCF (14). You can form 14 identical groups, each containing 2 red marbles and 3 blue marbles.
-
Scheduling and Timing: In scheduling tasks or events that need to occur at regular intervals, the LCM (Least Common Multiple) is crucial, and understanding the GCF plays a role in calculating the LCM. For instance, if two machines complete cycles every 28 seconds and 42 seconds respectively, the LCM tells you when they will both complete a cycle simultaneously. The calculation of the LCM uses the GCF as an intermediary step.
-
Algebra and Number Theory: GCF is fundamental in simplifying algebraic expressions, solving Diophantine equations (equations with integer solutions), and various other problems in number theory.
Least Common Multiple (LCM): A Related Concept
While we've focused on the GCF, it's worth mentioning the closely related concept of the least common multiple (LCM). The LCM is the smallest number that is a multiple of both numbers. The LCM of 28 and 42 is 84.
There's a simple relationship between the GCF and LCM of two numbers (a and b):
LCM(a, b) x GCF(a, b) = a x b
Using this formula, we can verify the LCM of 28 and 42:
LCM(28, 42) x GCF(28, 42) = 28 x 42
LCM(28, 42) x 14 = 1176
LCM(28, 42) = 1176 / 14 = 84
Conclusion: The Significance of Understanding Common Factors
This exploration has revealed that finding the common factors of 28 and 42, and particularly the GCF, is more than just a basic arithmetic exercise. It's a fundamental concept with far-reaching implications across various mathematical fields and practical applications. Mastering this concept provides a solid foundation for tackling more complex mathematical problems and understanding the relationships between quantities in the real world. From simplifying fractions to scheduling tasks, the principles of common factors and the GCF are essential tools in our mathematical toolkit. Understanding these concepts allows for efficiency, precision, and a deeper appreciation of the underlying structure of numbers. The methods described – listing factors, prime factorization, and the Euclidean algorithm – equip you with the necessary skills to find common factors and GCFs for a wide range of numbers, strengthening your mathematical prowess and problem-solving abilities.
Latest Posts
Latest Posts
-
Cbse Class 6th Maths Book Pdf
Apr 04, 2025
-
How Many Atoms Are In Carbon
Apr 04, 2025
-
3 632 Rounded To The Nearest Tenth
Apr 04, 2025
-
2550 50 100 20 100 100 200
Apr 04, 2025
-
What Are Two Kinds Of Fermentation
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 28 And 42 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
