Integral 1 X 1 2 X 1 3
Juapaving
Mar 29, 2025 · 5 min read
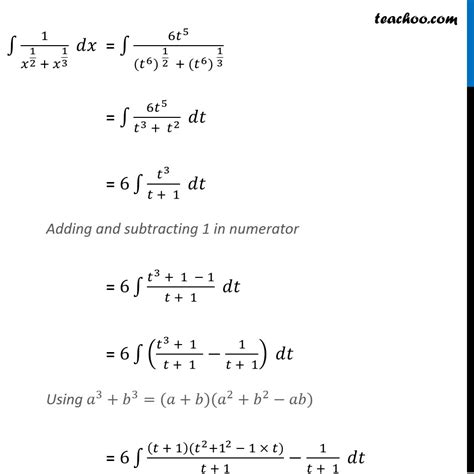
Table of Contents
Understanding and Solving the Integral ∫₁ˣ (1/2x)(1/3) dx
The seemingly simple integral ∫₁ˣ (1/2x)(1/3) dx presents a great opportunity to explore several key concepts in calculus, particularly concerning improper integrals and techniques of integration. This article will delve into a detailed explanation of how to approach this integral, highlighting potential pitfalls and offering a comprehensive understanding of the solution. We'll also touch upon related concepts to provide a broader perspective.
Deconstructing the Integral
First, let's clarify the integral: ∫₁ˣ (1/2x)(1/3) dx. This notation represents the definite integral of the function (1/2x)(1/3) with respect to x, evaluated from the lower limit 1 to the upper limit x. We can simplify the integrand:
(1/2x)(1/3) = 1/(6x)
Therefore, our integral becomes:
∫₁ˣ (1/(6x)) dx
Identifying the Type of Integral
This integral is an improper integral. An improper integral is a definite integral where one or both of the limits of integration are infinite, or where the integrand has a vertical asymptote within the interval of integration. In our case, the integrand 1/(6x) has a vertical asymptote at x = 0. Since our lower limit of integration is 1, this asymptote is outside our interval of integration [1, x]. However, the upper limit ‘x’ needs further consideration, and depending on its value, it can influence the nature of the integral and require a more careful examination.
Solving the Integral using the Power Rule
If we assume that x > 0 and that the interval of integration does not include the asymptote at x=0, we can proceed with solving the integral using the power rule of integration. Remember that the power rule states:
∫xⁿ dx = (xⁿ⁺¹)/(n+1) + C, where n ≠ -1
In our case, we have:
∫(1/(6x)) dx = (1/6) ∫(x⁻¹) dx
Notice that the exponent is -1, which is the exception in the power rule. The integral of x⁻¹ is not given by the power rule; instead, it is the natural logarithm function. Thus:
(1/6) ∫(x⁻¹) dx = (1/6) ln|x| + C
Where 'C' is the constant of integration.
Evaluating the Definite Integral
Now, we evaluate the definite integral:
∫₁ˣ (1/(6x)) dx = [(1/6) ln|x| + C]₁ˣ
Applying the limits of integration:
[(1/6) ln|x| + C] - [(1/6) ln|1| + C]
Since ln|1| = 0, the expression simplifies to:
(1/6) ln|x|
Therefore, the solution to the definite integral is (1/6) ln|x|, provided x > 0.
Important Considerations and Potential Pitfalls
Several critical points need emphasizing to ensure a complete understanding:
-
The Absolute Value: The presence of the absolute value within the natural logarithm, |x|, is crucial. The natural logarithm is only defined for positive arguments. The absolute value ensures the expression remains defined for both positive and negative values of x, provided x is not zero.
-
The Upper Limit x: The upper limit of integration is 'x,' which is a variable. The solution (1/6)ln|x| is therefore a function of x, representing the area under the curve of 1/(6x) from 1 to x.
-
The Lower Limit and the Asymptote: While the asymptote at x=0 doesn't directly affect this problem as stated, it highlights the critical nature of improper integrals. If the lower limit was changed to a value closer to 0 (like 0.001), the integral would still be solvable but would approach negative infinity as the lower limit approaches zero. This underlines the importance of carefully considering the interval of integration when working with functions that have vertical asymptotes.
-
The Constant of Integration: In definite integrals, the constant of integration 'C' cancels out. This is because we are subtracting the value of the integral at the lower limit from the value at the upper limit.
Expanding the Understanding: Exploring Related Concepts
To further solidify our understanding, let's explore some related concepts:
Improper Integrals with Infinite Limits
Consider the integral ∫₁^∞ (1/(6x)) dx. This represents an improper integral with an infinite upper limit. To evaluate it, we use a limit:
lim (b→∞) ∫₁^b (1/(6x)) dx = lim (b→∞) [(1/6) ln|b| - (1/6) ln|1|]
As b approaches infinity, ln|b| also approaches infinity. Therefore, this integral diverges – it does not have a finite value. This illustrates how a seemingly similar integral can have a drastically different outcome due to a change in the limits of integration.
Integrals with Asymptotes Within the Interval
Now, let's consider the integral ∫₋₁¹ (1/(6x)) dx. Here, the vertical asymptote at x=0 lies within the interval of integration [-1, 1]. This integral is also improper. To evaluate this, we must split it into two improper integrals:
∫₋₁⁰ (1/(6x)) dx + ∫₀¹ (1/(6x)) dx
Each integral must be evaluated as a limit. It turns out that both integrals diverge, meaning the original integral also diverges.
Applications and Real-World Relevance
While this particular integral might seem abstract, understanding how to solve it and the related concepts has practical applications in various fields:
-
Physics: Integrals are fundamental to solving problems in mechanics, electromagnetism, and thermodynamics. They allow us to calculate quantities like work, energy, and flux.
-
Engineering: Engineers use integrals to model and analyze systems, such as fluid flow, heat transfer, and structural mechanics.
-
Probability and Statistics: Integrals are used extensively to calculate probabilities and expectations in probability distributions.
Conclusion
The integral ∫₁ˣ (1/2x)(1/3) dx, while initially simple in appearance, provides a valuable learning experience. It highlights the importance of understanding the power rule, the correct application of integration techniques for different function types, the careful handling of improper integrals, and the implications of vertical asymptotes. By understanding these concepts, we gain a more profound comprehension of integral calculus and its wide-ranging applicability in various scientific and engineering disciplines. The seemingly simple integral serves as a springboard to a deeper understanding of more complex mathematical concepts. Remember to always carefully analyze the function, the limits of integration, and the potential presence of any asymptotes before attempting to solve any definite integral. This detailed examination will ensure accuracy and prevent common errors in solving complex problems involving integration.
Latest Posts
Latest Posts
-
What Does A Prokaryotic Cell Not Have
Apr 01, 2025
-
What Are More Things About The Major Components Of Soil
Apr 01, 2025
-
What Is The Lcm Of 5 6 7
Apr 01, 2025
-
Find The Complementary And Supplementary Angles
Apr 01, 2025
-
What Is The Specific Heat Of Liquid Water
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Integral 1 X 1 2 X 1 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
