How Do You Find The Perimeter Of A Semicircle
Juapaving
Mar 27, 2025 · 5 min read
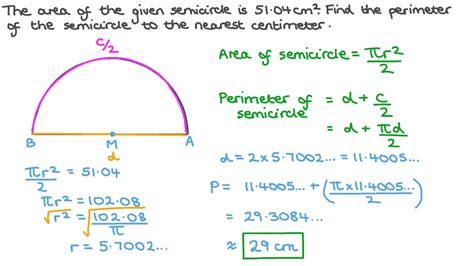
Table of Contents
How Do You Find the Perimeter of a Semicircle? A Comprehensive Guide
Finding the perimeter of a semicircle might seem straightforward, but understanding the nuances ensures accuracy and avoids common pitfalls. This comprehensive guide will delve into the process, exploring different approaches and providing practical examples. We'll also touch upon related geometrical concepts to enhance your understanding.
Understanding the Components of a Semicircle's Perimeter
Before diving into calculations, let's define the components contributing to a semicircle's perimeter. A semicircle is, simply put, half of a circle. Therefore, its perimeter comprises two distinct parts:
- The curved part (half the circumference): This is the arc representing half the circle's circumference.
- The diameter: This is the straight line segment connecting the two endpoints of the arc.
Therefore, the perimeter of a semicircle is the sum of the length of its curved portion and its diameter.
Formula for Calculating the Perimeter of a Semicircle
The formula for the perimeter (P) of a semicircle is derived from the circumference of a circle. The circumference of a circle is given by the formula C = 2πr, where 'r' represents the radius. Since a semicircle is half a circle, its curved portion has a length of (1/2) * 2πr = πr.
Adding the diameter (2r), the complete formula for the perimeter of a semicircle becomes:
P = πr + 2r
Alternatively, we can express this formula in terms of the diameter (d), where d = 2r:
P = (πd)/2 + d
Both formulas are equally valid, and the choice between them depends on the information given in the problem. If the radius is provided, the first formula is more convenient. If the diameter is given, the second formula is preferable.
Step-by-Step Calculation with Examples
Let's illustrate the calculation process with a few examples.
Example 1: Radius given
Suppose we have a semicircle with a radius of 7 cm. Using the formula P = πr + 2r, we substitute r = 7 cm:
P = π(7 cm) + 2(7 cm) P ≈ 21.99 cm + 14 cm P ≈ 35.99 cm
Therefore, the perimeter of the semicircle is approximately 35.99 cm. Remember to use an appropriate value for π (e.g., 3.14159 or the π button on your calculator) for greater accuracy.
Example 2: Diameter given
Consider a semicircle with a diameter of 10 inches. Using the formula P = (πd)/2 + d, where d = 10 inches:
P = (π * 10 inches) / 2 + 10 inches P ≈ 15.71 inches + 10 inches P ≈ 25.71 inches
Hence, the perimeter of this semicircle is approximately 25.71 inches.
Handling Different Units of Measurement
It's crucial to maintain consistency in units throughout the calculation. If the radius or diameter is given in centimeters, the perimeter will also be in centimeters. Similarly, if the dimensions are in inches, meters, or any other unit, the final answer will be in the same unit. Always double-check your units to avoid errors.
Advanced Applications and Related Concepts
The concept of calculating the perimeter of a semicircle extends beyond simple calculations. It forms the foundation for solving more complex problems in geometry and related fields.
1. Composite Shapes:
Semicircles often appear as parts of more complex shapes. For example, consider a figure consisting of a rectangle with semicircles attached to its ends. To find the perimeter of this composite figure, you need to calculate the perimeter of the rectangle (excluding the sides where the semicircles are attached) and add the perimeters of the two semicircles. Note that the two diameters of the semicircles will be accounted for by the sides of the rectangle. Thus you are essentially calculating the length of the rectangle's opposite sides along with the circumference of a full circle.
2. Arc Length Calculation:
Understanding the calculation of the arc length (the curved portion of the semicircle) is essential. This concept is vital in various applications, including calculating distances along curved paths or determining the amount of material needed for curved structures. The arc length is a significant part of the semicircle's perimeter.
3. Applications in Real-World Scenarios:
The ability to calculate a semicircle's perimeter is practical in various real-world scenarios:
- Construction: Determining the amount of fencing or other materials needed for a curved structure.
- Engineering: Calculating the length of curved pathways or pipes.
- Design: Designing aesthetically pleasing shapes incorporating semicircles.
- Manufacturing: Calculating the length of curved components in manufacturing processes.
Common Mistakes to Avoid
Several common mistakes can lead to inaccurate perimeter calculations:
- Forgetting the diameter: The most frequent error is neglecting to add the diameter to the arc length. Remember, the perimeter includes both the curved and straight parts.
- Incorrect use of the formula: Using the wrong formula or making errors during substitution can also lead to incorrect results. Always double-check your calculations.
- Inconsistent units: Maintaining consistency in units is crucial. Mixing units will lead to incorrect results.
- Approximation Errors: While using approximations for π is acceptable, using too crude an approximation will introduce significant errors, especially when dealing with larger semicircles.
Conclusion: Mastering Semicircle Perimeter Calculation
Calculating the perimeter of a semicircle is a fundamental geometric skill with practical applications across various fields. By understanding the formula, following the steps carefully, and avoiding common mistakes, you can accurately determine the perimeter and apply this knowledge to more complex geometric problems. Remember that precision is key, and paying close attention to detail ensures accurate results. Mastering this calculation empowers you to approach more advanced geometrical challenges with confidence. With practice and careful attention to detail, you will become proficient in calculating the perimeter of a semicircle and utilizing this knowledge in diverse real-world applications.
Latest Posts
Latest Posts
-
A Body Of Land Surrounded By Water On Three Sides
Mar 30, 2025
-
Is Square Root Of 15 An Irrational Number
Mar 30, 2025
-
When The Owner Withdraws Cash For Personal Use
Mar 30, 2025
-
What Is The Hybridization Of The Central Atom In Sf4
Mar 30, 2025
-
Model Of A Animal Cell With Labels
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about How Do You Find The Perimeter Of A Semicircle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
