Find The Area Between The Curves Calculator
Juapaving
Mar 26, 2025 · 6 min read
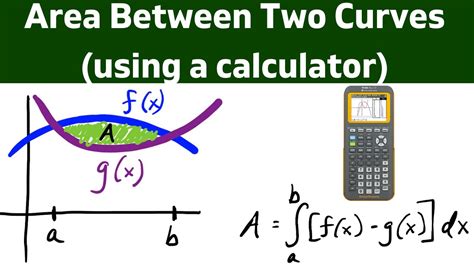
Table of Contents
Find the Area Between the Curves Calculator: A Comprehensive Guide
Finding the area between curves is a fundamental concept in integral calculus with applications across various fields, from physics and engineering to economics and statistics. While the underlying mathematical principles might seem daunting, numerous online tools, often referred to as "area between curves calculators," simplify this process significantly. This comprehensive guide will delve into the mechanics of calculating the area between curves, explore the functionality of area between curves calculators, and discuss their applications and limitations.
Understanding the Calculus Behind the Curves
Before diving into the practical application of calculators, let's solidify our understanding of the mathematical foundation. The area between two curves, f(x) and g(x), within a specified interval [a, b], is calculated using definite integrals. The formula is:
Area = ∫<sub>a</sub><sup>b</sup> |f(x) - g(x)| dx
This formula indicates that we're integrating the absolute difference between the two functions over the given interval. The absolute value is crucial; it ensures that we always obtain a positive area, regardless of which function is greater within the interval.
Breaking Down the Formula:
- ∫<sub>a</sub><sup>b</sup>: This represents the definite integral, which calculates the area under a curve between the limits a and b.
- |f(x) - g(x)|: This is the absolute difference between the two functions. This step is critical because the area calculation requires considering the magnitude of the difference, irrespective of whether f(x) or g(x) is larger at a given point.
- dx: This indicates that the integration is performed with respect to x.
Identifying the Interval [a, b]:
Determining the limits of integration (a and b) is crucial for accurate area calculation. These limits are usually found by identifying the points of intersection between the two curves. These intersection points represent the x-coordinates where f(x) = g(x). Solving this equation algebraically provides the values of a and b.
Handling Multiple Intersection Points:
If the curves intersect at more than two points, the calculation becomes slightly more complex. You'll need to break down the integral into separate parts, integrating over each sub-interval defined by the consecutive intersection points. In each sub-interval, the order of the functions in the absolute difference might change, depending on which function is above the other.
The Role of an Area Between Curves Calculator
While manual calculation is possible, especially for simpler functions, it can quickly become tedious and prone to errors for more complex equations. This is where an area between curves calculator comes in. These online tools automate the process, handling the integral calculation and providing the area between curves with minimal user input.
Key Features of a Typical Calculator:
- Function Input: Most calculators allow inputting functions using standard mathematical notation. This typically involves specifying the equations for f(x) and g(x).
- Interval Specification: The user needs to define the interval [a, b], either by directly inputting the limits or by specifying the intersection points. Some advanced calculators might automatically calculate the intersection points if provided with the functions.
- Output: The calculator directly provides the calculated area, often with a visual representation of the curves and the shaded area between them. This visualization helps verify the result and enhances understanding.
- Step-by-Step Solutions (Optional): Some advanced area between curves calculators offer step-by-step solutions, detailing the integration process. This feature is immensely valuable for educational purposes, as it allows users to learn from the process.
- Multiple Functions (Advanced): More sophisticated calculators allow for finding the area enclosed by more than two curves, adding layers of complexity and versatility.
Advantages of Using an Area Between Curves Calculator:
- Accuracy: Calculators eliminate the risk of human error in integral calculation, guaranteeing accurate results.
- Efficiency: The process is significantly faster than manual calculation, particularly for complex functions.
- Accessibility: These tools are readily available online, making them accessible to anyone with an internet connection.
- Visual Representation: The graphical output offers a clear visualization of the problem and the calculated area, improving comprehension.
- Educational Tool: Calculators with step-by-step solutions can be invaluable learning tools for students of calculus.
Limitations of Area Between Curves Calculators:
- Complexity Limitations: While advanced calculators can handle complex functions, extreme complexities might still present challenges.
- Dependence on Input: The accuracy of the results directly depends on the correctness of the input functions and limits.
- Lack of Understanding: Relying solely on calculators without understanding the underlying calculus can hinder deeper learning.
- Oversimplification: Calculators might not handle cases with intricate curves or piecewise functions efficiently. Understanding the piecewise nature of functions is crucial for accurate calculation.
Applications of Area Between Curves Calculations
The calculation of the area between curves finds applications in diverse fields:
1. Engineering and Physics:
- Calculating Work Done: In physics, the area between a force-displacement curve represents the work done by the force.
- Determining Fluid Flow: The area between curves in fluid dynamics helps to calculate the volume flow rate.
- Analyzing Stress-Strain Relationships: In material science, the area under stress-strain curves signifies the energy absorbed during deformation.
2. Economics:
- Consumer and Producer Surplus: In microeconomics, the area between supply and demand curves represents consumer and producer surplus, indicating the economic benefits to consumers and producers.
- Calculating Economic Losses/Gains: Changes in market conditions can be represented using curves, and the area between those curves show the associated economic impacts.
3. Statistics and Probability:
- Finding Probabilities: The area under a probability density function represents the probability of an event occurring within a specific range.
- Comparing Distributions: The area between two probability density functions can be used to compare different distributions.
4. Computer Graphics:
- Creating Shapes and Volumes: The calculation of areas between curves forms the basis for creating complex shapes and volumes in computer-aided design (CAD) software.
Advanced Concepts and Considerations
1. Integrating with Respect to y:
If it's more convenient or necessary, the area can be calculated by integrating with respect to y. This requires expressing the functions as x = f(y) and x = g(y), and the limits of integration will then be y-values. The formula becomes:
Area = ∫<sub>c</sub><sup>d</sup> |f(y) - g(y)| dy
where c and d are the y-coordinates of the intersection points.
2. Dealing with Regions Bounded by Multiple Curves:
In scenarios with several intersecting curves, the area calculation requires dividing the region into smaller sub-regions, calculating the area of each sub-region separately, and summing the individual areas to get the total area. This often involves careful identification of the limits of integration for each sub-region.
3. Applications of Numerical Integration:
For exceptionally complex functions or those without easily obtainable antiderivatives, numerical integration techniques, like the trapezoidal rule or Simpson's rule, are employed. Area between curves calculators often utilize these numerical methods behind the scenes for complex function inputs.
Conclusion: Empowering Calculation with Technology
Area between curves calculators are invaluable tools for anyone working with integral calculus. They drastically reduce calculation time and enhance accuracy, allowing for a focus on problem-solving and interpretation rather than tedious manual computations. However, it’s crucial to remember the underlying mathematical principles and limitations of these tools to ensure their effective and responsible use. By understanding both the calculus and the capabilities of these calculators, you'll be well-equipped to tackle various problems involving area calculations, enhancing your understanding and application of this fundamental concept.
Latest Posts
Latest Posts
-
1800 In Words For A Check
Mar 29, 2025
-
What Is The Lcm Of 5 6 And 7
Mar 29, 2025
-
How Much Valence Electrons Does Oxygen Have
Mar 29, 2025
-
What Percent Of 80 Is 12
Mar 29, 2025
-
Least Common Multiple Of 6 7 And 8
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Find The Area Between The Curves Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
