Electric Field Of A Spherical Shell
Juapaving
Apr 05, 2025 · 6 min read
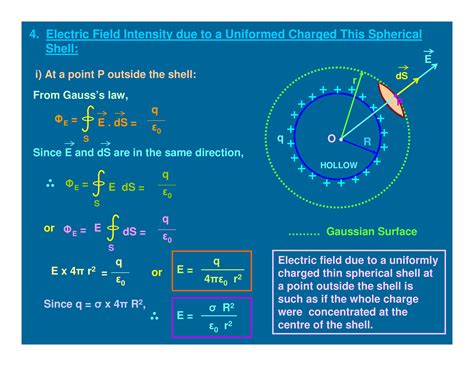
Table of Contents
The Electric Field of a Spherical Shell: A Comprehensive Guide
The electric field generated by a spherically symmetric charge distribution is a fundamental concept in electromagnetism with far-reaching applications in physics and engineering. Understanding this field is crucial for grasping more complex concepts like Gauss's Law and its implications for various systems. This article provides a comprehensive exploration of the electric field of a spherical shell, covering its derivation, properties, and practical significance.
Understanding the Problem: A Spherical Shell of Charge
We'll consider a non-conducting spherical shell with a total charge Q uniformly distributed across its surface. The shell has a radius R. Our goal is to determine the electric field E at any point in space, both inside and outside the shell. This problem elegantly demonstrates the power of Gauss's Law.
Gauss's Law: The Key to Solving the Problem
Gauss's Law states that the flux of the electric field through any closed surface is proportional to the enclosed charge. Mathematically, it's represented as:
∮ E ⋅ dA = Q<sub>enc</sub> / ε₀
where:
- E is the electric field vector
- dA is a differential area vector pointing outwards from the surface
- Q<sub>enc</sub> is the charge enclosed within the Gaussian surface
- ε₀ is the permittivity of free space (a constant)
The beauty of Gauss's Law lies in its ability to simplify calculations for systems with high symmetry, such as our spherical shell.
Deriving the Electric Field: Inside the Shell (r < R)
To find the electric field inside the shell (at a distance r < R from the center), we construct a spherical Gaussian surface with radius r concentric with the shell. Crucially, this Gaussian surface encloses no charge (all the charge resides on the shell's surface). Therefore, Q<sub>enc</sub> = 0.
Applying Gauss's Law:
∮ E ⋅ dA = 0 / ε₀ = 0
Since the electric field E is radial and the area vector dA is also radial, the dot product simplifies to:
E ∮ dA = 0
The integral ∮ dA is simply the surface area of our Gaussian sphere, 4πr². Therefore:
E (4πr²) = 0
This implies that E = 0 for r < R. This is a remarkable result: the electric field inside a uniformly charged spherical shell is zero.
Implications of Zero Electric Field Inside:
- Shielding: This property has significant implications for electrostatic shielding. A conducting spherical shell acts as a Faraday cage, protecting the interior from external electric fields.
- Electrostatic Equilibrium: The zero electric field ensures that no net force acts on any charge placed inside the shell, ensuring electrostatic equilibrium.
Deriving the Electric Field: Outside the Shell (r > R)
Now let's consider the electric field outside the shell (at a distance r > R). We construct another spherical Gaussian surface, this time with radius r > R. This surface now encloses the entire charge Q of the shell. Thus, Q<sub>enc</sub> = Q.
Applying Gauss's Law:
∮ E ⋅ dA = Q / ε₀
Again, due to the spherical symmetry, the electric field is radial and constant in magnitude on the Gaussian surface. The dot product simplifies, and we get:
E (4πr²) = Q / ε₀
Solving for the electric field E:
E = (1 / 4πε₀) * (Q / r²)
This is the familiar expression for the electric field due to a point charge Q located at the center of the sphere. This means that outside the spherical shell, the electric field behaves as if all the charge were concentrated at the center.
Implications of the External Electric Field:
- Coulomb's Law Similarity: The external field follows the inverse-square law, identical to the field of a point charge, highlighting the equivalence of a uniformly charged sphere and a point charge at the center from points outside the sphere.
- Applications: This result is vital for understanding the fields of many physical systems, including planets, stars, and atomic nuclei (as approximations).
Visualizing the Electric Field: A Graphical Representation
The electric field of a spherical shell can be effectively visualized using field lines. Inside the shell, no field lines exist, indicating zero electric field. Outside the shell, the field lines radiate outwards from the center, similar to those of a point charge, demonstrating the inverse-square dependence. This visual representation aids in understanding the discontinuity of the electric field at the shell's surface.
Beyond the Uniform Charge Distribution: Exploring Variations
The analysis above assumes a perfectly uniform charge distribution on the spherical shell. Let's briefly consider scenarios with non-uniform distributions:
- Non-uniform Surface Charge Density: If the charge distribution is not uniform, the calculation becomes considerably more complex, and Gauss's Law might not offer a straightforward solution. Numerical methods or more sophisticated techniques would likely be needed.
- Volume Charge Density: If the charge is distributed throughout the volume of a sphere instead of solely on the surface, the electric field calculation will also differ significantly, requiring a different approach to integrating the charge distribution.
Applications of the Spherical Shell Model: Real-World Examples
The concept of a spherical shell's electric field has far-reaching applications in various fields:
- Electrostatic Shielding: As mentioned earlier, the zero electric field inside a conducting spherical shell makes it an effective Faraday cage, protecting sensitive equipment from external electromagnetic interference. This is widely used in electronics and medical imaging.
- Nuclear Physics: The model provides a simplified representation of the electric field of an atomic nucleus, enabling the calculation of forces and interactions with other charged particles.
- Astrophysics: The electric fields of planets and stars can be approximated using the spherical shell model, especially when considering their overall charge distribution.
- Capacitors: Spherical capacitors consist of two concentric conducting shells, and understanding the electric field between them is crucial for determining their capacitance.
Advanced Concepts and Further Exploration
- Potential Difference: Calculating the potential difference between points inside and outside the shell provides additional insights into the energy associated with the electric field.
- Energy Density: Investigating the energy density of the electric field allows for a deeper understanding of the energy stored in the system.
- Multipole Expansion: For more complex charge distributions that deviate slightly from spherical symmetry, the concept of multipole expansion can be used to approximate the electric field.
Conclusion: The Significance of Understanding Spherical Shells
The electric field of a spherical shell is a fundamental concept in electromagnetism with diverse applications across many scientific and engineering disciplines. This article has provided a comprehensive overview of the derivation, properties, and implications of this field, emphasizing the power of Gauss's Law and the importance of understanding its applications. The insights gained from this study form a cornerstone for comprehending more complex electromagnetic phenomena. The spherical shell, while a simplified model, offers a powerful pedagogical tool to grasp the intricacies of electric fields and their behavior in various scenarios. Further exploration of the related advanced concepts will undoubtedly deepen one's understanding of this essential aspect of electromagnetism.
Latest Posts
Latest Posts
-
Are Diagonals Perpendicular In A Parallelogram
Apr 05, 2025
-
5 Letter Word Starts With Thi
Apr 05, 2025
-
Factors Of X 2 2x 3
Apr 05, 2025
-
How Many Feet In 77 Inches
Apr 05, 2025
-
A Bundle Of Muscle Fibers Is Known As A
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Electric Field Of A Spherical Shell . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
