Common Multiples Of 12 And 18
Juapaving
Mar 31, 2025 · 5 min read
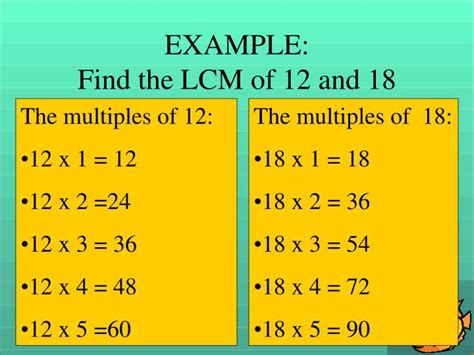
Table of Contents
Common Multiples of 12 and 18: A Deep Dive into Number Theory
Finding common multiples, especially for numbers like 12 and 18, might seem like a simple mathematical task. However, understanding the underlying concepts and exploring various methods for determining these multiples opens the door to a richer understanding of number theory and its applications. This article will delve deep into the world of common multiples, specifically focusing on 12 and 18, providing you with a comprehensive guide filled with examples, explanations, and practical applications.
Understanding Multiples and Common Multiples
Before we dive into the specifics of 12 and 18, let's establish a strong foundation. A multiple of a number is the product of that number and any integer. For example, multiples of 4 include 4 (4 x 1), 8 (4 x 2), 12 (4 x 3), 16 (4 x 4), and so on. These extend infinitely in both positive and negative directions.
A common multiple is a number that is a multiple of two or more numbers. For instance, if we consider the numbers 6 and 9, some of their multiples are:
- Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48, 54, 60...
- Multiples of 9: 9, 18, 27, 36, 45, 54, 63, 72, 81, 90...
Notice that some numbers appear in both lists. These are the common multiples of 6 and 9. In this case, 18, 36, and 54 are just a few examples of the common multiples of 6 and 9.
Finding Common Multiples of 12 and 18: Methods and Techniques
Now, let's focus on finding the common multiples of 12 and 18. We'll explore several methods, each offering a unique perspective and understanding.
1. Listing Multiples: A Simple Approach
The most straightforward method is to list the multiples of each number and identify the common ones.
- Multiples of 12: 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, 132, 144, 156, 168, 180...
- Multiples of 18: 18, 36, 54, 72, 90, 108, 126, 144, 162, 180, 198, 216...
By comparing these lists, we can readily identify the common multiples: 36, 72, 108, 144, 180, and so on. This method works well for smaller numbers, but it becomes cumbersome and prone to error for larger numbers.
2. Prime Factorization: A Powerful Tool
Prime factorization provides a more elegant and efficient approach. Let's find the prime factorization of 12 and 18:
- 12 = 2² x 3
- 18 = 2 x 3²
To find the least common multiple (LCM), we take the highest power of each prime factor present in either factorization: 2² x 3² = 4 x 9 = 36. The LCM is the smallest common multiple. All other common multiples are multiples of the LCM. Therefore, the common multiples of 12 and 18 are multiples of 36: 36, 72, 108, 144, 180, and so on.
3. Using the Formula: LCM and GCD
The relationship between the least common multiple (LCM) and the greatest common divisor (GCD) provides another powerful method. The formula is:
LCM(a, b) x GCD(a, b) = a x b
First, let's find the GCD of 12 and 18 using the Euclidean algorithm or prime factorization. The GCD is 6. Now, we can use the formula:
LCM(12, 18) x 6 = 12 x 18 LCM(12, 18) = (12 x 18) / 6 = 36
Again, we find that the LCM is 36, and all other common multiples are multiples of 36.
Least Common Multiple (LCM) and its Significance
The least common multiple (LCM) holds particular importance. It's the smallest positive integer that is divisible by both numbers. In our case, the LCM of 12 and 18 is 36. This value has practical applications in various scenarios:
- Fractions: Finding the LCM is crucial when adding or subtracting fractions. It allows us to find a common denominator, simplifying the calculation.
- Scheduling: Imagine two events occurring at regular intervals. One event happens every 12 hours, and another every 18 hours. The LCM (36 hours) determines when both events will coincide.
- Cyclic Patterns: Many real-world phenomena exhibit cyclical patterns. The LCM helps determine when these cycles will align.
Infinite Nature of Common Multiples
It's crucial to remember that the common multiples of 12 and 18 are infinite. We've identified 36 as the LCM, but we can generate an infinite number of common multiples by multiplying 36 by any integer:
36 x 1 = 36 36 x 2 = 72 36 x 3 = 108 36 x 4 = 144 ...and so on.
This infinite nature highlights the boundless possibilities within number theory.
Applications in Real-World Scenarios
The concepts of multiples and common multiples extend far beyond theoretical mathematics. They find practical applications in diverse fields:
- Construction: Calculating the lengths of materials needed for projects often requires finding common multiples to ensure precise measurements and avoid wastage.
- Music: Musical harmony and rhythm are based on mathematical relationships, including common multiples, to create pleasing sounds.
- Computer Science: Algorithms and data structures often utilize concepts of multiples and LCM for efficient processing and management of data.
- Scheduling and Time Management: As mentioned earlier, determining coinciding events or optimizing schedules often relies on finding common multiples.
Beyond the Basics: Exploring Advanced Concepts
While this article focuses on the common multiples of 12 and 18, the fundamental concepts extend to more complex scenarios:
-
Finding common multiples for more than two numbers: The methods discussed can be adapted to determine common multiples for three or more numbers. The LCM would be calculated by considering the highest powers of all prime factors present in the factorizations of all numbers.
-
Working with larger numbers: Prime factorization and the LCM/GCD relationship become even more valuable when dealing with larger numbers where listing multiples becomes impractical.
-
Exploring irrational numbers: While the focus here is on integers, the concepts of multiples and common multiples extend to other number systems. However, the calculation methods might differ.
Conclusion: A Foundation for Further Exploration
Understanding common multiples, particularly those of 12 and 18, provides a strong foundation for exploring more advanced concepts in number theory. The methods outlined in this article—listing multiples, prime factorization, and utilizing the LCM/GCD relationship—offer versatile tools for various mathematical problems and real-world applications. By mastering these techniques, you can confidently tackle complex calculations and gain a deeper appreciation for the elegance and practicality of mathematics. The infinite nature of common multiples also serves as a reminder of the vastness and interconnectedness of mathematical concepts. Continue your exploration, and you'll discover even more fascinating facets of this rich field.
Latest Posts
Latest Posts
-
Newtons First Law Examples In Everyday Life
Apr 01, 2025
-
15 4 As A Mixed Number
Apr 01, 2025
-
What Organelle Is The Site Of Aerobic Respiration
Apr 01, 2025
-
1 5 Gallons Is How Many Liters
Apr 01, 2025
-
How To Turn Gas Into Liquid
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Common Multiples Of 12 And 18 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
