An Object Following A Straight-line Path At A Constant Speed
Juapaving
Apr 05, 2025 · 5 min read
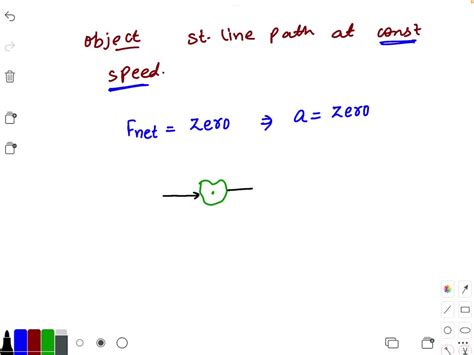
Table of Contents
An Object Following a Straight-Line Path at a Constant Speed: A Deep Dive into Uniform Linear Motion
Uniform linear motion, the concept of an object moving in a straight line at a constant speed, might seem simple at first glance. However, this seemingly straightforward idea forms the bedrock of classical mechanics and underpins many more complex physical phenomena. This comprehensive exploration will delve into the physics behind uniform linear motion, its mathematical representation, real-world examples, and its limitations. We'll also examine scenarios that appear to contradict this simple model and how we can analyze them.
Understanding Uniform Linear Motion
At its core, uniform linear motion (ULM) describes an object's movement along a straight path without any change in its velocity. Velocity, remember, is a vector quantity encompassing both speed (magnitude) and direction. Therefore, constant velocity implies both constant speed and a constant direction. Any deviation from a straight path or any alteration in speed signifies a departure from ULM.
Key Characteristics of ULM:
- Constant Speed: The object covers equal distances in equal intervals of time.
- Straight-Line Path: The object's trajectory is a straight line. No curves or changes in direction are involved.
- No Acceleration: Since both speed and direction remain constant, the object experiences zero acceleration. Acceleration is the rate of change of velocity. A change in either speed or direction results in acceleration.
Mathematical Representation of ULM
The simplicity of ULM translates into straightforward mathematical representations. The most fundamental equation is:
Distance (d) = Speed (v) × Time (t)
Where:
- d represents the distance traveled.
- v represents the constant speed.
- t represents the time elapsed.
This equation allows us to calculate any of the three variables if the other two are known. For instance, if we know the speed of a car and the time it travels, we can easily determine the distance covered. Conversely, knowing the distance and time, we can calculate the speed.
This equation is a simplification of more general kinematic equations that account for acceleration. Since acceleration is zero in ULM, these more complex equations reduce to this simpler form.
Real-World Examples (and the Limitations of the Model)
While perfectly uniform linear motion is an idealization, several real-world scenarios approximate ULM closely enough for practical applications:
- A car cruising on a straight highway at a constant speed: While minor variations in speed might occur due to slight changes in road incline or driver adjustments, the overall motion can be reasonably modeled as ULM.
- A puck sliding across frictionless ice: In a theoretical scenario with negligible friction, a puck would maintain a constant speed and direction, representing ULM. In reality, friction will cause the puck to decelerate.
- An object in freefall in a vacuum: In the absence of air resistance, an object falling under gravity would experience constant acceleration (due to gravity). However, if we consider horizontal motion, ignoring the effect of gravity in that plane, then we could approximate it to ULM.
- A train travelling on a long straight track at a constant speed: Similarly to the car example, minor variations in speed are likely, but the overall motion can be approximated as ULM.
Limitations of the ULM Model:
It's crucial to acknowledge the limitations of the ULM model. In the real world, perfect ULM rarely exists. Factors such as:
- Friction: Friction between moving objects and their surroundings always acts to slow down motion.
- Air Resistance: Air resistance opposes the motion of objects through the air, causing deceleration.
- Gravity: The force of gravity influences the motion of most objects, particularly vertically.
- Uneven Surfaces: Inaccuracies in the surface can cause deviations from a perfectly straight path.
These factors introduce acceleration, thus deviating from the idealized condition of ULM.
Analyzing Scenarios that Seem to Contradict ULM
Let's examine some scenarios that appear to challenge the concept of ULM but can still be analyzed using related physics principles:
Scenario 1: A ball rolling down a slope
A ball rolling down a slope does not exhibit ULM. Gravity causes it to accelerate. However, we can still analyze its motion using kinematic equations that incorporate acceleration. The distance traveled, the final speed, and the time taken can all be calculated using appropriate equations of motion.
Scenario 2: A projectile motion
Projectile motion, such as a ball thrown into the air, is clearly not ULM. The ball follows a curved path and experiences both horizontal and vertical accelerations (horizontal acceleration is near zero, if we ignore air resistance, but vertical acceleration is due to gravity). Again, we can analyze this using more advanced kinematic equations that consider both constant velocity (in the horizontal direction, with air resistance negligible) and constant acceleration (in the vertical direction).
Scenario 3: Circular motion
Circular motion, like a car going around a roundabout, is fundamentally different from ULM. The object's direction is constantly changing, which means it experiences centripetal acceleration, even if its speed is constant. This acceleration is directed towards the center of the circle and keeps the object moving in a circular path.
Applications of ULM Principles
Despite its limitations as a perfect representation of real-world motion, the concept of ULM forms the basis for understanding more complex forms of motion. Its principles are applied in various fields:
- Navigation: Simple navigation systems utilize ULM assumptions for short distances and constant speeds.
- Robotics: Programming robots to move in straight lines at constant speeds is a fundamental aspect of robotics programming.
- Traffic Engineering: Understanding ULM helps in designing efficient traffic flow and estimating travel times.
- Physics Simulations: ULM provides a starting point for more complex simulations involving various forces and accelerations.
Conclusion: The Importance of Idealized Models
While perfectly uniform linear motion is an idealization, its study remains crucial. It provides a foundational understanding of motion that serves as a building block for analyzing more complex scenarios. The simplicity of its mathematical representation makes it an accessible starting point for learning classical mechanics. By understanding the limitations of the ULM model and the factors that cause deviations from it, we can gain a deeper appreciation of the dynamics of motion in the real world. The ability to identify when ULM is a reasonable approximation and when more complex models are needed is key to applying physics effectively. Furthermore, analyzing scenarios that depart from ULM strengthens our understanding of the broader principles of kinematics and dynamics. This foundational knowledge provides the stepping stones for comprehending more sophisticated aspects of physics and engineering.
Latest Posts
Latest Posts
-
What Is The Si Unit Of Weight
Apr 05, 2025
-
A Particle Executes Simple Harmonic Motion
Apr 05, 2025
-
2 9 As A Decimal And Percent
Apr 05, 2025
-
Force Is A Scalar Or Vector Quantity
Apr 05, 2025
-
Are Diagonals Perpendicular In A Parallelogram
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about An Object Following A Straight-line Path At A Constant Speed . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
