2/9 As A Decimal And Percent
Juapaving
Apr 05, 2025 · 5 min read
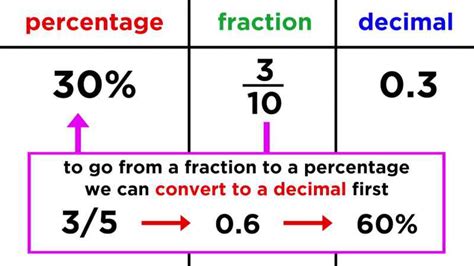
Table of Contents
2/9 as a Decimal and Percent: A Comprehensive Guide
Converting fractions to decimals and percentages is a fundamental skill in mathematics with widespread applications in various fields. This comprehensive guide delves into the process of converting the fraction 2/9 into its decimal and percentage equivalents, exploring the underlying concepts and offering practical examples. We'll also examine different methods for performing these conversions and discuss their significance in real-world scenarios.
Understanding Fractions, Decimals, and Percentages
Before diving into the conversion of 2/9, let's establish a clear understanding of the three key concepts: fractions, decimals, and percentages.
Fractions: A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates the number of parts considered, while the denominator indicates the total number of equal parts the whole is divided into. For example, in the fraction 2/9, 2 is the numerator and 9 is the denominator. This means we have 2 parts out of a total of 9 equal parts.
Decimals: A decimal is a number expressed in base-10, where the digits to the right of the decimal point represent fractions with denominators that are powers of 10 (10, 100, 1000, etc.). Decimals are a convenient way to represent fractional parts of a whole number. For instance, 0.5 is equivalent to 5/10 or 1/2.
Percentages: A percentage is a fraction or ratio expressed as a number out of 100. It represents a proportion of a whole, often expressed using the "%" symbol. For example, 50% means 50 out of 100, or 1/2, or 0.5.
Converting 2/9 to a Decimal
There are several ways to convert the fraction 2/9 to a decimal. The most common method involves long division.
Method 1: Long Division
To convert 2/9 to a decimal, we divide the numerator (2) by the denominator (9):
2 ÷ 9 = 0.22222...
Notice that the decimal representation of 2/9 is a repeating decimal. The digit "2" repeats infinitely. We can represent this using a bar over the repeating digit: 0.$\overline{2}$. This indicates that the "2" continues indefinitely.
Method 2: Using Equivalent Fractions
While less efficient for 2/9, this method can be useful for other fractions. We can try to find an equivalent fraction with a denominator that is a power of 10. However, in the case of 2/9, this is not easily achievable. 9 doesn't easily divide into powers of 10 (10, 100, 1000, etc.).
Converting 2/9 to a Percentage
Once we have the decimal equivalent of 2/9 (0.$\overline{2}$), converting it to a percentage is straightforward. We simply multiply the decimal by 100 and add the "%" symbol.
0.$\overline{2}$ x 100 = 22.222...%
Again, we have a repeating decimal. For practical purposes, we often round the percentage to a specific number of decimal places. For example, we might round 22.222...% to 22.22%, or even 22%. The level of precision required depends on the context of the problem.
Significance and Applications
The conversion of fractions to decimals and percentages is crucial in many real-world applications, including:
- Finance: Calculating interest rates, discounts, and profit margins often involves working with fractions, decimals, and percentages.
- Science: Representing experimental data and measurements often uses decimal and percentage notation.
- Engineering: Designing and building structures often requires precise calculations involving fractions and decimals.
- Everyday Life: Calculating tips, sales tax, and proportions in cooking recipes commonly utilizes these mathematical concepts.
Advanced Concepts and Related Topics
Let's explore some related mathematical concepts that deepen our understanding of fractions, decimals, and percentages.
Repeating Decimals
As we've seen with 2/9, some fractions result in repeating decimals. These are decimals where a sequence of digits repeats infinitely. Understanding how to represent and work with repeating decimals is important for accurate calculations.
Terminating Decimals
Conversely, some fractions result in terminating decimals – decimals that end after a finite number of digits. For example, 1/4 = 0.25. This occurs when the denominator of the fraction can be expressed as a product of 2s and/or 5s.
Converting Repeating Decimals to Fractions
The reverse process, converting a repeating decimal to a fraction, involves algebraic manipulation. For example, to convert 0.$\overline{3}$ to a fraction, we can let x = 0.$\overline{3}$. Then, 10x = 3.$\overline{3}$. Subtracting x from 10x gives 9x = 3, so x = 3/9 = 1/3.
Percentage Increase and Decrease
Calculating percentage increase or decrease is a common application of percentages. For example, if a price increases from $10 to $12, the percentage increase is calculated as [(12-10)/10] x 100% = 20%.
Applications in Statistics and Probability
Percentages play a vital role in statistical analysis and probability calculations, representing proportions and probabilities of events.
Practical Examples
Let's solidify our understanding with some practical examples:
Example 1: A student scored 2 out of 9 questions correctly on a quiz. What is their score as a percentage?
Solution: The fraction is 2/9. We already know this is approximately 22.22%. Therefore, the student scored approximately 22.22% on the quiz.
Example 2: A store offers a 20% discount on an item originally priced at $50. What is the discounted price?
Solution: The discount is 20% of $50, which is (20/100) x $50 = $10. The discounted price is $50 - $10 = $40.
Example 3: If you spend 2/9 of your day studying, what percentage of your day is spent studying?
Solution: 2/9 is approximately 22.22%, so you spend approximately 22.22% of your day studying.
Conclusion
Converting 2/9 to a decimal (0.$\overline{2}$) and a percentage (approximately 22.22%) involves fundamental mathematical concepts with broad real-world applications. Understanding the methods for performing these conversions and their significance is essential for anyone working with numbers and data. By mastering these skills, you’ll be better equipped to tackle various mathematical problems and make informed decisions in various aspects of life. This guide provides a comprehensive foundation for further exploration of related mathematical topics and their practical applications. Remember to choose the level of precision appropriate for the specific context you are working with.
Latest Posts
Latest Posts
-
Least Common Multiple Of 12 And 5
Apr 06, 2025
-
Cross Section Of A Sphere Parallel Perpendicular And Diagonal
Apr 06, 2025
-
The Main Circuit Board Of A Computer Is Called The
Apr 06, 2025
-
Mass Of An Alpha Particle In Kg
Apr 06, 2025
-
5 Letters Words With A And E
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about 2/9 As A Decimal And Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
