Write 78 As A Product Of Prime Factors
Juapaving
Mar 29, 2025 · 5 min read
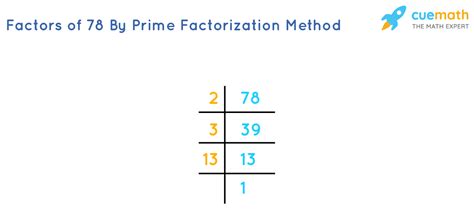
Table of Contents
- Write 78 As A Product Of Prime Factors
- Table of Contents
- Writing 78 as a Product of Prime Factors: A Deep Dive into Prime Factorization
- Understanding Prime Numbers and Prime Factorization
- Method 1: The Factor Tree Method
- Method 2: Repeated Division by Prime Numbers
- The Uniqueness of Prime Factorization: The Fundamental Theorem of Arithmetic
- Applications of Prime Factorization
- Beyond 78: Exploring Prime Factorization of Other Numbers
- Identifying Prime Numbers: A Quick Guide
- Challenges and Further Exploration in Prime Factorization
- Conclusion: The Enduring Significance of Prime Factorization
- Latest Posts
- Latest Posts
- Related Post
Writing 78 as a Product of Prime Factors: A Deep Dive into Prime Factorization
Prime factorization, the process of breaking down a number into its prime number components, is a fundamental concept in number theory with widespread applications in various fields like cryptography and computer science. This article will meticulously explore the prime factorization of 78, demonstrating the process step-by-step, explaining the underlying principles, and illustrating its broader significance within mathematics. We'll delve into different methods, explore related concepts, and even touch upon the practical uses of prime factorization.
Understanding Prime Numbers and Prime Factorization
Before we embark on factorizing 78, let's solidify our understanding of the key terms.
Prime Numbers: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. Note that 1 is not considered a prime number.
Prime Factorization: Prime factorization, also known as prime decomposition, is the process of finding the prime numbers that, when multiplied together, give the original number. Every composite number (a number that is not prime) can be uniquely expressed as a product of prime factors. This uniqueness is guaranteed by the Fundamental Theorem of Arithmetic.
Method 1: The Factor Tree Method
This is a visually intuitive method often used to introduce prime factorization to beginners.
-
Start with the number: Begin with the number 78.
-
Find a pair of factors: Find any two numbers that multiply to give 78. A simple starting point is often using the smallest prime number, 2. Since 78 is an even number, it's divisible by 2. 78 = 2 x 39.
-
Continue branching: Now, we consider each factor. 2 is already a prime number, so we stop branching from there. However, 39 is not prime. We can see that 39 = 3 x 13.
-
Break down until all are prime: Both 3 and 13 are prime numbers. We've reached the end of our branching.
-
Write the prime factorization: The prime factors are 2, 3, and 13. Therefore, the prime factorization of 78 is 2 x 3 x 13.
Here's a visual representation of the factor tree:
78
/ \
2 39
/ \
3 13
Method 2: Repeated Division by Prime Numbers
This method is more systematic and particularly useful for larger numbers.
-
Start with the smallest prime: Divide the number 78 by the smallest prime number, 2. 78 ÷ 2 = 39.
-
Continue dividing by primes: 39 is divisible by 3 (39 ÷ 3 = 13).
-
Repeat until you reach 1: 13 is a prime number. We've reached 1 after dividing by a prime.
-
Write the prime factorization: The prime factors are the numbers we divided by: 2, 3, and 13. Thus, the prime factorization of 78 is 2 x 3 x 13.
The Uniqueness of Prime Factorization: The Fundamental Theorem of Arithmetic
The Fundamental Theorem of Arithmetic states that every integer greater than 1 is either a prime number itself or can be represented uniquely as a product of prime numbers, disregarding the order of the factors. This theorem is fundamental to many areas of number theory. It means that no matter which method we use to find the prime factors of 78, we will always arrive at the same result: 2 x 3 x 13.
Applications of Prime Factorization
Prime factorization might seem like a purely mathematical exercise, but it has several significant real-world applications:
-
Cryptography: Modern cryptography heavily relies on the difficulty of factoring very large numbers into their prime components. RSA encryption, a widely used public-key cryptosystem, relies on this principle. The security of RSA depends on the fact that it's computationally expensive to factor the product of two large prime numbers.
-
Computer Science: Algorithms in computer science often use prime factorization concepts for tasks such as generating unique identifiers, managing data structures, and optimizing calculations.
-
Number Theory Research: Prime factorization is a core topic in number theory, leading to ongoing research in areas like the distribution of prime numbers, the Riemann Hypothesis, and related conjectures.
-
Coding Theory: Prime numbers play a crucial role in coding theory, specifically in error-correcting codes.
-
Modular Arithmetic: Prime factorization is essential in understanding modular arithmetic, a branch of number theory used in cryptography and computer science.
Beyond 78: Exploring Prime Factorization of Other Numbers
Let's extend our understanding by briefly looking at the prime factorization of some other numbers:
- 12: 2 x 2 x 3 (2² x 3)
- 24: 2 x 2 x 2 x 3 (2³ x 3)
- 36: 2 x 2 x 3 x 3 (2² x 3²)
- 100: 2 x 2 x 5 x 5 (2² x 5²)
- 105: 3 x 5 x 7
Identifying Prime Numbers: A Quick Guide
Identifying prime numbers is crucial for successful prime factorization. While there's no single simple formula to determine if a number is prime, here are some helpful techniques:
-
Trial division: Divide the number by all prime numbers less than its square root. If it's not divisible by any of them, it's prime.
-
Sieve of Eratosthenes: This is a more efficient algorithm for finding all prime numbers up to a specified limit.
-
Primality tests: For very large numbers, advanced primality tests are used to determine if a number is prime. These tests are computationally more efficient than trial division.
Challenges and Further Exploration in Prime Factorization
While the concept of prime factorization is relatively straightforward, the practical application, especially for very large numbers, can become computationally challenging. This complexity is what makes prime factorization crucial for the security of many cryptographic systems.
Furthermore, the distribution of prime numbers themselves is a fascinating area of ongoing mathematical research. Questions surrounding the gaps between primes and the density of primes continue to intrigue mathematicians.
Conclusion: The Enduring Significance of Prime Factorization
Prime factorization, despite its seemingly simple concept, underpins significant advancements in various fields. Understanding the method, its uniqueness, and its applications provides a solid foundation for further explorations in number theory, cryptography, and computer science. The prime factorization of 78 – 2 x 3 x 13 – serves as a simple yet powerful illustration of this fundamental mathematical concept and its enduring relevance. By grasping the principles involved in this seemingly basic factorization, we open the door to a deeper appreciation of the intricacies and practical implications of number theory.
Latest Posts
Latest Posts
-
The Fine Adjustment Knob On The Microscope
Apr 02, 2025
-
40 C By 70cm Kite Area
Apr 02, 2025
-
Is Denatured Alcohol And Rubbing Alcohol The Same
Apr 02, 2025
-
Do Prokaryotic Cells Have Membrane Bound Organelles
Apr 02, 2025
-
Common Multiples Of 4 And 5
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Write 78 As A Product Of Prime Factors . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
