Which Of The Following Are Rational Numbers
Juapaving
Apr 03, 2025 · 5 min read
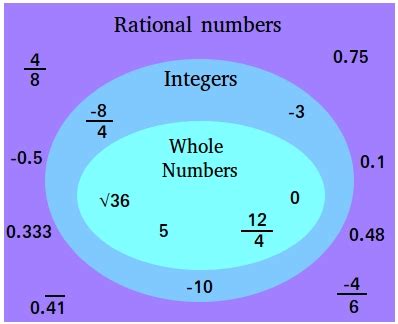
Table of Contents
Which of the Following are Rational Numbers? A Deep Dive into Rational and Irrational Numbers
Understanding rational and irrational numbers is fundamental to grasping the broader landscape of mathematics. This comprehensive guide will delve deep into the definition of rational numbers, explore various examples, and contrast them with irrational numbers. We'll also address common misconceptions and provide you with the tools to confidently identify rational numbers in any given set.
What are Rational Numbers?
A rational number is any number that can be expressed as a fraction p/q, where 'p' and 'q' are integers, and 'q' is not equal to zero. The key here is the ability to represent the number as a ratio of two whole numbers. This seemingly simple definition encompasses a surprisingly broad range of numbers.
Examples of Rational Numbers:
- Integers: All integers are rational numbers. For example, 5 can be expressed as 5/1, -3 as -3/1, and 0 as 0/1.
- Fractions: Any fraction where the numerator and denominator are integers (and the denominator is not zero) is a rational number. Examples include 1/2, 3/4, -2/5, and 7/10.
- Terminating Decimals: Decimals that end after a finite number of digits are rational numbers. For instance, 0.75 (which is 3/4), 2.5 (which is 5/2), and -0.125 (which is -1/8) are all rational.
- Repeating Decimals: Decimals that have a repeating pattern of digits are also rational. Numbers like 0.333... (which is 1/3), 0.666... (which is 2/3), and 0.142857142857... (which is 1/7) fall into this category. The repeating pattern is what allows these to be expressed as fractions.
Understanding Irrational Numbers: The Contrast
To truly appreciate rational numbers, we need to understand their counterpart: irrational numbers. Irrational numbers cannot be expressed as a fraction of two integers. Their decimal representation is neither terminating nor repeating; it goes on forever without any predictable pattern.
Examples of Irrational Numbers:
- √2: The square root of 2 is a classic example. Its decimal representation (approximately 1.41421356...) continues infinitely without repeating.
- π (Pi): The ratio of a circle's circumference to its diameter is an irrational number, approximately 3.14159... Its decimal expansion is infinite and non-repeating.
- e (Euler's number): This mathematical constant, approximately 2.71828..., is also irrational. Like π, it has an infinite, non-repeating decimal expansion.
- √3, √5, √7, etc.: The square roots of most non-perfect squares are irrational.
Identifying Rational Numbers: A Practical Approach
Let's move on to some practical exercises to solidify your understanding. Consider the following numbers:
-
2/3: This is clearly a rational number because it's expressed as a fraction of two integers.
-
-5: This is also a rational number because it can be written as -5/1.
-
0.625: This is a terminating decimal, and can be written as 5/8, making it a rational number.
-
1.414...: This is the decimal representation of √2, which is an irrational number because its decimal expansion is non-terminating and non-repeating.
-
0.333...: This repeating decimal is equivalent to 1/3, making it a rational number.
-
√9: The square root of 9 is 3, which is an integer and thus a rational number (3/1).
-
π/2: While π itself is irrational, this expression is not necessarily easy to categorize. Because π is irrational and 2 is rational, the result is irrational. Multiplying or dividing an irrational number by a rational number (excluding zero) results in an irrational number.
-
√(-4): This represents an imaginary number, not a rational or irrational real number. The realm of complex numbers extends beyond the scope of rational and irrational numbers.
Advanced Considerations and Common Misconceptions
Misconception 1: All decimals are irrational.
This is false. As we've seen, both terminating and repeating decimals are rational. Only non-terminating, non-repeating decimals are irrational.
Misconception 2: Rational numbers are always fractions.
While all rational numbers can be expressed as fractions, they can also be expressed as integers or terminating/repeating decimals. The crucial point is the possibility of expressing them as a ratio of two integers.
Misconception 3: Irrational numbers are useless.
Irrational numbers are extremely important in mathematics and various applications. Pi is crucial for calculating the circumference and area of circles, while Euler's number (e) appears in many areas of mathematics, including calculus and finance.
Working with Rational Numbers: Operations and Properties
Rational numbers obey the standard rules of arithmetic:
- Addition: Adding two rational numbers always results in another rational number.
- Subtraction: Similarly, subtracting two rational numbers yields a rational number.
- Multiplication: Multiplying two rational numbers also results in a rational number.
- Division: Dividing two rational numbers (where the divisor is not zero) yields a rational number.
These properties are essential when working with rational numbers in various mathematical contexts, from solving equations to performing calculations in scientific applications.
Real-World Applications of Rational Numbers
Rational numbers are ubiquitous in everyday life:
- Measurements: Most measurements we encounter are rational, such as 2.5 liters of milk, 3/4 of a cup of flour, or 1.5 meters of cloth.
- Money: Currency systems are based on rational numbers; $5.75 is a rational number.
- Fractions in recipes: Recipes often use fractional measurements, indicating rational numbers in action.
- Data representation: Computers often represent numbers using rational approximations.
Conclusion: Mastering the Distinction
The ability to distinguish between rational and irrational numbers is vital for a strong foundation in mathematics. This detailed guide has provided a robust understanding of rational numbers, illustrated through various examples and practical exercises. By understanding the defining characteristics of rational and irrational numbers, you’ll be better equipped to solve mathematical problems and tackle more advanced concepts. Remember to practice identifying rational numbers in different forms – fractions, decimals, and integers – to truly master this fundamental mathematical concept. This knowledge is crucial for progression to more complex mathematical ideas and real-world applications.
Latest Posts
Latest Posts
-
A Sound Wave Is An Example Of A Longitudinal Wave
Apr 04, 2025
-
Fundamental Building Block Of All Matter
Apr 04, 2025
-
Exterior Angle Of A Regular Hexagon
Apr 04, 2025
-
What Is The Radius Of Circle With Centre N
Apr 04, 2025
-
Pick Up The Incorrect Statement From The Following
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Which Of The Following Are Rational Numbers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
