What Percentage Is 3 Out Of 4
Juapaving
May 13, 2025 · 5 min read
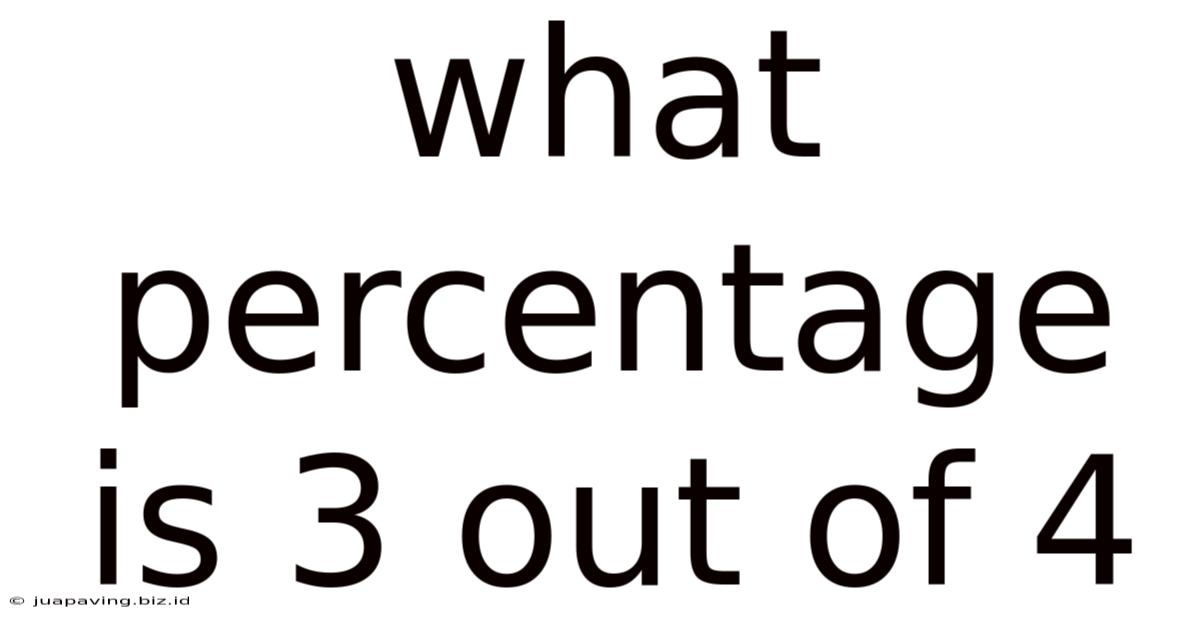
Table of Contents
What Percentage is 3 out of 4? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous aspects of life, from calculating discounts in a store to analyzing financial reports. This comprehensive guide will delve into the question, "What percentage is 3 out of 4?", providing not only the answer but also a thorough explanation of the underlying concepts and various methods for calculating percentages. We'll also explore real-world applications and tackle more complex percentage problems.
Understanding the Basics: Fractions, Decimals, and Percentages
Before we tackle the specific problem, let's solidify our understanding of the relationship between fractions, decimals, and percentages. They are all different ways of representing parts of a whole.
-
Fractions: Express a part of a whole using a numerator (top number) and a denominator (bottom number). For example, 3/4 represents three parts out of four equal parts.
-
Decimals: Express a part of a whole using a base-ten system. For example, 0.75 is the decimal equivalent of 3/4.
-
Percentages: Express a part of a whole as a fraction of 100. The symbol "%" represents "per hundred". For example, 75% represents 75 parts out of 100.
These three representations are interchangeable. Understanding this interchangeability is key to solving percentage problems.
Calculating the Percentage: 3 out of 4
The question, "What percentage is 3 out of 4?", can be solved using several methods. Here are two common approaches:
Method 1: Using the Fraction-to-Percentage Conversion
-
Express the values as a fraction: The problem "3 out of 4" translates directly to the fraction 3/4.
-
Convert the fraction to a decimal: Divide the numerator (3) by the denominator (4): 3 ÷ 4 = 0.75
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol (%): 0.75 x 100 = 75%.
Therefore, 3 out of 4 is 75%.
Method 2: Using Proportions
This method involves setting up a proportion. A proportion is an equation stating that two ratios are equal.
-
Set up the proportion: We can represent the problem as a proportion:
3/4 = x/100
Where 'x' represents the unknown percentage.
-
Cross-multiply: To solve for 'x', cross-multiply:
4x = 300
-
Solve for x: Divide both sides of the equation by 4:
x = 75
Therefore, 3 out of 4 is 75%.
Real-World Applications of Percentage Calculations
Understanding how to calculate percentages is crucial in many everyday situations:
-
Shopping: Calculating discounts, sales tax, and tips. For example, a 25% discount on a $100 item means a saving of $25.
-
Finance: Determining interest rates, loan payments, and investment returns. Understanding compound interest requires a solid grasp of percentages.
-
Grades and Scores: Calculating academic performance based on test scores and assignments. A score of 3 out of 4 questions correct would translate to a 75% score.
-
Statistics: Analyzing data sets to understand trends and patterns. Percentages are essential for presenting data in a clear and concise manner. For example, understanding response rates in surveys.
-
Cooking and Baking: Adjusting recipes based on the number of servings. If a recipe calls for 3 cups of flour for 4 servings and you need to make 8 servings, you'll need to adjust the amount of flour accordingly by calculating the necessary percentage increase.
Tackling More Complex Percentage Problems
While the "3 out of 4" example is straightforward, many percentage problems are more complex. Let's explore some variations:
Finding the Percentage Increase or Decrease
Calculating percentage change involves comparing an initial value to a final value.
Formula: [(Final Value - Initial Value) / Initial Value] x 100%
For example, if the price of an item increased from $50 to $60, the percentage increase is:
[(60 - 50) / 50] x 100% = 20%
Finding a Specific Value Given a Percentage
Sometimes, you know the percentage and need to find the original value or a portion of it.
For example, if 20% of a number is 10, what is the number?
Let the number be 'x'. Then, 0.20x = 10. Solving for x gives x = 50.
Calculating Percentages with Multiple Components
Some situations involve calculating percentages with more than two values. For example, you might need to calculate the percentage of each category in a budget.
Consider a budget with the following categories: Rent (40%), Food (30%), Transportation (20%), and Entertainment (10%). Understanding how these percentages are calculated and how they add up to 100% is essential for effective budgeting.
Improving Your Percentage Calculation Skills
Mastering percentage calculations involves practice and a thorough understanding of the underlying concepts. Here are some tips:
-
Practice Regularly: Work through various problems to reinforce your understanding. Start with simple problems and gradually increase the difficulty.
-
Use Multiple Methods: Try different approaches (like the fraction method and the proportion method) to solve problems. This will help you develop a deeper understanding of the concepts.
-
Check Your Work: Always verify your answers using different methods or by using a calculator to ensure accuracy.
-
Understand the Context: Pay attention to the context of the problem to correctly interpret the question and select the appropriate calculation method.
-
Utilize Online Resources: Numerous online resources, including calculators and tutorials, can help you practice and improve your understanding of percentages.
Conclusion
Understanding percentages is a versatile skill with far-reaching applications. This guide provided a thorough exploration of how to calculate percentages, using the example of "What percentage is 3 out of 4?", which equates to 75%. We explored various methods, including fraction-to-percentage conversion and proportion methods. We also delved into real-world applications and more complex percentage problems. By mastering these concepts and practicing regularly, you can confidently tackle percentage calculations in any context and apply this crucial skill to various aspects of your life. Remember, practice makes perfect, and with consistent effort, you'll become proficient in handling percentages effectively.
Latest Posts
Latest Posts
-
What Is 40 Percent Of 2000
May 13, 2025
-
Which Image Best Represents The Particles In Liquids
May 13, 2025
-
Which Part Of The Sperm Contains The Chromosomes
May 13, 2025
-
Is Burning Paper A Chemical Or Physical Change
May 13, 2025
-
What Is 1 3 Of 75000
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 3 Out Of 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.