What Is The Square Root 69
Juapaving
Apr 01, 2025 · 5 min read
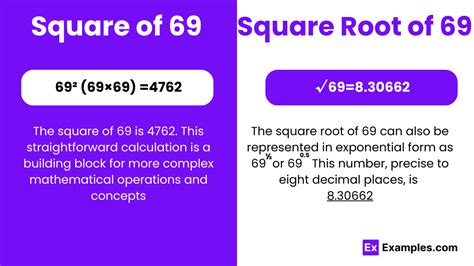
Table of Contents
What is the Square Root of 69? A Deep Dive into Square Roots and Numerical Approximation
The seemingly simple question, "What is the square root of 69?" opens a door to a fascinating exploration of mathematics, specifically the concept of square roots and the various methods used to approximate their values. While a simple calculator readily provides a decimal approximation, understanding the underlying principles and the history of calculating square roots enhances our mathematical literacy. This article will delve into the meaning of square roots, explore different methods for finding the square root of 69, and discuss the practical applications of such calculations.
Understanding Square Roots
A square root of a number is a value that, when multiplied by itself (squared), gives the original number. In mathematical notation, the square root of a number 'x' is denoted as √x. For instance, the square root of 9 (√9) is 3, because 3 * 3 = 9. Square roots are the inverse operation of squaring a number.
The number 69, however, isn't a perfect square – meaning there's no whole number that, when multiplied by itself, equals 69. This leads us to the need for approximation techniques to determine its square root.
Methods for Approximating √69
Several methods exist for approximating the square root of 69, each with varying levels of complexity and accuracy:
1. Using a Calculator: The Quick and Easy Method
The most straightforward approach is to use a calculator. Simply enter 69 and press the square root button (√). Most calculators will display a decimal approximation, typically around 8.30662386292. This method provides a rapid solution but offers little insight into the mathematical process.
2. The Babylonian Method (Heron's Method): An Iterative Approach
The Babylonian method, also known as Heron's method, is an iterative algorithm that refines an initial guess to progressively approximate the square root. It's based on the principle of averaging successive approximations.
Here's how it works:
-
Make an initial guess: Let's start with 8, as 8² = 64, which is close to 69.
-
Improve the guess: Divide the number (69) by the initial guess (8): 69 / 8 = 8.625
-
Average the guesses: Average the initial guess (8) and the result from step 2 (8.625): (8 + 8.625) / 2 = 8.3125
-
Repeat: Use the average from step 3 as the new guess and repeat steps 2 and 3 until the desired level of accuracy is achieved. Each iteration brings the approximation closer to the actual square root.
Let's perform another iteration:
- 69 / 8.3125 ≈ 8.300
- (8.3125 + 8.300) / 2 ≈ 8.30625
The Babylonian method converges relatively quickly to the true value. With each iteration, the approximation becomes more precise.
3. The Digit-by-Digit Method: A Manual Approach
This method involves a manual process of finding the square root digit by digit. It's more laborious than the Babylonian method but provides a deeper understanding of the underlying mechanics. The method is quite involved and requires a detailed explanation which is beyond the scope of this quick explanation. However, it's a fascinating method for those who enjoy exploring manual calculation techniques. Many resources are available online that detail the step-by-step procedure.
4. Using Logarithms: An Advanced Technique
Logarithms can be used to calculate square roots. The logarithm of a square root is half the logarithm of the original number. This method requires a logarithm table or a calculator with logarithmic functions.
Applications of Square Roots
The square root function isn't just a mathematical curiosity; it has widespread applications across various fields:
- Physics: Calculating velocity, acceleration, and other physical quantities often involves square roots. For example, in projectile motion calculations.
- Engineering: Structural engineers use square roots in calculations related to stress, strain, and stability. Designing bridges or buildings requires intricate calculations that utilize square roots.
- Geometry: Finding the lengths of diagonals in rectangles or the hypotenuse of right-angled triangles relies on the Pythagorean theorem, which involves square roots. This is crucial in fields like architecture, surveying, and cartography.
- Finance: Calculating standard deviation in financial modeling employs square roots. Understanding risk and volatility in investments hinges on this mathematical concept.
- Computer Graphics: Square roots are essential in rendering images and animations, especially in 3D graphics, to handle vector calculations and transformations.
- Statistics: Standard deviation, a measure of data dispersion, relies heavily on the square root. Understanding data variability is crucial across numerous fields.
Beyond the Calculation: Understanding the Significance
While the decimal approximation of √69 is readily available, the value lies not just in the numerical result but in the understanding of the underlying mathematical principles. The exploration of different approximation methods highlights the power of iterative processes and the elegance of mathematical algorithms. The wide range of applications across different disciplines emphasizes the practical relevance of square roots in our world. This seemingly simple calculation serves as a gateway to deeper mathematical knowledge and a better appreciation for the interconnectedness of mathematics and the real world.
Conclusion: The Square Root of 69 and Beyond
The square root of 69, approximately 8.3066, is more than just a number; it's a representation of a fundamental mathematical concept with far-reaching applications. By exploring different methods of approximation, we not only obtain the numerical answer but also gain a deeper understanding of mathematical processes and algorithms. This detailed analysis serves as a testament to the fascinating world of mathematics and its importance across various fields of study and application. The pursuit of knowledge extends beyond simply finding an answer; it's about grasping the 'why' and the 'how,' which ultimately empowers us to tackle more complex mathematical challenges and appreciate the beauty of mathematical principles.
Latest Posts
Latest Posts
-
Allows Materials In And Out Of The Cell
Apr 02, 2025
-
Which Of The Following Is Matched Correctly
Apr 02, 2025
-
Transitional Tissue Is Found In What System
Apr 02, 2025
-
Is There An Exception To The Law Of Segregation
Apr 02, 2025
-
5 Postulates Of Kinetic Molecular Theory
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root 69 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
