What Is The Prime Factorization Of 90
Juapaving
Apr 03, 2025 · 5 min read
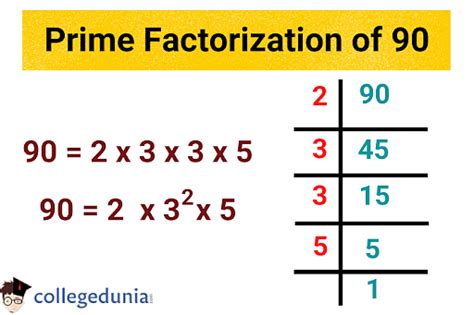
Table of Contents
What is the Prime Factorization of 90? A Deep Dive into Prime Numbers and Factorization
The seemingly simple question, "What is the prime factorization of 90?" opens a door to a fascinating world of number theory. While the answer itself is straightforward, understanding the process and the underlying concepts reveals the elegance and power of prime factorization in mathematics and computer science. This article will delve deep into finding the prime factorization of 90, explaining the method, its applications, and exploring related mathematical concepts.
Understanding Prime Numbers
Before tackling the prime factorization of 90, let's solidify our understanding of prime numbers. A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. The number 1 is not considered a prime number.
Prime numbers are the fundamental building blocks of all other natural numbers. This fundamental property is the cornerstone of prime factorization.
What is Factorization?
Factorization, in the context of number theory, is the process of expressing a composite number (a number that is not prime) as a product of its prime factors. This means breaking down the number into its smallest prime number components. For instance, the factorization of 12 is 2 x 2 x 3 (or 2² x 3).
Finding the Prime Factorization of 90: A Step-by-Step Approach
Now, let's find the prime factorization of 90. We can use a method called the factor tree.
-
Start with the number: We begin with 90.
-
Find the smallest prime factor: The smallest prime number that divides 90 is 2. So, we divide 90 by 2: 90 ÷ 2 = 45.
-
Continue factoring: Now we have 45. The smallest prime factor of 45 is 3. Dividing 45 by 3 gives us 15.
-
Repeat the process: 15 is divisible by 3, resulting in 5.
-
Prime Factor Achieved: 5 is a prime number. We've reached the end of our factorization.
Therefore, the prime factorization of 90 is 2 x 3 x 3 x 5, which can also be written as 2 x 3² x 5.
Visualizing with a Factor Tree
A factor tree visually represents this process:
90
/ \
2 45
/ \
3 15
/ \
3 5
Each branch ends with a prime number. The prime factorization is the product of all the prime numbers at the end of the branches.
The Uniqueness of Prime Factorization
A crucial theorem in number theory is the Fundamental Theorem of Arithmetic, which states that every integer greater than 1 can be represented uniquely as a product of prime numbers (ignoring the order of the factors). This means that no matter how you approach factoring a number, you will always arrive at the same set of prime factors. This uniqueness is vital in various mathematical applications.
Applications of Prime Factorization
Prime factorization, despite its seemingly simple nature, has profound applications in various fields:
1. Cryptography:
Prime factorization is the backbone of many modern encryption algorithms, such as RSA. The security of these systems relies on the difficulty of factoring extremely large numbers into their prime factors. Finding the prime factors of a sufficiently large number is computationally very expensive, making it practically impossible to break the encryption within a reasonable timeframe.
2. Computer Science:
Prime numbers play a crucial role in algorithms related to hashing, data structures, and random number generation. Understanding prime factorization aids in optimizing these algorithms and improving their efficiency.
3. Number Theory:
Prime factorization is central to numerous number theory problems and theorems. It underpins concepts like modular arithmetic, which has applications in cryptography and computer science.
4. Mathematics Education:
Understanding prime factorization helps build foundational skills in algebra, arithmetic, and abstract mathematical thinking. It's a stepping stone to more advanced topics in number theory and abstract algebra.
Beyond the Basics: Exploring Related Concepts
Let's explore some related concepts that deepen our understanding of prime factorization and number theory:
1. Greatest Common Divisor (GCD):
The GCD of two or more numbers is the largest number that divides all of them without leaving a remainder. Prime factorization is a powerful tool for finding the GCD. By finding the prime factorization of each number, we can easily identify the common prime factors and their lowest powers to calculate the GCD.
2. Least Common Multiple (LCM):
The LCM of two or more numbers is the smallest number that is a multiple of all the numbers. Similar to the GCD, prime factorization simplifies the calculation of the LCM.
3. Modular Arithmetic:
Modular arithmetic is a system of arithmetic for integers, where numbers "wrap around" upon reaching a certain value, called the modulus. Prime numbers play a crucial role in modular arithmetic, particularly in cryptography and coding theory.
4. Distribution of Prime Numbers:
The distribution of prime numbers among integers is a fascinating area of mathematical research. The Prime Number Theorem provides an approximation of the number of primes less than a given integer, showcasing the irregular but predictable pattern of prime numbers.
Conclusion: The Enduring Importance of Prime Factorization
The seemingly simple question of the prime factorization of 90 has led us on a journey through the fundamental concepts of number theory. From the definition of prime numbers to the powerful applications of prime factorization in cryptography and computer science, we've explored the depth and breadth of this essential mathematical concept. The uniqueness of prime factorization, as guaranteed by the Fundamental Theorem of Arithmetic, underpins many significant advancements in mathematics and its applications, showcasing the enduring importance of this seemingly simple process. Understanding prime factorization isn't just about finding the factors of a number; it's about understanding the building blocks of numbers themselves and their far-reaching implications in the world around us.
Latest Posts
Latest Posts
-
How Many Feet Is A 100 Yards
Apr 04, 2025
-
How Is Solar Energy Used In Everyday Life
Apr 04, 2025
-
Least Common Multiple Of 7 And 6
Apr 04, 2025
-
What Is 17 Cm In Inches
Apr 04, 2025
-
Are Acids Good Conductors Of Electricity
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is The Prime Factorization Of 90 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
