What Is The Prime Factor Of 135
Juapaving
Mar 31, 2025 · 5 min read
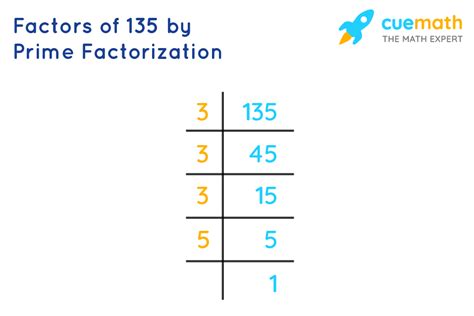
Table of Contents
What is the Prime Factor of 135? A Deep Dive into Prime Factorization
Finding the prime factors of a number might seem like a simple mathematical exercise, but it's a fundamental concept with far-reaching applications in various fields, from cryptography to computer science. This article will explore the prime factorization of 135 in detail, explaining the process step-by-step and highlighting the importance of prime numbers and their factorization in mathematics. We'll delve into different methods for finding prime factors and consider some related mathematical concepts.
Understanding Prime Numbers
Before we tackle the prime factorization of 135, let's refresh our understanding of prime numbers. A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself without leaving a remainder. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. The number 1 is not considered a prime number.
Prime numbers are the building blocks of all other natural numbers. This is because of the Fundamental Theorem of Arithmetic, which states that every integer greater than 1 can be represented uniquely as a product of prime numbers (ignoring the order of the factors). This unique representation is called the prime factorization of the number.
Methods for Finding Prime Factors
Several methods can be used to determine the prime factors of a number. Let's examine a few:
1. The Factor Tree Method
This is a visual method that's particularly helpful for smaller numbers like 135. We start by finding any two factors of the number and branching them out. We continue this process until all the branches end in prime numbers.
Let's apply this to 135:
- 135 = 5 x 27 (We find that 135 is divisible by 5)
- 27 = 3 x 9 (27 is divisible by 3)
- 9 = 3 x 3 (9 is also divisible by 3)
Therefore, the prime factorization of 135 is 3 x 3 x 3 x 5, or 3³ x 5.
2. Repeated Division Method
This method involves repeatedly dividing the number by the smallest prime number that divides it evenly until we are left with 1. We record the prime numbers used in the division.
Let's factorize 135 using this method:
- 135 ÷ 3 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
The prime factors are 3, 3, 3, and 5. Therefore, the prime factorization is 3³ x 5.
3. Using Divisibility Rules
Knowing divisibility rules for prime numbers can speed up the factorization process. For example:
- A number is divisible by 2 if it's an even number.
- A number is divisible by 3 if the sum of its digits is divisible by 3.
- A number is divisible by 5 if its last digit is 0 or 5.
Applying these rules to 135:
- 135 is not divisible by 2 (it's odd).
- 135 is divisible by 3 because 1 + 3 + 5 = 9, which is divisible by 3.
- 135 is divisible by 5 because its last digit is 5.
Using these rules, we can quickly start the factorization process.
The Prime Factorization of 135: A Detailed Explanation
As demonstrated by both the factor tree and repeated division methods, the prime factorization of 135 is 3³ x 5. This means 135 can be expressed as the product of three 3s and one 5. This representation is unique, according to the Fundamental Theorem of Arithmetic. There is no other way to express 135 as a product of prime numbers.
Significance of Prime Factorization
The seemingly simple process of prime factorization has significant implications across various mathematical and computational domains:
-
Cryptography: Prime numbers form the backbone of many modern encryption algorithms, such as RSA. The difficulty of factoring large numbers into their prime components is crucial to the security of these systems.
-
Number Theory: Prime factorization is a central theme in number theory, a branch of mathematics that explores the properties of integers. Many important theorems and concepts rely on the understanding of prime numbers and their factorization.
-
Computer Science: Algorithms for finding prime factors are essential in various computational tasks, including primality testing and the efficient factorization of large numbers.
-
Abstract Algebra: Prime factorization plays a role in understanding algebraic structures like rings and fields, which are fundamental concepts in abstract algebra.
Related Concepts and Further Exploration
Understanding prime factorization opens doors to exploring more advanced mathematical concepts:
-
Greatest Common Divisor (GCD): The GCD of two or more numbers is the largest number that divides all of them without leaving a remainder. Prime factorization can simplify the calculation of the GCD.
-
Least Common Multiple (LCM): The LCM of two or more numbers is the smallest number that is a multiple of all of them. Prime factorization helps in efficient LCM calculation.
-
Modular Arithmetic: Modular arithmetic involves performing arithmetic operations within a specified range (modulo). Prime numbers and their properties are critical in modular arithmetic.
Conclusion
The prime factorization of 135, 3³ x 5, is a simple yet powerful illustration of a fundamental concept in mathematics. The process of finding prime factors, seemingly straightforward, underlies many complex mathematical and computational applications. Understanding prime factorization enhances one's grasp of number theory, cryptography, and computer science, highlighting its importance in various fields. Exploring related concepts such as GCD and LCM, alongside a deeper dive into modular arithmetic, will further enrich your understanding of the significance of prime numbers in the broader mathematical landscape.
Latest Posts
Latest Posts
-
What Is The Prime Factorization 81
Apr 01, 2025
-
No Name This Compound According To Iupac Nomenclature Rules Responses
Apr 01, 2025
-
Why Does Heat Not Transfer Through Solids By Convection
Apr 01, 2025
-
Solid Has Definite Shape And Volume
Apr 01, 2025
-
What Animal Lays Eggs Thats Not A Bird
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Prime Factor Of 135 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
