What Is The Factors Of 89
Juapaving
Mar 27, 2025 · 5 min read
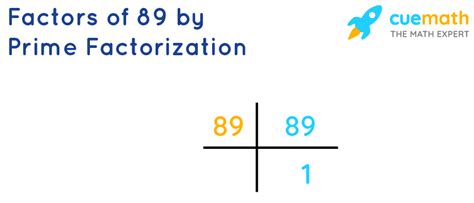
Table of Contents
What are the Factors of 89? Unraveling Prime Numbers and Divisibility
The question, "What are the factors of 89?" might seem deceptively simple. However, delving into its answer opens a door to understanding fundamental concepts in number theory, specifically prime numbers and divisibility rules. This article will not only answer the question directly but also explore the broader mathematical context, making the seemingly basic concept engaging and informative.
Understanding Factors
Before we tackle the factors of 89, let's define what a factor is. A factor (also known as a divisor) of a number is a whole number that divides the number exactly, without leaving a remainder. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12, because each of these numbers divides 12 evenly. Understanding factors is crucial in various mathematical operations, including simplification, factorization, and solving equations.
Identifying Factors: A Systematic Approach
There are several ways to identify the factors of a given number. For smaller numbers, we can often determine factors by simple mental calculation or using a multiplication table. However, as numbers grow larger, a more systematic approach is necessary.
One common method is to systematically check for divisibility by each integer starting from 1 up to the square root of the number. If a number divides the given number without a remainder, then it's a factor, and its corresponding quotient is also a factor. This method is particularly effective for identifying all factors efficiently. Let's illustrate this with a smaller number, say 24:
- Check divisibility by 1: 24 ÷ 1 = 24 (1 and 24 are factors)
- Check divisibility by 2: 24 ÷ 2 = 12 (2 and 12 are factors)
- Check divisibility by 3: 24 ÷ 3 = 8 (3 and 8 are factors)
- Check divisibility by 4: 24 ÷ 4 = 6 (4 and 6 are factors)
- Check divisibility by 5: 24 ÷ 5 = 4.8 (5 is not a factor)
- We stop at 4 because 4 x 4 = 16 < 24, but 5 x 5 = 25 > 24. We've found all the factors.
Therefore, the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. Notice that we only needed to check divisibility up to the square root of 24 (approximately 4.89).
Prime Numbers: The Building Blocks of Numbers
Prime numbers play a vital role in understanding factors. A prime number is a whole number greater than 1 that has only two distinct factors: 1 and itself. Examples of prime numbers include 2, 3, 5, 7, 11, and 13. Prime numbers are considered the "building blocks" of all other whole numbers, as every whole number greater than 1 can be expressed as a unique product of prime numbers (this is known as the Fundamental Theorem of Arithmetic).
The Factors of 89: A Prime Number Revelation
Now, let's return to our original question: What are the factors of 89?
Using the systematic approach described earlier, we would check for divisibility:
- Check divisibility by 1: 89 ÷ 1 = 89 (1 and 89 are factors)
- Check divisibility by 2: 89 ÷ 2 = 44.5 (2 is not a factor)
- Check divisibility by 3: 89 ÷ 3 = 29.66... (3 is not a factor)
- Check divisibility by 4: 89 ÷ 4 = 22.25 (4 is not a factor)
- Check divisibility by 5: 89 ÷ 5 = 17.8 (5 is not a factor)
- Check divisibility by 7: 89 ÷ 7 = 12.71... (7 is not a factor)
- ...and so on. We would continue checking until we reach the square root of 89 (approximately 9.43).
However, we'll find that no whole number other than 1 and 89 divides 89 evenly. This means that 89 is a prime number. Therefore, the only factors of 89 are 1 and 89.
Significance of Prime Numbers
The fact that 89 is a prime number is significant for several reasons:
-
Mathematical Foundations: Prime numbers are fundamental in number theory and cryptography. Their unique properties are used in various algorithms and security protocols.
-
Unique Factorization: As mentioned earlier, every whole number can be uniquely expressed as a product of prime numbers. This property is crucial in many mathematical proofs and computations.
-
Distribution and Patterns: The distribution of prime numbers is a fascinating area of mathematical research. While there isn't a simple formula to predict prime numbers, mathematicians have discovered interesting patterns and relationships among them.
-
Applications in Cryptography: The difficulty of factoring large numbers into their prime factors forms the basis of many modern cryptographic systems. This ensures the security of online transactions and sensitive data.
Beyond the Factors of 89: Exploring Divisibility Rules
While we've determined the factors of 89, it's beneficial to understand divisibility rules to quickly check for factors of other numbers. Divisibility rules are shortcuts that help us determine whether a number is divisible by certain integers without performing long division. Here are some common divisibility rules:
- Divisibility by 2: A number is divisible by 2 if its last digit is an even number (0, 2, 4, 6, or 8).
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3.
- Divisibility by 4: A number is divisible by 4 if the number formed by its last two digits is divisible by 4.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 6: A number is divisible by 6 if it is divisible by both 2 and 3.
- Divisibility by 9: A number is divisible by 9 if the sum of its digits is divisible by 9.
- Divisibility by 10: A number is divisible by 10 if its last digit is 0.
These divisibility rules can significantly speed up the process of finding factors, especially for larger numbers. They are also helpful in simplifying fractions and solving various mathematical problems.
Conclusion: The Simplicity and Depth of Prime Numbers
The seemingly simple question, "What are the factors of 89?", has led us on a journey into the fascinating world of prime numbers and divisibility. We've learned that 89 is a prime number, possessing only two factors: 1 and itself. This seemingly basic concept has far-reaching implications in mathematics and computer science. Understanding factors, prime numbers, and divisibility rules provides a solid foundation for tackling more complex mathematical problems and appreciating the beauty and elegance of number theory. The seemingly simple number 89 reveals a depth of mathematical significance that underscores the power and intricacy of numerical relationships.
Latest Posts
Latest Posts
-
Who First Used The Term Cell
Mar 30, 2025
-
Light Energy Is Converted To Chemical Energy In The
Mar 30, 2025
-
How Do You Add Scientific Notation
Mar 30, 2025
-
Solving Systems Of Equations By Elimination Solver
Mar 30, 2025
-
What Is The Valence Value Of Carbon
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about What Is The Factors Of 89 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
