What Is A Whole Number But Not A Natural Number
Juapaving
Apr 06, 2025 · 6 min read
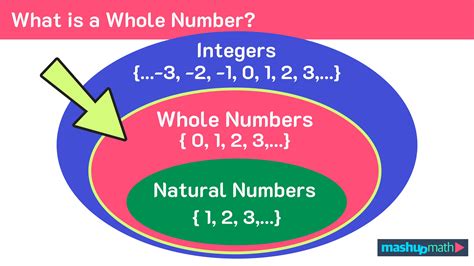
Table of Contents
What is a Whole Number But Not a Natural Number? Understanding the Subtle Difference
The world of mathematics can seem deceptively simple at first glance. However, a deeper dive reveals intricate layers of definitions and classifications, each with its own nuances and significance. One such area of subtle distinction lies within the seemingly straightforward concept of numbers. Many struggle to differentiate between whole numbers and natural numbers, often assuming they are interchangeable. This article aims to clarify the difference, focusing specifically on identifying numbers that are whole but not natural. We'll explore the definitions, provide examples, and delve into the implications of this distinction in various mathematical contexts.
Defining Whole Numbers and Natural Numbers
Before we can understand what constitutes a whole number that isn't a natural number, we need clear definitions:
Natural Numbers (Counting Numbers): These are the numbers we use for counting objects. They begin with 1 and continue infinitely: 1, 2, 3, 4, 5... Some mathematicians include 0 in the set of natural numbers, while others exclude it. For consistency throughout this article, we'll adopt the more common definition that excludes 0: Natural Numbers = {1, 2, 3, 4, 5,...}
Whole Numbers: This set includes all natural numbers and zero. Essentially, it's the set of natural numbers with the addition of 0. Whole Numbers = {0, 1, 2, 3, 4, 5,...}
The crucial difference lies in the inclusion of zero. Zero is a significant concept representing nothingness or the absence of quantity. While essential for many mathematical operations and systems, it's not used in the fundamental act of counting discrete objects. This is the root of the distinction between whole and natural numbers.
The Only Whole Number That is Not a Natural Number: Zero
Given the definitions above, the answer becomes strikingly clear: the only whole number that is not a natural number is zero (0).
This seemingly small difference has significant implications in various areas of mathematics and beyond.
Zero's Unique Properties
Zero possesses several unique properties that set it apart from other numbers:
- Additive Identity: Adding zero to any number does not change the number's value. This makes it the additive identity element. For example, 5 + 0 = 5.
- Multiplicative Annihilator: Multiplying any number by zero always results in zero. This property is crucial in many algebraic manipulations.
- Division by Zero: Division by zero is undefined in standard mathematics. This results from the fact that there's no number that, when multiplied by zero, would yield a non-zero result.
- Representation of Nothingness: Zero represents the absence of quantity, a crucial concept in various fields, from accounting to computer science.
These unique properties highlight zero's critical role in mathematical systems, even though it's not used in the act of counting in the same way as natural numbers.
Implications in Different Mathematical Contexts
The distinction between whole numbers and natural numbers isn't just a matter of semantics; it has practical consequences in various areas:
Set Theory
In set theory, the distinction is fundamental. The set of whole numbers is a superset of the set of natural numbers, meaning it contains all the elements of the natural number set and more (in this case, only zero). This relationship is often represented using set notation: ℕ ⊂ 𝕎, where ℕ represents the set of natural numbers and 𝕎 represents the set of whole numbers.
Number Systems
The inclusion or exclusion of zero impacts the characteristics of number systems. For instance, the properties of certain algebraic structures might change depending on whether zero is included (as in whole numbers) or excluded (as in natural numbers).
Programming and Computer Science
In programming, the distinction can influence data type definitions. Some programming languages might have specific data types for natural numbers (positive integers only) and others for whole numbers (including zero and positive integers). Understanding this difference is crucial for efficient coding and preventing errors.
Arithmetic Operations
While basic arithmetic operations (+, -, ×) work similarly for both whole and natural numbers, the impact of zero is evident in division. Division by zero is undefined, highlighting a key difference when working with these number sets.
Real-World Applications
Beyond abstract mathematics, the distinction can impact real-world applications. Consider inventory management: if you have zero items in stock, it's a valid whole number representing the stock level, but it's not a number you would use to count existing items (which would fall within the realm of natural numbers). Similarly, measuring temperature in Celsius or Fahrenheit often includes zero or negative values, which aren't natural numbers but fall under the umbrella of whole numbers (or even integers, a broader set).
Expanding the Understanding: Integers, Rational Numbers, and Beyond
To further clarify the position of whole numbers and natural numbers, let's briefly consider other number systems:
Integers: This set includes all whole numbers and their negative counterparts: {... -3, -2, -1, 0, 1, 2, 3,...}. Whole numbers are a subset of integers.
Rational Numbers: These are numbers that can be expressed as a fraction (a/b) where 'a' and 'b' are integers, and 'b' is not zero. Integers (and therefore whole and natural numbers) are a subset of rational numbers. Rational numbers encompass fractions, decimals that terminate or repeat, and integers.
Irrational Numbers: These are numbers that cannot be expressed as a fraction of two integers. They include numbers like π (pi) and √2 (square root of 2).
Real Numbers: This is the union of rational and irrational numbers, encompassing all numbers on the number line.
This hierarchy shows that natural numbers are a subset of whole numbers, which are a subset of integers, which are a subset of rational numbers, which are a subset of real numbers. Understanding this hierarchy provides a broader perspective on the role and significance of each number set.
Conclusion: A Subtle but Significant Difference
While the difference between whole numbers and natural numbers might seem trivial at first, it highlights a fundamental distinction in mathematical thought. The inclusion or exclusion of zero significantly impacts various aspects of mathematics, from set theory and number systems to programming and real-world applications. The unique properties of zero make it a critical element in mathematical structures, despite not being part of the fundamental process of counting individual objects. Understanding this subtle but significant difference is crucial for anyone looking to gain a deeper grasp of mathematical concepts and their practical implications. The single whole number that isn't a natural number—zero—plays a pivotal, unique, and indispensable role in the vast and fascinating landscape of mathematics.
Latest Posts
Latest Posts
-
Ground State Electron Configuration For Arsenic
Apr 07, 2025
-
A Single Carbon Atom Can Form A Maximum Of
Apr 07, 2025
-
Which Is A Biotic Component Of A Marine Ecosystem
Apr 07, 2025
-
How Does Pitch Relate To Frequency
Apr 07, 2025
-
How Many Lines Of Symmetry Does A Octagon Have
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about What Is A Whole Number But Not A Natural Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.