What Is 20 Percent Of 15000
Juapaving
May 09, 2025 · 5 min read
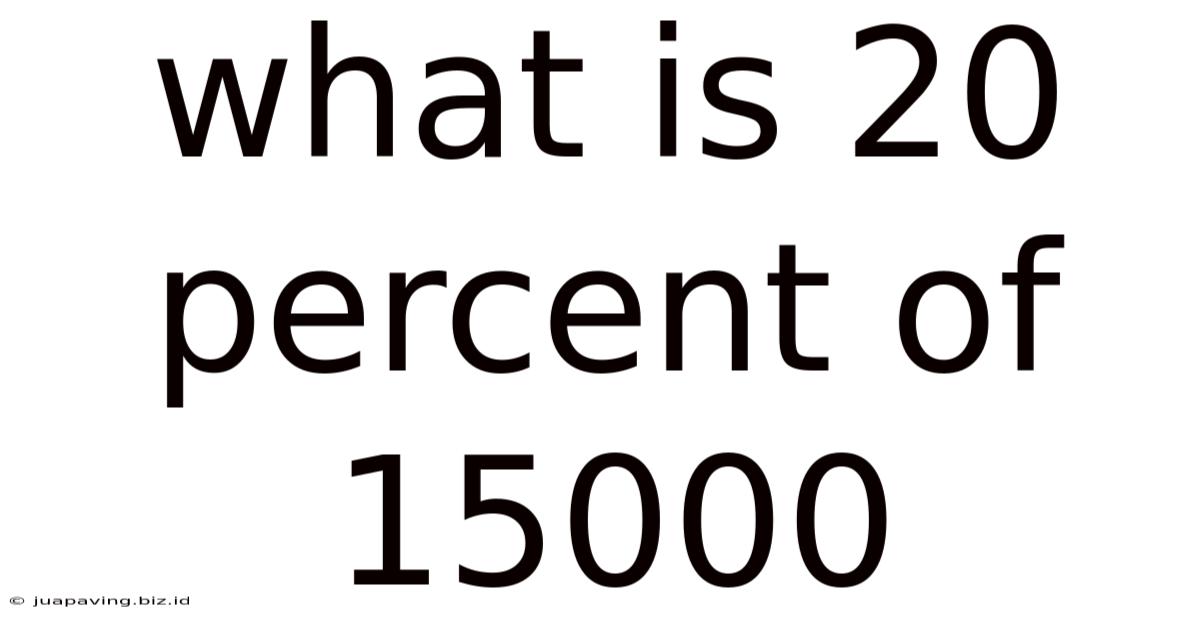
Table of Contents
What is 20 Percent of 15000? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from finance and budgeting to sales and discounts. Understanding how to calculate percentages empowers you to make informed decisions, analyze data effectively, and navigate numerical challenges confidently. This comprehensive guide will walk you through the process of determining what 20 percent of 15000 is, explaining the underlying principles and providing practical examples to solidify your understanding.
Understanding Percentages
Before diving into the calculation, let's refresh our understanding of percentages. A percentage is a fraction expressed as a part of 100. The symbol "%" represents "per cent" or "out of 100". For example, 20% means 20 out of 100, which can be written as the fraction 20/100 or the decimal 0.20.
Method 1: Using Decimal Multiplication
This is the most straightforward method for calculating percentages. It involves converting the percentage to a decimal and then multiplying it by the original number.
Steps:
-
Convert the percentage to a decimal: Divide the percentage by 100. In this case, 20% / 100 = 0.20.
-
Multiply the decimal by the original number: Multiply 0.20 by 15000. This calculation yields 0.20 * 15000 = 3000.
Therefore, 20% of 15000 is 3000.
This method is efficient and easily adaptable to various percentage calculations. Its simplicity makes it ideal for quick mental calculations and solving problems using calculators.
Method 2: Using Fraction Multiplication
This method utilizes the fractional representation of a percentage.
Steps:
-
Express the percentage as a fraction: 20% can be written as the fraction 20/100, which simplifies to 1/5.
-
Multiply the fraction by the original number: Multiply 1/5 by 15000. This can be written as (1/5) * 15000.
-
Perform the multiplication: (1/5) * 15000 = 3000.
Therefore, 20% of 15000 is 3000.
This method offers a deeper understanding of the underlying mathematical principles, demonstrating the relationship between percentages, fractions, and whole numbers.
Method 3: Using Proportions
This method uses the concept of ratios and proportions to solve the problem.
Steps:
-
Set up a proportion: We can set up a proportion: 20/100 = x/15000, where 'x' represents 20% of 15000.
-
Cross-multiply: Cross-multiplying gives us 100x = 20 * 15000 = 300000.
-
Solve for x: Divide both sides of the equation by 100 to solve for x: x = 300000 / 100 = 3000.
Therefore, 20% of 15000 is 3000.
This method is particularly useful for understanding the proportional relationships involved and is often employed in more complex percentage problems.
Real-World Applications of Percentage Calculations
Understanding percentage calculations has practical implications across various fields:
Finance and Budgeting:
-
Calculating interest: Interest rates on loans, savings accounts, and investments are expressed as percentages. Calculating interest earned or owed requires understanding percentage calculations. For example, if you have a savings account with a 3% annual interest rate and a balance of $10,000, you can easily determine the yearly interest earned.
-
Tax calculations: Income tax, sales tax, and property tax are usually expressed as percentages. Accurate tax calculation relies heavily on understanding how to compute percentages of different income levels or purchase values.
-
Investment returns: Evaluating investment performance often involves calculating percentage returns on investment to assess profitability.
Sales and Discounts:
-
Calculating discounts: Sales and promotions frequently offer discounts expressed as percentages. Understanding percentage calculations enables consumers to quickly determine the actual price after a discount. For example, a 25% discount on a $50 item is easy to calculate.
-
Markup pricing: Businesses use percentages to determine the selling price of products after adding a markup to the cost price.
Data Analysis and Statistics:
-
Percentage change: Calculating percentage change helps to analyze trends and growth patterns in data. This is crucial in fields like economics, market research, and science. Understanding the percentage increase or decrease in sales, population, or any other quantifiable data is essential for effective decision-making.
-
Frequency distribution: Percentages are used to express the frequency distribution of data. This is vital in statistics for representing data in a concise and easily understandable way.
Everyday Life:
-
Tip calculations: Calculating tips in restaurants is a common application of percentage calculations. Determining a 15% or 20% tip is a straightforward percentage calculation.
-
Calculating proportions in recipes: Scaling up or down recipes often involves adjusting ingredient amounts based on percentage calculations.
Advanced Percentage Calculations
While the examples above focus on basic percentage calculations, more complex scenarios might involve:
-
Calculating percentages of percentages: For instance, determining a 10% discount on an item that is already discounted by 20%. This requires a multi-step calculation involving nested percentages.
-
Calculating the percentage increase or decrease between two numbers: This requires calculating the difference between two numbers and then expressing that difference as a percentage of the original number.
-
Compound interest calculations: Compound interest involves adding interest to the principal amount, and then calculating interest on the new total. This leads to exponential growth and is a more complex application of percentage calculations.
Conclusion
Understanding and applying percentage calculations is a vital skill applicable across numerous domains. This guide has explored multiple methods for calculating percentages, highlighted real-world applications, and touched upon more advanced scenarios. Mastering percentage calculations empowers you to make better financial decisions, analyze data accurately, and confidently navigate many daily numerical challenges. The ability to quickly and accurately calculate percentages provides a significant advantage in various academic, professional, and personal contexts. Regular practice and exposure to different applications will solidify your understanding and improve your proficiency. By consistently using these methods, you’ll become confident and adept at handling percentage calculations with ease.
Latest Posts
Latest Posts
-
What Is 50 As A Percent
May 11, 2025
-
Are Lentils And Legumes The Same
May 11, 2025
-
Is Salt A Mixture Or Pure Substance
May 11, 2025
-
Round 94686 70835 To The Nearest Thousand
May 11, 2025
-
What Is The Relation Between Frequency And Amplitude
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Percent Of 15000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.