What Is 1/2 Of 1 Percent
Juapaving
Mar 29, 2025 · 5 min read
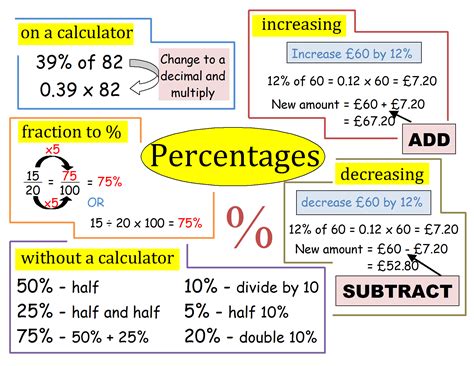
Table of Contents
What is 1/2 of 1 Percent? A Deep Dive into Percentage Calculations
Understanding percentages is a fundamental skill in many aspects of life, from calculating discounts and taxes to analyzing financial data and understanding statistical information. While simple percentages are straightforward, fractional percentages can sometimes seem more complex. This article will thoroughly explore the question: "What is 1/2 of 1 percent?" and delve into the methods and applications of such calculations. We'll cover different approaches to solving this problem, explore the underlying mathematical principles, and offer practical examples to illustrate its relevance.
Understanding Percentages: A Quick Refresher
Before we tackle the specific problem, let's refresh our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. Similarly, 1% means 1 out of 100, or 1/100.
Calculating 1/2 of 1 Percent: Method 1 - Fractional Approach
The most straightforward approach to calculating 1/2 of 1 percent involves working directly with fractions. We can represent 1 percent as 1/100. Therefore, 1/2 of 1 percent is:
(1/2) * (1/100) = 1/200
This means 1/2 of 1 percent is equivalent to one two-hundredth. To express this as a decimal, we perform the division:
1 ÷ 200 = 0.005
Therefore, 1/2 of 1 percent is equal to 0.005 or 0.5%.
Calculating 1/2 of 1 Percent: Method 2 - Decimal Approach
Another method utilizes decimals. We know that 1% is equal to 0.01. To find half of this, we simply divide by 2:
0.01 ÷ 2 = 0.005
This confirms our previous result: 1/2 of 1 percent is 0.005.
Representing 1/2 of 1 Percent: Different Formats
It's crucial to understand that this value can be expressed in several ways, depending on the context and desired level of precision:
- Decimal: 0.005
- Fraction: 1/200
- Percentage: 0.5%
Choosing the appropriate format depends on the specific application. For example, in financial calculations, decimals are often preferred for accuracy. In everyday conversations, percentages are typically more readily understood.
Practical Applications of 1/2 of 1 Percent
While seemingly small, understanding 1/2 of 1 percent has practical applications in various fields:
Finance and Investment:
-
Interest Rates: Interest rates on savings accounts or loans can be expressed in percentages, and understanding fractional percentages is crucial for accurately calculating interest earned or paid. A 0.5% increase in interest might seem insignificant, but over a long period, the cumulative effect can be substantial.
-
Investment Returns: Analyzing investment returns often involves calculating percentage changes. Understanding fractional percentages can help interpret minor fluctuations in portfolio value. A 0.5% increase or decrease in the value of an investment might be a small change, but it can still have a noticeable impact on total profits or losses over time.
-
Marginal Changes: Many financial indicators, like margin, leverage and other metrics, move in small increments. Understanding tiny percentage changes is essential for financial analysis and decision-making.
Statistics and Data Analysis:
-
Error Margins: In statistical analysis, error margins are often expressed as percentages. Understanding fractional percentages allows for a more nuanced interpretation of the accuracy and reliability of data. For instance, a survey might have a margin of error of 0.5%, indicating a small, but still present, level of uncertainty in the results.
-
Growth Rates: Analyzing growth rates in various sectors (e.g., population growth, economic growth) can involve very small percentage changes. Understanding fractional percentages helps in accurately interpreting and comparing these rates.
-
Sampling and Surveys: In statistical analysis, fractional percentages can help to understand variations within a population based on sample size and margin of error.
Science and Engineering:
-
Measurement Precision: In scientific measurements, precision is paramount. Understanding fractional percentages is essential for expressing and interpreting small variations in measurements. For example, a 0.5% error in a scientific experiment might be significant depending on the nature of the experiment.
-
Material Properties: Certain properties of materials, such as concentration of a particular chemical, may change incrementally. A 0.5% change could signify a substantial shift in the overall properties of that material.
-
Calibration and Testing: In calibration and testing procedures, small percentage differences can be very important. A slight deviation of 0.5% might mean the difference between a successful and failed test, or a correctly and incorrectly calibrated instrument.
Beyond the Basics: Working with More Complex Fractional Percentages
The principles applied to calculating 1/2 of 1 percent can be extended to more complex fractional percentages. For example, let's calculate 3/4 of 2 percent:
- Convert percentages to decimals: 2% = 0.02
- Perform the calculation: (3/4) * 0.02 = 0.015
- Convert back to percentage (optional): 0.015 * 100 = 1.5%
Therefore, 3/4 of 2 percent is 0.015 or 1.5%.
This illustrates the versatility of the techniques discussed. The key is to systematically convert percentages to their decimal or fractional equivalents, perform the necessary arithmetic, and then convert back to the desired format if needed.
Conclusion: Mastering Fractional Percentages
Understanding fractional percentages is a valuable skill with broad applications across numerous fields. While seemingly minor, these calculations are vital for accurate analysis and interpretation of data, particularly in quantitative fields. The ability to confidently calculate and interpret fractional percentages demonstrates a strong grasp of mathematical principles and contributes to effective problem-solving in various real-world scenarios. By mastering these techniques, you enhance your analytical capabilities and improve your ability to engage with data-driven decision-making. Remember the core principles: converting percentages to decimals or fractions, performing the calculations, and then converting back to the appropriate format as needed. Practice makes perfect, so work through various examples to solidify your understanding of fractional percentages and their importance.
Latest Posts
Latest Posts
-
Carbon Dioxide And Water Combine To Form
Apr 01, 2025
-
What Is Lcm Of 3 And 8
Apr 01, 2025
-
What Is The Lcm Of 9 12 15
Apr 01, 2025
-
Five Letter Word Ends With Er
Apr 01, 2025
-
What Is The Correct Order Of Photosynthesis
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is 1/2 Of 1 Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
