What Are All The Factors Of 23
Juapaving
Mar 27, 2025 · 5 min read
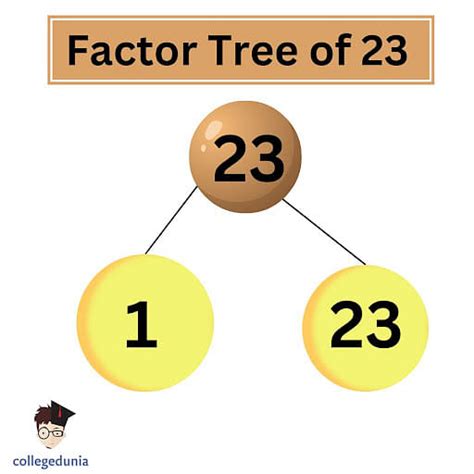
Table of Contents
What Are All the Factors of 23? A Deep Dive into Prime Numbers and Factorization
The seemingly simple question, "What are all the factors of 23?" opens a door to a fascinating world of number theory, prime numbers, and factorization. While the immediate answer might seem obvious, understanding the underlying concepts reveals a deeper appreciation for the structure of mathematics. Let's embark on a journey to explore this seemingly simple number and uncover its rich mathematical properties.
Understanding Factors and Divisibility
Before we delve into the specifics of the number 23, let's establish a firm understanding of fundamental concepts. A factor (or divisor) of a number is a whole number that divides the number exactly, leaving no remainder. In simpler terms, if we divide a number by one of its factors, the result will be another whole number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12 because each of these numbers divides 12 without leaving a remainder.
Divisibility rules provide shortcuts for determining if a number is a factor of another. For example, a number is divisible by 2 if it's even, divisible by 3 if the sum of its digits is divisible by 3, and divisible by 5 if it ends in 0 or 5. While these rules are helpful for smaller numbers, they become less efficient for larger numbers. However, the core principle remains the same: A number is a factor if the division results in a whole number.
Identifying the Factors of 23
Now, let's return to our central question: What are the factors of 23? To find the factors, we need to systematically check each whole number to see if it divides 23 without leaving a remainder.
Let's start with 1: 23 divided by 1 is 23, which is a whole number. Therefore, 1 is a factor of 23.
Next, let's try 2: 23 divided by 2 is 11.5, which is not a whole number. Therefore, 2 is not a factor.
Continuing this process, we find that:
- 23 divided by 3 is approximately 7.67 (not a whole number)
- 23 divided by 4 is approximately 5.75 (not a whole number)
- 23 divided by 5 is 4.6 (not a whole number)
- 23 divided by 6 is approximately 3.83 (not a whole number)
- 23 divided by 7 is approximately 3.29 (not a whole number)
- 23 divided by 8 is approximately 2.88 (not a whole number)
- 23 divided by 9 is approximately 2.56 (not a whole number)
- 23 divided by 10 is 2.3 (not a whole number)
- 23 divided by 11 is approximately 2.09 (not a whole number)
- 23 divided by 12 is approximately 1.92 (not a whole number)
- 23 divided by 22 is approximately 1.05 (not a whole number)
- 23 divided by 23 is 1, which is a whole number. Therefore, 23 is a factor of 23.
This systematic approach reveals that the only whole numbers that divide 23 without leaving a remainder are 1 and 23. Therefore, the factors of 23 are 1 and 23.
The Significance of Prime Numbers
The fact that 23 only has two factors, 1 and itself, signifies a crucial property in number theory: 23 is a prime number.
A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. Prime numbers are the fundamental building blocks of all other whole numbers, as every whole number greater than 1 can be expressed as a unique product of prime numbers (this is known as the Fundamental Theorem of Arithmetic).
Prime numbers are scattered irregularly throughout the number line, and their distribution is a subject of ongoing mathematical research. The search for large prime numbers is also important in cryptography, as the security of many encryption algorithms relies on the difficulty of factoring large numbers into their prime components.
Exploring Related Concepts: Prime Factorization
The concept of prime numbers leads us to the important process of prime factorization. Prime factorization is the process of expressing a composite number (a number that is not prime) as a product of its prime factors. For example, the prime factorization of 12 is 2 x 2 x 3 (or 2² x 3).
Since 23 is a prime number, its prime factorization is simply 23. There's no further breakdown possible because it cannot be factored into smaller prime numbers.
Understanding prime factorization is crucial in various mathematical applications, including:
- Simplifying fractions: Finding the greatest common divisor (GCD) of the numerator and denominator often involves prime factorization.
- Solving algebraic equations: Prime factorization can help in simplifying expressions and finding solutions.
- Cryptography: As mentioned earlier, prime factorization is the basis of many modern encryption techniques.
The Uniqueness of 23: A Closer Look
While 23 might seem like an unremarkable number at first glance, its status as a prime number gives it a special place within the world of mathematics. The seemingly simple act of identifying its factors leads us to a deeper understanding of fundamental concepts and their broader implications.
Here are some additional points to ponder about the number 23:
- Its position in the sequence of prime numbers: 23 is the ninth prime number. The sequence of prime numbers is an infinite sequence, and mathematicians continue to explore the patterns and properties of this fascinating sequence.
- Its relationship to other mathematical concepts: 23 appears in various mathematical contexts, from number theory to geometry and beyond. Its properties contribute to a deeper understanding of underlying mathematical structures.
- Its cultural significance: The number 23 has acquired a certain cultural significance, appearing in various works of fiction and popular culture.
Conclusion: Beyond the Simple Answer
The seemingly simple question of finding the factors of 23 opens a rich tapestry of mathematical concepts. The answer, 1 and 23, highlights the fundamental importance of prime numbers and prime factorization. By exploring this seemingly simple question, we've delved into the heart of number theory, uncovering the intricate beauty and profound significance hidden within the seemingly ordinary world of numbers. The seemingly simple act of finding the factors of 23 has unveiled a wealth of mathematical knowledge, demonstrating how even the most basic questions can lead to deeper insights into the structure and elegance of mathematics. Understanding these concepts enhances our appreciation for the fundamental building blocks of mathematics and their far-reaching applications in various fields.
Latest Posts
Latest Posts
-
What Is The Least Common Multiple Of 5 6 7
Mar 30, 2025
-
How Many Feet In 118 Inches
Mar 30, 2025
-
Rusting Of Iron Is A Chemical Change True Or False
Mar 30, 2025
-
Number Of Atoms In 558 5 Gram Fe
Mar 30, 2025
-
A Piece Of Land That Is Completely Surrounded By Water
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about What Are All The Factors Of 23 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
