The Shapes Of The Horizontal Cross-sections
Juapaving
Mar 28, 2025 · 6 min read
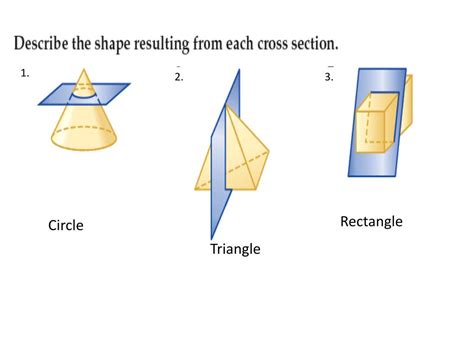
Table of Contents
The Shapes of Horizontal Cross-Sections: A Comprehensive Guide
Understanding the shapes of horizontal cross-sections is crucial in various fields, from engineering and architecture to geology and medicine. A horizontal cross-section, simply put, is the shape you see when you slice through an object parallel to the ground. This seemingly simple concept unlocks a wealth of information about the object's internal structure, volume, and overall form. This article will explore the diverse shapes these cross-sections can take, the factors that influence them, and their practical applications.
Understanding Horizontal Cross-Sections: The Basics
Before delving into the myriad shapes, let's establish a clear understanding of what constitutes a horizontal cross-section. Imagine slicing through a loaf of bread. Each slice represents a horizontal cross-section. The shape of that slice depends entirely on the shape of the loaf. This principle applies to any three-dimensional object.
A horizontal cross-section is a two-dimensional representation of a three-dimensional object, obtained by slicing it parallel to a horizontal plane. This plane is usually considered to be parallel to the Earth's surface. The shape of the cross-section is dictated by the object's geometry and how it varies along its vertical axis.
Key Considerations:
- Orientation: The orientation of the object relative to the horizontal plane significantly impacts the shape of the cross-section. A slightly tilted object will yield different cross-sections compared to one perfectly aligned.
- Uniformity: Objects with uniform shapes along their height (like a cylinder) will produce consistently shaped cross-sections. Non-uniform objects (like a cone) will exhibit varying cross-sections at different heights.
- Complexity: Simple objects produce simple cross-sections, while complex objects, especially those with curves or irregular surfaces, can generate far more intricate shapes.
Common Shapes of Horizontal Cross-Sections
While the possibilities are virtually limitless, several common shapes frequently emerge when examining horizontal cross-sections. Let's explore some of these:
1. Circular Cross-Sections
Circular cross-sections are perhaps the most recognizable and frequently encountered. They are produced when slicing through objects with cylindrical or spherical symmetry.
Examples:
- Cylinders: Pipes, columns, drinking glasses, and many other everyday objects have circular horizontal cross-sections. The cross-section remains consistent regardless of the height at which the slice is taken.
- Spheres: A sphere, when sliced horizontally, reveals a series of concentric circles, with the largest circle being at the sphere's equator.
- Toruses: A torus (donut shape) reveals circular cross-sections, except at its inner and outer edges where the shapes become more complex.
2. Square and Rectangular Cross-Sections
Square and rectangular cross-sections are hallmarks of objects with prismatic shapes. These are common in buildings, structural elements, and many manufactured goods.
Examples:
- Prisms: Rectangular prisms are everywhere – from boxes and bricks to rooms and buildings. The cross-sections are consistently square or rectangular throughout their height.
- Beams: Structural beams in buildings and bridges often possess rectangular cross-sections, providing optimal strength and stability.
- Cuboids: Similar to prisms, a cuboid will consistently yield square or rectangular horizontal cross-sections depending on the orientation of the cut.
3. Elliptical Cross-Sections
Elliptical cross-sections result from slicing through objects with elliptical or slightly irregular cylindrical shapes. They are intermediate between circles and rectangles.
Examples:
- Slightly Tilted Cylinders: If a cylinder is not perfectly upright, its horizontal cross-sections will appear elliptical.
- Ellipsoids: Similar to spheres, ellipsoids (3D ellipses) will yield elliptical cross-sections.
- Certain Biological Structures: Some biological structures, such as certain types of bone or plant stems, might exhibit elliptical horizontal cross-sections.
4. Triangular Cross-Sections
Triangular cross-sections are found in objects with a pyramidal or conical base. The shape varies depending on the height of the slice.
Examples:
- Pyramids: A pyramid, when sliced horizontally, will produce triangular cross-sections, smaller towards the apex and increasing in size towards the base.
- Cones: Similar to pyramids, cones yield triangular cross-sections that diminish in size as you approach the apex.
- Certain Engineered Structures: Some specialized structures in engineering utilize triangular cross-sections for their inherent strength.
5. Irregular Cross-Sections
A significant number of objects possess irregular cross-sections. These shapes defy easy categorization and often require more advanced mathematical techniques to analyze.
Examples:
- Naturally Occurring Objects: Rocks, trees, and many geological formations exhibit irregular and unpredictable horizontal cross-sections.
- Complex Manufactured Goods: Some intricate manufactured goods, like car parts or specialized tools, can have highly irregular cross-sections.
- Biological Structures: Many biological structures, such as organs, display irregular cross-sections due to their complex and often asymmetrical shapes.
Factors Influencing Cross-Sectional Shape
Several factors influence the shapes of horizontal cross-sections:
- Object's Geometry: The inherent shape of the three-dimensional object is the most significant factor.
- Orientation: The angle at which the object is cut relative to the horizontal plane directly affects the resulting shape.
- Internal Structure: Internal cavities, voids, or varying densities within the object can lead to complex cross-sections.
- External Forces: External forces, such as erosion or deformation, can alter the object's shape and consequently its cross-sections.
Applications of Understanding Horizontal Cross-Sections
The knowledge of horizontal cross-sections has vast applications across diverse fields:
1. Engineering and Architecture
- Structural Analysis: Engineers rely heavily on cross-sectional analysis to determine the strength and stability of structures like buildings, bridges, and dams.
- Fluid Dynamics: Understanding cross-sectional area is crucial in fluid dynamics, for example, in designing pipes and channels for optimal flow.
- Material Science: Studying the cross-sections of materials helps determine their internal structure and properties.
2. Geology and Earth Science
- Geological Mapping: Geologists use cross-sectional data to map subsurface formations and understand geological structures.
- Resource Exploration: Analyzing the cross-sections of rock formations helps in locating mineral deposits and other natural resources.
- Environmental Studies: Cross-sections are used in analyzing soil profiles and groundwater flow.
3. Medicine and Biology
- Medical Imaging: Medical imaging techniques like CT scans and MRI produce cross-sectional images of the body, allowing doctors to diagnose diseases and plan treatments.
- Anatomical Studies: Studying the cross-sections of organs and tissues helps researchers understand their structure and function.
- Plant Biology: Analyzing the cross-sections of plants allows botanists to understand their growth and internal structure.
4. Manufacturing and Design
- Product Design: Understanding cross-sectional shapes is essential in designing products for optimal functionality, strength, and aesthetics.
- Manufacturing Processes: Cross-sectional analysis is used in various manufacturing processes to ensure quality control and dimensional accuracy.
Advanced Concepts and Further Exploration
While this article provides a general overview, the topic of horizontal cross-sections extends into far more complex realms. Further exploration might include:
- Calculus and Integral Calculus: Calculating the area and volume of irregular cross-sections often requires calculus-based methods.
- Computer-Aided Design (CAD): CAD software is extensively used to generate and analyze cross-sections of complex three-dimensional objects.
- Finite Element Analysis (FEA): FEA uses cross-sectional data to simulate the behavior of objects under various loads and conditions.
Conclusion
Understanding the shapes of horizontal cross-sections is a fundamental concept with far-reaching implications across many disciplines. From the simple circular cross-section of a pipe to the complex irregular shape of a geological formation, the information contained within a cross-section is invaluable for analysis, design, and problem-solving. This detailed exploration aims to provide a solid foundation for anyone seeking to comprehend and utilize the power of horizontal cross-sectional analysis. Further research into specific applications will unveil the vast potential of this seemingly simple yet profoundly important concept.
Latest Posts
Latest Posts
-
Plants Exchange Gas With The Atmosphere
Mar 31, 2025
-
Numbers In Words 1 To 1000000
Mar 31, 2025
-
Digestion Of Food Is A Physical Or Chemical Change
Mar 31, 2025
-
What Is The Prime Factorization For 63
Mar 31, 2025
-
What Soil Type Holds The Most Water
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about The Shapes Of The Horizontal Cross-sections . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
