Is The Absolute Value Of A Number Always Positive
Juapaving
Mar 26, 2025 · 5 min read
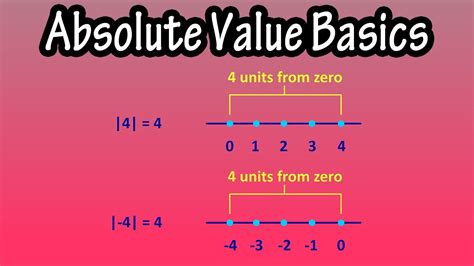
Table of Contents
Is the Absolute Value of a Number Always Positive? A Deep Dive into Absolute Value
The concept of absolute value is fundamental in mathematics, appearing in various areas from basic algebra to advanced calculus. A common question, especially for those new to the subject, is whether the absolute value of a number is always positive. The short answer is yes, with a crucial caveat. This article will delve into the precise definition of absolute value, exploring its properties, applications, and the nuanced exceptions that clarify this seemingly straightforward concept.
Defining Absolute Value: More Than Just a Positive Sign
The absolute value of a number is defined as its distance from zero on the number line. This distance is always non-negative, meaning it's either positive or zero. We often represent the absolute value of a number x using the notation |x|.
Formally, we can define the absolute value function as follows:
- |x| = x if x ≥ 0 (If x is zero or positive, the absolute value is just x itself.)
- |x| = -x if x < 0 (If x is negative, the absolute value is the negation of x, making it positive.)
This two-part definition highlights the crucial aspect: the absolute value function transforms a number into its non-negative counterpart. This transformation is crucial in many mathematical operations and problem-solving scenarios.
Illustrative Examples: Understanding the Mechanics
Let's consider some examples to solidify our understanding:
- |5| = 5: The absolute value of 5 is 5, as 5 is already a positive number.
- |-5| = 5: The absolute value of -5 is also 5. The negative sign is effectively removed, resulting in the distance from zero, which is 5 units.
- |0| = 0: The absolute value of 0 is 0. Zero is neither positive nor negative, but its distance from zero is, naturally, zero.
- | -π | = π: The absolute value of negative pi is simply pi, a positive irrational number.
- | 2/3 | = 2/3: Even with fractions, the absolute value keeps only the magnitude.
These examples demonstrate that the absolute value function strips away the sign, leaving only the magnitude or size of the number. This is its core functionality.
Properties of the Absolute Value Function: A Mathematical Toolkit
The absolute value function exhibits several important properties that make it a powerful tool in various mathematical contexts. Understanding these properties is vital for effective problem-solving.
1. Non-negativity: The Foundational Property
As previously stated, the most fundamental property is non-negativity: |x| ≥ 0 for all x. This is the direct consequence of the definition—the distance from zero cannot be negative.
2. Even Function Property: Symmetry Around the Y-axis
The absolute value function is an even function. This means it exhibits symmetry around the y-axis. Mathematically, this translates to: |-x| = |x. The absolute value of a negative number is the same as the absolute value of its positive counterpart.
3. Multiplicative Property: Absolute Values of Products
The absolute value of a product is equal to the product of the absolute values: |xy| = |x||y|. This property extends to products of multiple numbers.
4. Triangle Inequality: A Powerful Bound
The absolute value function satisfies the triangle inequality: |x + y| ≤ |x| + |y|. This inequality states that the absolute value of the sum of two numbers is less than or equal to the sum of their absolute values. This property is crucial in various areas of mathematics, including analysis and linear algebra.
5. Absolute Value and Inequalities: Solving Equations
Absolute value inequalities are frequently encountered in algebra. Solving these requires careful consideration of both positive and negative cases. For instance, solving |x| < 5 means finding the values of x such that -5 < x < 5.
Applications of Absolute Value: Beyond the Textbook
The absolute value function isn't just a theoretical concept; it finds extensive applications in various fields:
1. Geometry and Distance: Measuring Separation
In geometry, the absolute value naturally represents distance. The distance between two points on the number line is given by the absolute value of the difference between their coordinates. For example, the distance between 3 and -2 is |3 - (-2)| = |5| = 5.
2. Physics and Engineering: Magnitude Without Direction
In physics and engineering, absolute value is used to represent the magnitude of a quantity, disregarding its direction. For example, speed is the absolute value of velocity. Velocity includes both speed and direction (e.g., 20 m/s east), whereas speed is only the magnitude (20 m/s).
3. Computer Science and Programming: Error Handling and Comparisons
In computer programming, absolute value functions are frequently used in error handling and comparisons. For example, checking if two floating-point numbers are "close enough" often involves comparing the absolute value of their difference to a small tolerance.
4. Statistics and Data Analysis: Measuring Deviation
In statistics, absolute value is used in calculating measures of dispersion such as the mean absolute deviation. This provides a measure of the average distance of data points from the mean.
5. Real-World Scenarios: Modeling Variations
Real-world applications often involve modeling variations or deviations from a standard or expected value. Absolute values are essential here, as the direction of deviation is often less important than its magnitude. For example, a manufacturing process might specify an acceptable tolerance range around a target measurement, expressed using absolute values.
Addressing the "Exception": Zero
While the absolute value of any non-zero number is always positive, the absolute value of zero is zero. This is not a contradiction but a specific case consistent with the definition. The distance from zero to zero is, of course, zero. So, while the absolute value is always non-negative, it's only strictly positive for non-zero numbers.
Conclusion: A Comprehensive Understanding
The absolute value of a number is always non-negative. While it's often positive, the exception of zero reinforces the idea that absolute value measures distance from zero. Understanding its properties and applications is vital for anyone working with mathematical concepts, whether in pure mathematics, applied sciences, or computer programming. Mastering this seemingly simple concept unlocks the door to a deeper understanding of numerous mathematical tools and their practical implications. The consistent use of absolute values in various fields underscores its importance and its enduring relevance in various disciplines. Its ability to express magnitude without direction makes it a ubiquitous tool in mathematical modelling and problem-solving. The absolute value of a number, therefore, is a fundamental concept with wide-ranging and significant implications.
Latest Posts
Latest Posts
-
How Much Valence Electrons Does Oxygen Have
Mar 29, 2025
-
What Percent Of 80 Is 12
Mar 29, 2025
-
Least Common Multiple Of 6 7 And 8
Mar 29, 2025
-
What Is 7 8 Expressed As A Percent
Mar 29, 2025
-
How To Write 200 In Words
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Is The Absolute Value Of A Number Always Positive . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
