Is 6 A Factor Of 12
Juapaving
Mar 30, 2025 · 5 min read
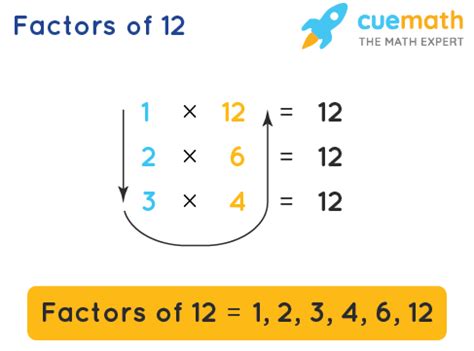
Table of Contents
Is 6 a Factor of 12? A Deep Dive into Divisibility and Factors
The question, "Is 6 a factor of 12?" might seem simple at first glance. For many, the answer is immediately apparent. But let's delve deeper than a simple "yes" or "no." This article will explore the concept of factors, divisibility rules, and related mathematical concepts, using the example of 6 and 12 to illustrate these ideas thoroughly. We'll uncover why 6 is indeed a factor of 12 and explore broader implications within the realm of number theory.
Understanding Factors and Divisibility
Before directly addressing whether 6 is a factor of 12, let's establish a clear understanding of the fundamental terms involved.
Factors: Factors of a number are whole numbers that divide into that number without leaving a remainder. In simpler terms, they are numbers that can be multiplied by other whole numbers to produce the original number. For instance, the factors of 12 are the numbers that can be multiplied to get 12.
Divisibility: Divisibility refers to the property of a number being perfectly divisible by another number, resulting in a zero remainder. We say that a number a is divisible by a number b if the division of a by b leaves no remainder. This is often expressed as a ≡ 0 (mod b).
Methods to Determine if 6 is a Factor of 12
There are several ways to determine if 6 is a factor of 12. Let's examine the most common approaches:
1. Division
The most straightforward method is to perform the division: 12 ÷ 6 = 2. Since the division results in a whole number (2) with no remainder, we can definitively conclude that 6 is a factor of 12.
2. Prime Factorization
Prime factorization involves breaking down a number into its prime factors – prime numbers that multiply together to produce the original number. Let's find the prime factorization of both 6 and 12:
- 6: 2 x 3
- 12: 2 x 2 x 3 (or 2² x 3)
Notice that all the prime factors of 6 (2 and 3) are also present in the prime factorization of 12. This is a key indicator that 6 is a factor of 12. If the prime factorization of a smaller number is entirely contained within the prime factorization of a larger number, then the smaller number is a factor of the larger number.
3. Listing Factors
We can list all the factors of 12: 1, 2, 3, 4, 6, and 12. Since 6 is present in this list, it confirms that 6 is indeed a factor of 12. This method is particularly useful for smaller numbers but becomes less efficient as numbers grow larger.
4. Divisibility Rules
While not directly applicable to the number 6 itself as a divisibility rule, understanding divisibility rules for its component factors (2 and 3) can indirectly help. A number is divisible by 6 if it's divisible by both 2 and 3. Since 12 is divisible by both 2 (12 ÷ 2 = 6) and 3 (12 ÷ 3 = 4), it follows that 12 is divisible by 6.
Expanding the Concept: Factors and Multiples
Understanding factors is intimately linked to understanding multiples.
Multiples: Multiples of a number are the products of that number and any whole number. For example, multiples of 6 are 6, 12, 18, 24, and so on.
Notice the relationship: 6 is a factor of 12, and 12 is a multiple of 6. This reciprocal relationship is a fundamental concept in number theory. If a is a factor of b, then b is a multiple of a.
Applications of Factors and Divisibility
The concept of factors and divisibility has far-reaching applications in various areas of mathematics and beyond:
- Algebra: Factoring algebraic expressions relies heavily on understanding factors. For example, factoring x² + 5x + 6 involves finding factors of 6 that add up to 5.
- Fraction Simplification: Finding the greatest common factor (GCF) of the numerator and denominator of a fraction is crucial for simplifying fractions to their lowest terms. The GCF is the largest factor common to both numbers.
- Geometry: Divisibility plays a role in geometric problems, such as determining if a shape can be divided into equal parts.
- Computer Science: Concepts like modular arithmetic (which deals with remainders) are fundamental in cryptography and other areas of computer science.
- Real-World Applications: Divisibility is essential in everyday scenarios, such as dividing items equally among people, calculating proportions, or scheduling tasks.
Beyond 6 and 12: Exploring Other Numbers
Let's extend our understanding by considering similar scenarios:
Is 4 a factor of 12? Yes, because 12 ÷ 4 = 3.
Is 8 a factor of 12? No, because 12 ÷ 8 leaves a remainder of 4.
Is 6 a factor of 18? Yes, because 18 ÷ 6 = 3.
Is 6 a factor of 20? No, because 20 ÷ 6 leaves a remainder of 2.
These examples highlight the importance of performing the division or examining prime factorizations to determine if one number is a factor of another.
Conclusion: The Definitive Answer
Returning to our original question: Yes, 6 is a factor of 12. We've explored multiple methods to confirm this, ranging from simple division to the analysis of prime factorizations. This seemingly straightforward question provided an excellent opportunity to delve into the rich and fundamental concepts of factors, divisibility, multiples, and their broader applications within mathematics and beyond. Understanding these concepts is crucial for developing a solid foundation in mathematical reasoning and problem-solving skills. The relationship between 6 and 12 serves as a foundational example for understanding these crucial mathematical building blocks. The exploration of this seemingly simple query opens doors to a deeper understanding of more complex mathematical relationships.
Latest Posts
Latest Posts
-
What Are The Common Factors Of 56
Apr 01, 2025
-
Which Noble Gas Has The Highest First Ionization Energy
Apr 01, 2025
-
1000 Meters Is Equal To How Many Kilometers
Apr 01, 2025
-
The Type Of Life Cycle Seen In Plants Is Called
Apr 01, 2025
-
Decimal Greater Than Less Than Calculator
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Is 6 A Factor Of 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
