Is 12 A Multiple Of 4
Juapaving
Apr 12, 2025 · 5 min read
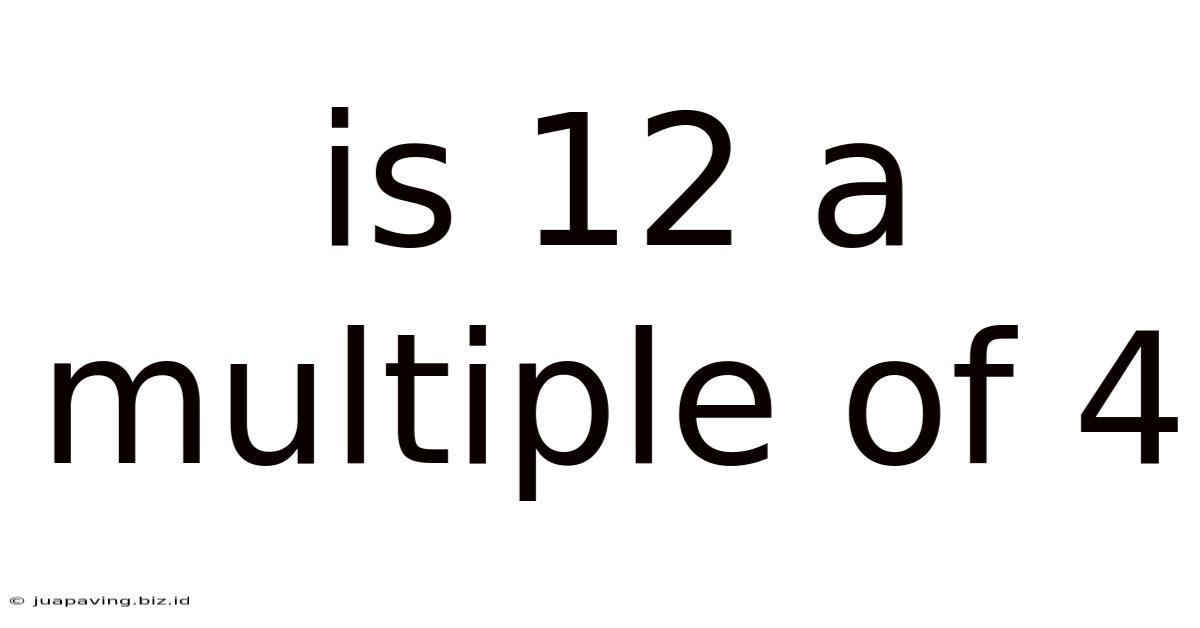
Table of Contents
Is 12 a Multiple of 4? A Deep Dive into Divisibility and Factors
The question, "Is 12 a multiple of 4?" might seem simple at first glance. For many, the answer is immediately apparent. However, exploring this seemingly straightforward question allows us to delve into fundamental mathematical concepts like divisibility, multiples, factors, and even touch upon more advanced ideas like modular arithmetic. This comprehensive guide will not only answer the question definitively but also provide a robust understanding of the underlying principles.
Understanding Multiples and Divisibility
Before tackling the specific question, let's solidify our understanding of key terms.
Multiple: A multiple of a number is the product of that number and any integer (whole number). For example, multiples of 4 are 4 (4 x 1), 8 (4 x 2), 12 (4 x 3), 16 (4 x 4), and so on. Essentially, a multiple is the result of repeated addition of a number.
Divisibility: Divisibility refers to whether a number can be divided by another number without leaving a remainder. If a number is divisible by another, it means the second number is a factor of the first. For instance, 12 is divisible by 4 because 12 ÷ 4 = 3 with no remainder.
Factor: A factor of a number is a whole number that divides the number exactly without leaving a remainder. The factors of 12 are 1, 2, 3, 4, 6, and 12. These are the numbers that can be multiplied together to produce 12.
Answering the Question: Is 12 a Multiple of 4?
Now, let's return to our central question: Is 12 a multiple of 4? The answer is a resounding yes.
Here's why:
-
Multiplication: 12 can be obtained by multiplying 4 by an integer: 4 x 3 = 12. Since 3 is an integer, 12 is a multiple of 4.
-
Division: 12 can be divided by 4 without leaving a remainder: 12 ÷ 4 = 3. This demonstrates the divisibility of 12 by 4.
-
Factorization: 4 is a factor of 12. This means 4 divides 12 evenly.
Exploring Further: Related Concepts and Applications
The simple question about the multiplicity of 12 and 4 opens doors to several related mathematical concepts:
1. Prime Factorization
Understanding prime factorization can help us further analyze the relationship between 12 and 4. Prime factorization involves expressing a number as a product of its prime factors (numbers divisible only by 1 and themselves).
- The prime factorization of 12 is 2 x 2 x 3 (or 2² x 3).
- The prime factorization of 4 is 2 x 2 (or 2²).
Notice that the prime factors of 4 (2 x 2) are also contained within the prime factorization of 12. This is another way to see that 4 is a factor of 12, and therefore, 12 is a multiple of 4.
2. Greatest Common Divisor (GCD) and Least Common Multiple (LCM)
The concepts of GCD and LCM are crucial in number theory and have practical applications in various fields.
-
GCD: The greatest common divisor (GCD) of two or more numbers is the largest number that divides all of them without leaving a remainder. The GCD of 12 and 4 is 4.
-
LCM: The least common multiple (LCM) of two or more numbers is the smallest number that is a multiple of all of them. The LCM of 12 and 4 is 12.
3. Modular Arithmetic
Modular arithmetic, often denoted as "mod," is a system of arithmetic for integers where numbers "wrap around" upon reaching a certain value, called the modulus. In the context of our question, we can express the relationship as:
12 ≡ 0 (mod 4)
This reads as "12 is congruent to 0 modulo 4." It signifies that when 12 is divided by 4, the remainder is 0. This is another way to confirm that 12 is a multiple of 4.
4. Real-World Applications
The understanding of multiples and divisibility isn't just confined to theoretical mathematics. It has practical applications in various areas:
-
Measurement and Units: Converting units of measurement often involves understanding multiples. For example, converting inches to feet (12 inches = 1 foot) relies on the fact that 12 is a multiple of 12.
-
Scheduling and Time Management: Scheduling tasks or events that repeat at regular intervals involves using multiples. For example, if an event happens every 4 days, determining when it occurs again uses the concept of multiples.
-
Data Structures and Algorithms: In computer science, the concepts of multiples and divisibility are crucial for optimizing algorithms and managing data structures efficiently.
Expanding the Concept: Exploring Other Multiples
Let's extend our understanding by exploring other multiples of 4:
- Multiples less than 12: 4, 8
- Multiples between 12 and 20: 16
- Multiples between 20 and 30: 24, 28
- Multiples greater than 30: 32, 36, 40... and so on.
The sequence of multiples of 4 is infinite, extending indefinitely in both positive and negative directions.
Conclusion: A Simple Question with Profound Implications
The seemingly simple question, "Is 12 a multiple of 4?" serves as a springboard to explore a rich tapestry of mathematical concepts. By understanding multiples, divisibility, factors, and related ideas, we gain a deeper appreciation for the fundamental building blocks of number theory and their applications in various fields. The affirmative answer – yes, 12 is indeed a multiple of 4 – is only the starting point for a broader exploration of mathematical relationships. This exploration not only enhances our mathematical literacy but also equips us with problem-solving skills applicable across diverse disciplines. The interconnectedness of these concepts highlights the beauty and elegance of mathematics. The seemingly simple question about 12 and 4 underscores the power of fundamental mathematical principles to unlock deeper understanding and applications across various fields.
Latest Posts
Latest Posts
-
Words That Starts With A S
May 09, 2025
-
Which Is More 25 Or 5
May 09, 2025
-
As Altitude Increases The Air Pressure
May 09, 2025
-
7 16 As A Fraction In Simplest Form
May 09, 2025
-
How Many Hours Is 1600 Minutes
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 12 A Multiple Of 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.