Is 1 3 Greater Than 2 6
Juapaving
May 12, 2025 · 5 min read
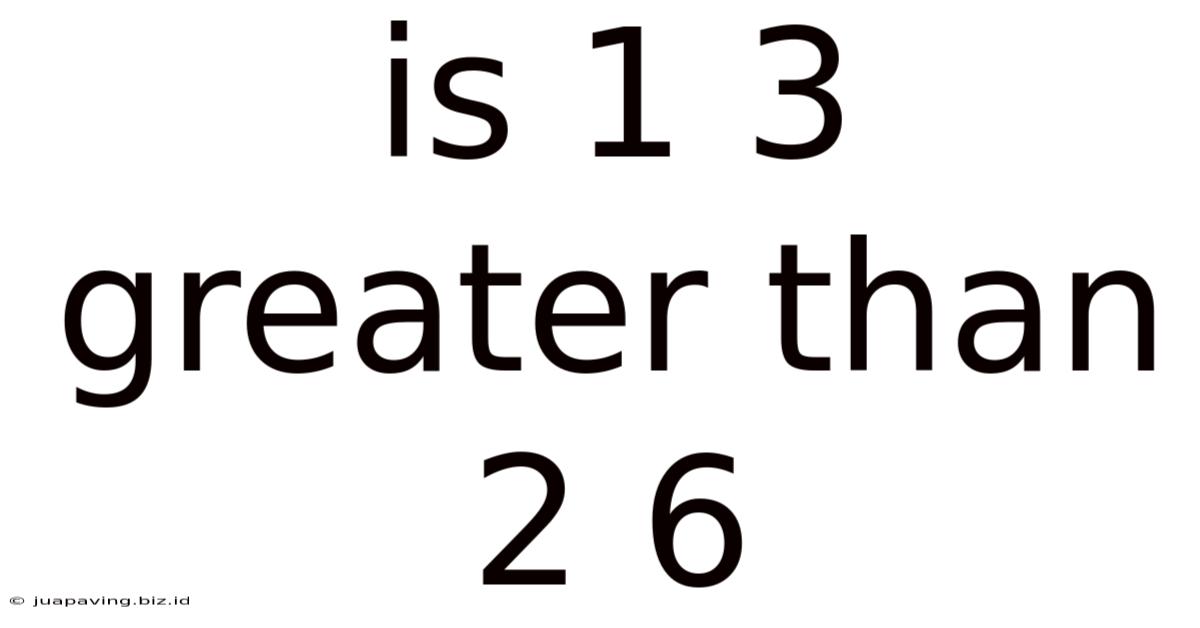
Table of Contents
Is 1/3 Greater Than 2/6? A Deep Dive into Fraction Comparison
The question, "Is 1/3 greater than 2/6?" might seem trivial at first glance. For those well-versed in fractions, the answer is immediately apparent. However, understanding the underlying principles of fraction comparison is crucial for building a strong foundation in mathematics. This article will not only answer the question definitively but also explore various methods for comparing fractions, delving into the conceptual understanding behind the calculations. We’ll also touch upon the practical applications of this seemingly simple concept.
Understanding Fractions: A Quick Refresher
Before we tackle the main question, let's ensure a solid understanding of fractions. A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, while the denominator shows how many equal parts the whole is divided into. For example, in the fraction 1/3, the numerator is 1 and the denominator is 3, indicating one part out of three equal parts.
Method 1: Finding a Common Denominator
The most common and reliable method for comparing fractions is to find a common denominator. This involves converting the fractions so they share the same denominator. Once they share a denominator, comparing the numerators directly will reveal which fraction is larger.
Let's apply this method to our question: Is 1/3 greater than 2/6?
-
Step 1: Identify the denominators: The denominators are 3 and 6.
-
Step 2: Find the least common multiple (LCM): The LCM of 3 and 6 is 6. This means we'll convert both fractions to have a denominator of 6.
-
Step 3: Convert the fractions:
-
1/3 remains unchanged if we multiply both numerator and denominator by 2: (12)/(32) = 2/6
-
2/6 stays as it is.
-
-
Step 4: Compare the numerators: Now we have 2/6 and 2/6. Since the numerators are equal, the fractions are equivalent.
Therefore, 1/3 is NOT greater than 2/6; they are equal.
Method 2: Simplifying Fractions
Another effective approach involves simplifying fractions to their lowest terms. This means reducing the numerator and denominator by dividing both by their greatest common divisor (GCD). Simplifying fractions often makes comparison easier.
Let's apply this method:
-
Step 1: Simplify the fractions:
-
1/3 is already in its simplest form.
-
2/6 can be simplified by dividing both the numerator and the denominator by their GCD, which is 2: (2/2)/(6/2) = 1/3
-
-
Step 2: Compare the simplified fractions: Now we have 1/3 and 1/3. Again, they are equal.
Method 3: Visual Representation
Visual aids, such as diagrams or pie charts, can be incredibly helpful, especially for beginners. Let's visualize the fractions:
Imagine a circle divided into three equal parts. Shading one part represents 1/3.
Now imagine another circle divided into six equal parts. Shading two parts represents 2/6.
Visually, it becomes clear that both representations cover the same area, confirming that 1/3 and 2/6 are equivalent.
Practical Applications: Why is Understanding Fraction Comparison Important?
The ability to compare fractions isn't just an academic exercise; it has numerous real-world applications:
-
Cooking and Baking: Recipes often require precise measurements. Understanding fractions is essential to accurately measure ingredients and adjust recipes. For example, knowing whether 1/2 cup is more or less than 3/8 cup is crucial for a successful outcome.
-
Construction and Engineering: Precise measurements are paramount in construction and engineering. Fractions are used extensively in blueprints and calculations to ensure accuracy and structural integrity.
-
Finance: Understanding fractions is fundamental in finance for calculations involving percentages, interest rates, and stock prices.
-
Data Analysis: In data analysis, fractions are frequently encountered when working with proportions and probabilities. The ability to accurately compare fractions allows for effective interpretation of data.
-
Everyday Life: Dividing resources fairly, sharing items, and understanding sales discounts all require an understanding of fractions.
Beyond the Basics: Comparing More Complex Fractions
The methods discussed above work well for simple fractions. However, when dealing with more complex fractions (e.g., fractions with larger numbers, mixed numbers, or improper fractions), a more systematic approach is necessary.
Mixed Numbers: Mixed numbers are numbers that combine a whole number and a fraction (e.g., 1 1/2). To compare mixed numbers, you should first convert them into improper fractions (fractions where the numerator is larger than the denominator). Then, apply the common denominator method or simplify the fractions before comparing them.
Improper Fractions: Improper fractions are fractions where the numerator is greater than or equal to the denominator (e.g., 5/4). These are often converted into mixed numbers for easier comparison and interpretation.
Advanced Techniques: Cross-Multiplication
For comparing two fractions directly without finding a common denominator, cross-multiplication can be used.
To compare fractions a/b and c/d, we cross-multiply:
-
Multiply the numerator of the first fraction by the denominator of the second fraction (a * d).
-
Multiply the numerator of the second fraction by the denominator of the first fraction (c * b).
-
If (a * d) > (c * b), then a/b > c/d.
-
If (a * d) < (c * b), then a/b < c/d.
-
If (a * d) = (c * b), then a/b = c/d.
Let’s apply cross-multiplication to 1/3 and 2/6:
1 * 6 = 6 2 * 3 = 6
Since 6 = 6, 1/3 = 2/6.
Conclusion: Mastering Fraction Comparison
The simple question, "Is 1/3 greater than 2/6?" serves as a gateway to understanding the broader concept of fraction comparison. This fundamental skill is essential across numerous fields and everyday situations. By mastering various methods – finding a common denominator, simplifying fractions, using visual representations, and cross-multiplication – you build a solid mathematical foundation and equip yourself with tools for tackling more complex fraction-related problems. Remember to choose the method that best suits the complexity of the fractions you are comparing, and always strive for a deep understanding of the underlying principles. The ability to confidently and accurately compare fractions is a valuable skill that will benefit you throughout your life.
Latest Posts
Latest Posts
-
Base 10 To Base 5 Converter
May 12, 2025
-
Your Image In A Plane Mirror Is
May 12, 2025
-
What Percentage Is 2 Of 6
May 12, 2025
-
Is Mixing Sugar In Water A Chemical Change
May 12, 2025
-
Ammeter Is Connected In Series Or Parallel
May 12, 2025
Related Post
Thank you for visiting our website which covers about Is 1 3 Greater Than 2 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.