Integral Of X 2e X 2
Juapaving
May 11, 2025 · 5 min read
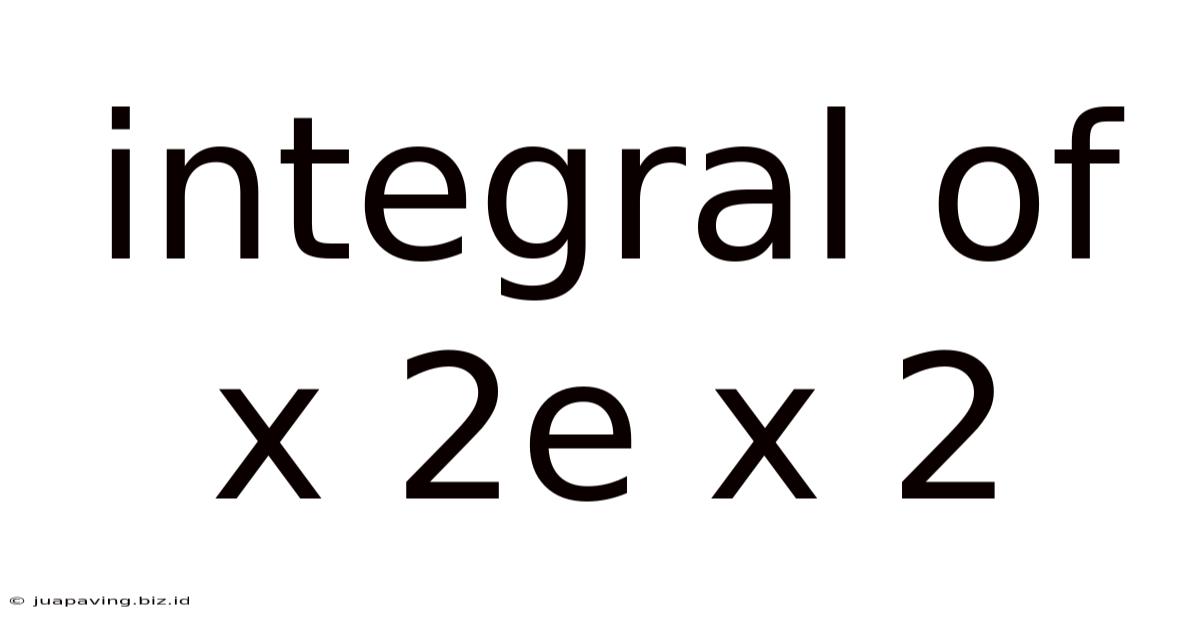
Table of Contents
Demystifying the Integral of x²e^(x²)
The integral ∫x²e^(x²)dx is a fascinating beast in the world of calculus. Unlike many integrals that yield to straightforward techniques like substitution or integration by parts, this one presents a significant challenge. There's no elementary antiderivative; it cannot be expressed using a finite combination of elementary functions (polynomials, exponentials, logarithms, trigonometric functions, and their inverses). This doesn't mean it's unsolvable; rather, it requires a deeper understanding of advanced mathematical techniques and often leads to the use of special functions. This article will delve into the complexities of this integral, exploring different approaches and highlighting the reasons behind its intractability.
Why is ∫x²e^(x²)dx So Difficult?
The difficulty arises from the interplay between the polynomial term (x²) and the exponential term (e^(x²)). Let's examine the standard integration techniques and see why they fail:
1. Substitution:
Substitution is often the first tool we reach for. However, trying to substitute u = x² leads to du = 2x dx, leaving us with an extra 'x' that we can't easily handle within the integral. Similarly, substituting u = e^(x²) doesn't simplify the expression in a manageable way.
2. Integration by Parts:
Integration by parts, based on the formula ∫udv = uv - ∫vdu, seems promising at first. However, repeated applications of this method only lead to more complex integrals, creating a cyclical pattern that doesn't lead to a solution using elementary functions. Let's illustrate this:
If we let u = x² and dv = e^(x²)dx, then du = 2x dx and v would require the very integral we're trying to solve! This creates a recursive situation, preventing us from reaching a closed-form solution.
Exploring Approaches and Special Functions
Since elementary methods fail, we must explore alternative avenues. These often involve advanced mathematical concepts and the introduction of special functions that are not part of the standard calculus curriculum.
1. The Error Function (erf(x)) and its Relatives
The error function, erf(x), is defined as:
erf(x) = (2/√π) ∫₀ˣ e^(-t²) dt
This function is closely related to the Gaussian distribution and frequently appears in probability and statistics. While it doesn't directly solve our integral, it highlights the connection between integrals involving e^(-x²) and special functions. Notice the key difference: our integral involves e^(x²), not e^(-x²). The negative sign significantly alters the behavior and solvability of the integral.
2. Power Series Expansion
One approach is to express e^(x²) as its Maclaurin series:
e^(x²) = 1 + x² + (x⁴)/2! + (x⁶)/3! + ...
Substituting this into our integral, we get:
∫x²e^(x²)dx = ∫x²(1 + x² + (x⁴)/2! + (x⁶)/3! + ...) dx
This can be integrated term by term:
∫x²(1 + x² + (x⁴)/2! + (x⁶)/3! + ...) dx = x³/3 + x⁵/5 + (x⁷)/(7*2!) + (x⁹)/(9*3!) + ... + C
This provides an infinite series representation of the integral. While this series converges for all x, it doesn't provide a closed-form, elementary function solution. It's a useful approximation, especially for small values of x, but the series representation quickly becomes unwieldy for larger values or higher degrees of accuracy.
3. Numerical Integration Techniques
For practical applications where an exact solution is not crucial, numerical integration techniques such as the trapezoidal rule, Simpson's rule, or Gaussian quadrature can be employed to approximate the definite integral over a specific interval. These methods offer a way to obtain numerical solutions with varying degrees of accuracy. However, this approach yields numerical results for specific integration limits and not a general antiderivative.
Why the Lack of an Elementary Solution Matters
The absence of an elementary solution for ∫x²e^(x²)dx underscores an important point: not all integrals have elegant, closed-form solutions using standard mathematical functions. This situation highlights the limitations of elementary calculus and points towards the need for more advanced mathematical tools and approaches. Understanding this limitation is crucial for both theoretical and practical applications.
Practical Applications and Context
Despite its lack of an elementary solution, integrals similar to ∫x²e^(x²)dx arise in various fields, including:
-
Probability and Statistics: Integrals related to Gaussian distributions frequently appear in statistical calculations and probability density function evaluations. While not directly identical, understanding the related integrals involving e^(-x²) provides valuable insight.
-
Physics and Engineering: The Gaussian function, closely tied to the error function, is essential in representing various physical phenomena, such as the distribution of molecular velocities in a gas (Maxwell-Boltzmann distribution) and in solving the heat equation.
-
Quantum Mechanics: Gaussian functions are used extensively in quantum mechanical calculations and wave function approximations.
Conclusion: Embracing the Complexity
The integral ∫x²e^(x²)dx serves as a reminder that the mathematical landscape extends beyond the realm of elementary functions. While we cannot express its antiderivative using a finite combination of standard functions, we can explore alternative methods such as power series expansion or numerical techniques to obtain approximations or solutions for specific applications. Understanding the limitations and exploring the advanced mathematical concepts required to address such challenges is an important step in mastering calculus and appreciating the richness and complexity of mathematical analysis. The journey of tackling this seemingly simple integral leads to a deeper understanding of special functions, numerical analysis, and the broader landscape of mathematical techniques. This underscores that the "unsolvability" in elementary terms shouldn't be viewed as a failure but rather an invitation to explore more advanced and sophisticated tools in mathematical analysis.
Latest Posts
Latest Posts
-
Proportions In Triangles Quiz Part 1
May 12, 2025
-
Direction Of Impulse In A Neuron
May 12, 2025
-
How Many Valence Electrons Do Calcium Have
May 12, 2025
-
What Is The Surface Area Of The Right Cylinder Below
May 12, 2025
-
Is 1 6 Bigger Than 1 4
May 12, 2025
Related Post
Thank you for visiting our website which covers about Integral Of X 2e X 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.